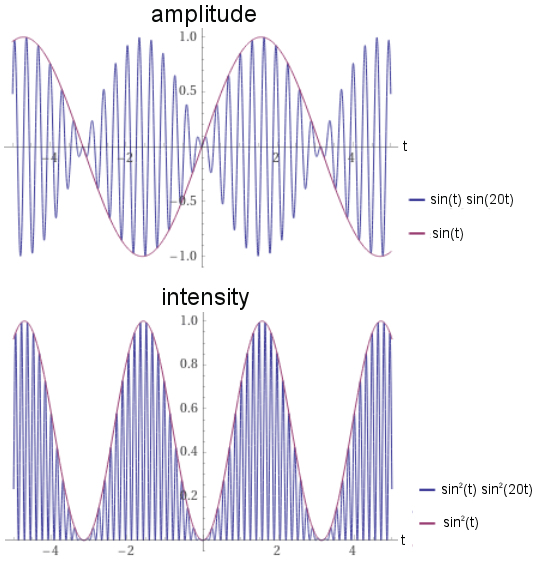
Perhaps if one thinks about the intensity (energy per unit area per second) of a wave rather than the amplitude of the wave the doubling of frequency becomes clearer?

You will note that the $\sin^2(t)$ envelope modulates the $\sin^2(20t)$ graph and that modulation envelope has twice the frequency of the $\sin(t)$ graph.

Or put another way, the intensity modulating envelope $\sin^2(t) = \frac12(1-\cos(2t)$, which is made up of a $\cos(2t)$ function displaced by $+1$ which has twice the frequency of a $\sin(t)$ function

The equation $x=A\sin\left(\dfrac{(ω_1-ω_2)t}{2}\right)\times \cos\left(\dfrac{(ω_1+ω_2)t}{2}\right)$ can be thought of as consisting of two parts which are multiplied together.

The $\cos\left(\dfrac{(ω_1+ω_2)t}{2}\right)$ term which is an oscillation at a frequency which is the average of $\omega_1$ and $\omega_2$.

The $A\sin\left(\dfrac{(ω_1-ω_2)t}{2}\right)$ term which oscillates at a lower frequency, $\dfrac{(ω_1-ω_2)}{2}$, than the other term and has an amplitude $A$.

Adding some numbers, let $A=1,\,\omega_1 = 2\,\pi\,100.5$ and $\omega_2 = 2\,\pi\,99.5$, which is a difference of $1\,\rm Hz$.

Thus $\dfrac{(ω_1+ω_2)}{2} = 2\,\pi\, 100$ and $\dfrac{(ω_1-ω_2)}{2} = 2\,\pi\, \frac 12$ ie oscillations at $100\,\rm Hz$ are changing in amplitude at a frequency of $\frac 12\,\rm Hz$ as shown in the graph below.

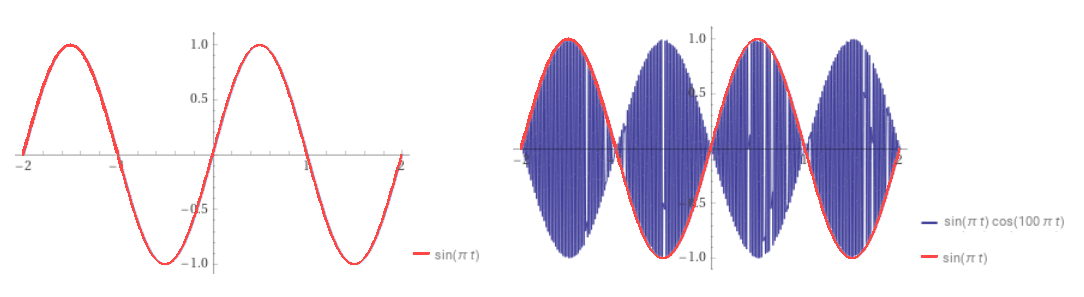
The variation in amplitude of the wave of frequency $100\,\rm Hz$ is shown in the right hand diagram.
The $100\,\rm Hz$ wave cannot be properly drawn because between $t=0\,\rm s$ and $t=1\,\rm s$ one hundred oscillations would have to be displayed.

What you will note is that there is zero amplitude of the $100\,\rm Hz$ wave at $t=0\,\rm s$, maximum amplitude at $t=\frac 12\,\rm s$, zero amplitude at at $t=1\,\rm s$, maximum amplitude at $t=\frac 32\,\rm s$, zero amplitude at at $t=2\,\rm s$.
Thus in $2$ seconds (the period of the $\sin \left(2\,\pi\,\frac12\right)$ term, the amplitude of the $100\rm \, Hz$ goes through two oscillations ie the beat frequency is $\dfrac{(ω_1-ω_2)}{2} \times 2 = ω_1-ω_2$ which in the case is $100.5-99.5=1\,\rm Hz$.

Perhaps what you actually hear will become clearer after you use this rather nice simulation, Interference in Time and Beats Simulation?

When first started the simulation sets $f_{\rm A}=300\,\rm Hz$ and $f_{\rm B}=305\,\rm Hz$.

On running the simulation you will hear a sound wave of frequency $302.5\rm\,Hz$ $pulsating$ at a frequency of $5\rm\,Hz$ which is the beat frequency.

Keeping frequency $f_{\rm A}$ the same change $f_{\rm B}$ to:

$\bf 1$ $301\,\rm Hz$ to hear a $1\rm\,Hz$ beat,

$\bf 2$ $300.1\,\rm Hz$ to hear a $0.1\rm\,Hz$, period $10\,\rm s$, beat,

$\bf 3$ $300.05\,\rm Hz$ to hear a $0.05\rm\,Hz$, period $20\,\rm s$, beat. [Do not worry that the hundredth of a hertz are not displayed as the simulation will still work.]

$\bf 4$ Have fun noting that the lowest available frequency is $40\,\rm Hz$ and the highest $1000\,\rm Hz$ so it is not possible with this simulation to show two ultrasonic frequencies producing an audible beat frequency eg $40,000\,\rm Hz$ and $40,100\,\rm Hz$ producing an audible $100\,\rm Hz$ tone