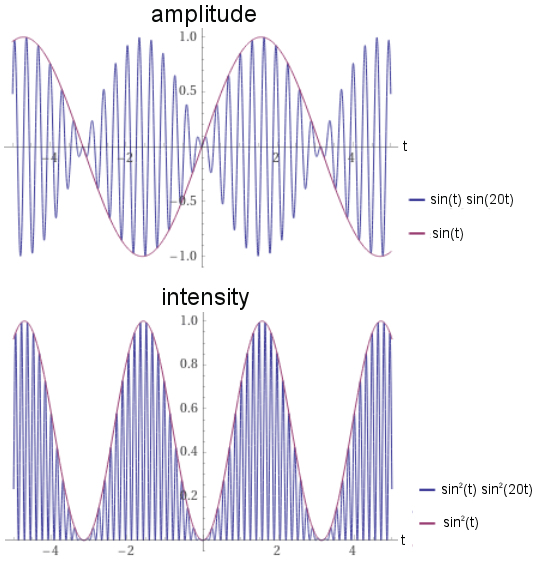
Perhaps if one thinks about the intensity (energy per unit area per second) of a wave rather than the amplitude of the wave the doubling of frequency becomes clearer?
You will note that the $\sin^2(t)$ envelope modulates the $\sin^2(20t)$ graph and that modulation envelope has twice the frequency of the $\sin(t)$ graph.
Or put another way, the intensity modulating envelope $\sin^2(t) = \frac12(1-\cos(2t)$, which is made up of a $\cos(2t)$ function displaced by $+1$ which has twice the frequency of a $\sin(t)$ function