This question was previously asked 11 years ago here, but I believe it does not answer my question for the following reasons:
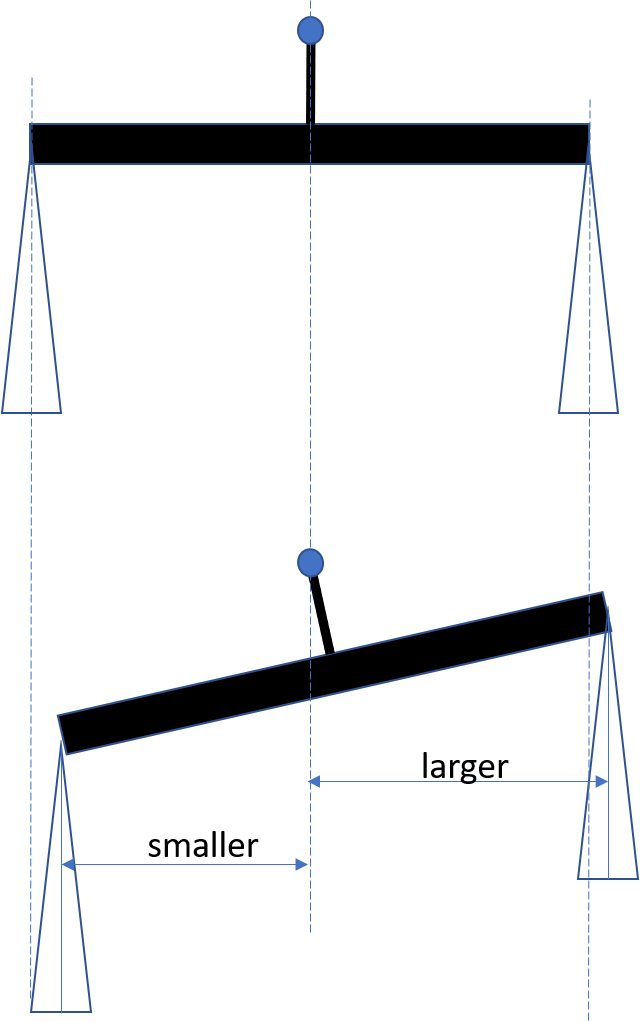
(A) The first answer takes a different system, mine looks like this.
(B) The second answer says that tangential torques are different but doesn't explain why, which is my question. The tangential torques are both $mg\sinθr$, where $r$ is the distance of the weights from the pivot and $θ$ is the angle of deflection with the horizontal. The centre of mass is also vertically below the pivot as no force is applied in the horizontal direction.