Deriving Lagrange equation with constraint

Question

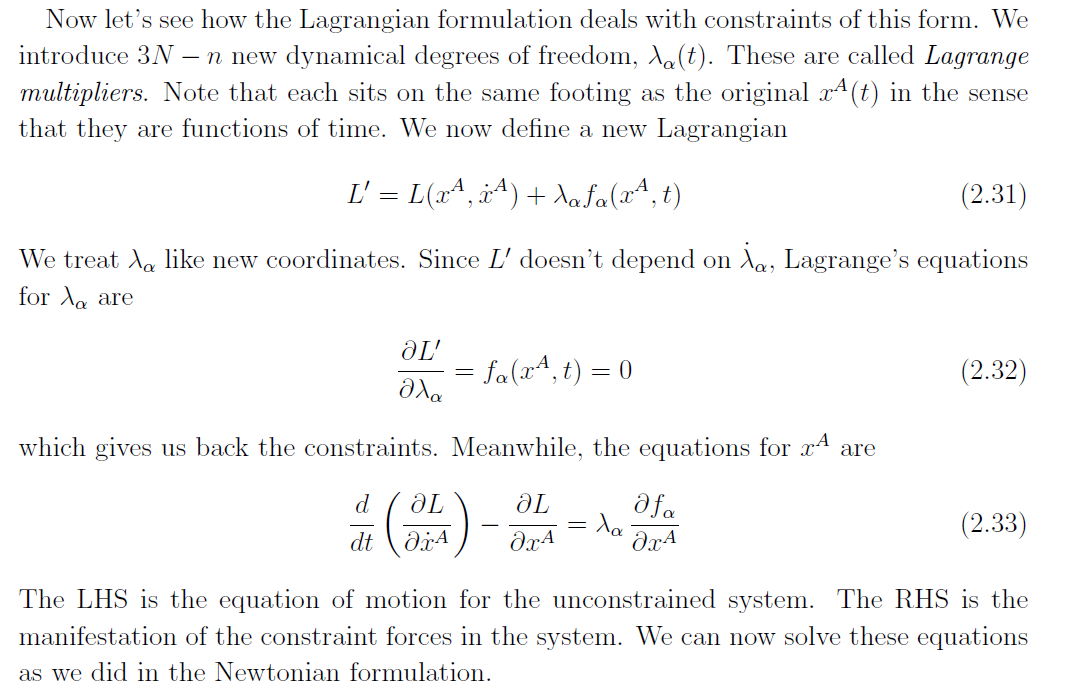
I'm having a hard time understanding the derivation of Lagrange equation from Newton's law when there is constraint (I'm ok with the basic case where there is only kinetic energy and potential function; I don't want to take least action principle to be the fundamental axiom). Many books use either virtual work (which I can't make sense of am still trying to translate into differential) or some obscure analysis on manifold (which I'm not well-versed in). I saw a proof in these Classical Mechanics notes by Tong. It is short but still very confusing to me. Why does (2.33) in the following screenshot give the equations for motion of $x^A$? They are Euler-Lagrange equations for $L'$, which is just some "random" Lagrangian that he writes down; why does $L'$ have any physical meaning?

Answer 1

1. In Ref. 1 the stationary action principle (SAP) for the old action $S~=~\int\! dt~L$ is treated as a first principle, so the new SAP $S^{\prime}~=~\int\! dt~ L^{\prime}$ in eq. (2.31) follows from the method of Lagrange multipliers.

2. Alternatively, in Ref 2. d'Alembert's principle (DAP) leads to Lagrange equations
   $$ \frac{d}{dt}\frac{\partial L}{\partial \dot{q}^j}-\frac{\partial L}{\partial q^j}~=~Q_j, \qquad j~\in \{1,\ldots, n\}. \tag{A}$$ A holonomic constraint $f(q,t)=0$ now has a generalized constraint force $$ Q_j~=~\lambda\frac{\partial f}{\partial q^j}\tag{B}$$ proportional to the gradient of the constraint function $f$. The Lagrange multiplier $\lambda$ is the proportionality factor. Such reasoning leads to eq. (2.33) in Ref. 1.

References:

1. D. Tong, Lectures on Classical Dynamics.

2. H. Goldstein, Classical Mechanics; chapter 1.