1
$\begingroup$

enter image description here

This is a screenshot from my textbook. It says the diver's angular momentum is conserved, and yet it is acted upon by gravity, and that would yield torque on the diver, wouldn't it?

But if we were to say the angular momentum of the diver is in fact, not conserved, how do we explain the increased spinning speed of the diver when he retracts his arms?

Edit:I doubt this is a duplicate question because I am asking why angular momentum can be conserved in the first place . The "duplicate question" assumes it is conserved, and wants to know why it looks as if it does not.

Thanks!

Improve this question
$\endgroup$
4
  • $\begingroup$ NOTE: A person is not a rigid body, and the joints can add/remove energy for the system. $\endgroup$
    – John Alexiou
    Commented Oct 18, 2022 at 13:24
  • $\begingroup$ Concerning your first question: it is possible to show that a uniform gravity field never exerts a net torque on a body about its center of mass, even if the center of mass is accelerating. So if we measure the angular momentum of a diver about their center of mass, it is in fact conserved. $\endgroup$
    – Michael Seifert
    Commented Oct 18, 2022 at 14:15
  • $\begingroup$ @MichaelSeifert how do you prove that? Could you provide a link to a similar proof? $\endgroup$
    – Lee Laindingold
    Commented Oct 18, 2022 at 14:25
  • $\begingroup$ See here: Torque due to Gravity. That question proves in general that for the purposes of torque, we can view the force of gravity over an extended body as though it acts at the CM; and if our origin is the CM, then $\vec{r} = 0$ and we have no torque. $\endgroup$
    – Michael Seifert
    Commented Oct 18, 2022 at 16:49

1 Answer 1

Reset to default
0
$\begingroup$

To answer this question, we need to first talk about torque.

The formula for torque $T$ is given by $ \vec{T} = \vec{r} \times \vec{F}$, where $\vec{F}$ is the force acted on the body and $\vec{r}$ is the position vector. Note that there is a subtlety in this equation. The value of the torque depends on your reference frame. This can be easily seen as $\vec{r}$ changes from coordinate system to coordinate system. However, in general, this can be solved by decomposing the motion into motion about the Center of Motion and Center of Mass motion.

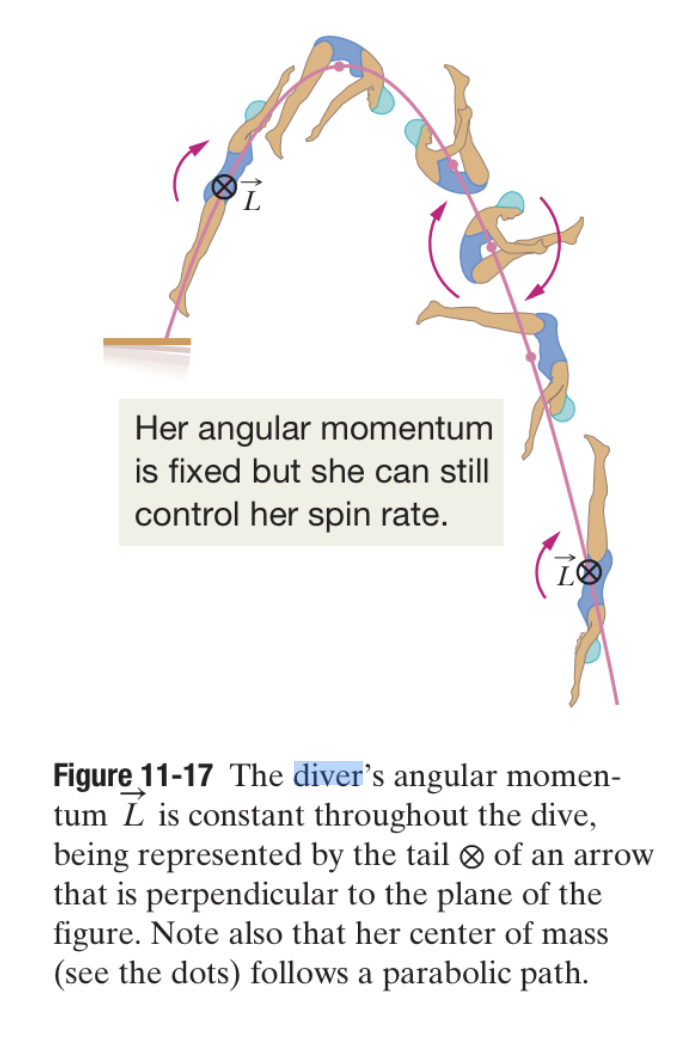
Let's look at the problem at hand. Imagine I am a bystander and the diver decides to jump. In my coordinate frame, the diver has a motion about its center of mass and the motion of its center of mass due to gravity. Now note that you are absolutely correct in the sense that there is a torque acting on the diver. However, this torque does not affect the motion about the center of mass. Gravity, generally, cannot make objects turn about their center of mass. The torque acting is actually affecting the motion of the center of mass. In this question, therefore, they are saying that the angular momentum about the center of mass is conserved, which is true as there is no torque.

Improve this answer
$\endgroup$
0

Not the answer you're looking for? Browse other questions tagged or ask your own question.