Suppose a spherical ball moving on a straight line struck an obstacle of rectangle shape(fixed) , once it tries to go upwards of it (assume it goes ), we say we can conserve angular momentum about that pointy edge about which it tries rotating , but in my opinion why will angular momentum be conserved as such no doubt impulsive torque would be zero about that point edge but what about gravity torque (for full duration from time of rotating and going fully upwards) and what about normal torque from ground at the time of striking ? They dont give zero torque about pointy edge so how is angular momentum conserved about pointy edge ?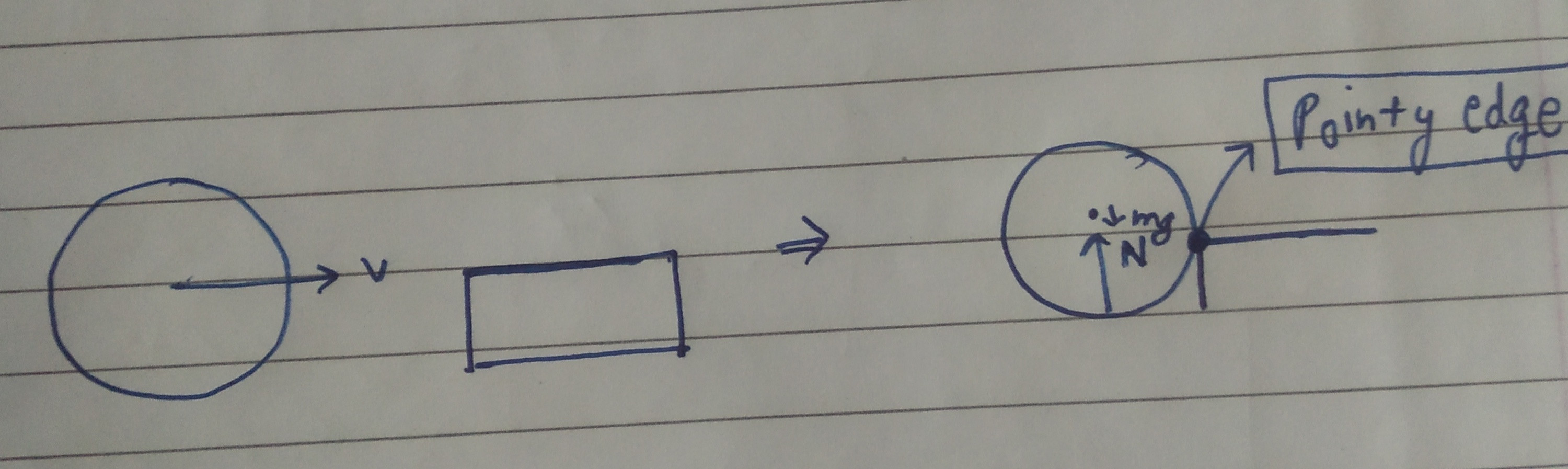 
$\begingroup$
$\endgroup$
2
-
$\begingroup$ "as such no doubt impulsive torque would be zero about that point edge" What does this sentence mean? $\endgroup$– SteevenCommented Dec 1, 2021 at 14:56
-
$\begingroup$ Normal force from point edge would be just impulsive(only one ) . So its torque is zero as the point of consideration is edge itself $\endgroup$– Orion_PaxCommented Dec 1, 2021 at 15:04
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
8
This setup needs to be thought of in three distinct phases: before the collision, a small time $\Delta t$ around the moment of the collision, and finally the motion after the collision while the ball is rising. Your question is not 100% clear about which phase you're concerned with, so I'll give a brief description of all three.
Before the ball hits the "pointy edge", gravity does indeed exert a torque on the ball if we take the "pointy edge" to be our origin point. So does the normal force from the surface it is rolling on, before it hits the edge. Since these two forces are equal and opposite and act along the same line, they exert opposite torques before the ball hits the pointy edge, and so angular momentum is constant with respect to this point.
In a small amount of time $\Delta t$ around the moment of the collision, the ball may experience an impulsive force from the pointy edge; this exerts no torque. In addition, in this brief amount of time, the torque from gravity will be approximately $m g \ell$, where $\ell$ is the horizontal distance between the edge and the center of the ball. This means that the change in angular momentum of the ball in this time will be approximately $m g \ell \Delta t$, which is negligible if we take the limit $\Delta t \to 0$. Thus, in the collision with the edge, angular momentum is conserved. This means that the angular momentum of the ball immediately after the collision is the same as it was long before the collision. (It is not immediately clear to me whether energy is conserved in the time $\Delta t$ around the collision; I suspect it is not.)
Finally, once the ball has lost contact with the surface below, the normal force vanishes, while gravity still exerts a torque. As before, the contact force exerts no torque on the ball. Thus, there is now a net torque on the ball, and the angular momentum with respect to the pivot point will no longer be constant. This means that we can't use angular momentum conservation to make statements about the motion of the ball while it is "tripping" over the edge. Energy is still conserved from the moment after the collision onwards, though. (This is important for some problems you might ask about this setup.)
answered Dec 1, 2021 at 15:12
-
$\begingroup$ If we assume friction is sufficient enough and there is no slipping at edge point , can we then say angular momentum is conserved about edgy point Sir or not ? Like in this video the problem at the starting ?youtu.be/hDf0NeYGZzQ and then they used that at 5:08 $\endgroup$ Commented Dec 1, 2021 at 15:17
-
$\begingroup$ And Sir u said energy still conserved but isnt contact force from edge impulsive so energy loss might happen isnt even if friction not there at edge? $\endgroup$ Commented Dec 1, 2021 at 15:21
-
1$\begingroup$ @Orion_Pax: See my edits, particularly the new third paragraph. I think you are concerned with a different phase of the problem than I was originally discussing. $\endgroup$ Commented Dec 1, 2021 at 15:32
-
$\begingroup$ Yeah Sir i fully satsified by your point, one thing i didnt get is how will energy get lost in that dt time as such friction (impulsive ) does no work etc isnt? And in that dt time normal from ground will not be taken into account for torque calc right Sir? $\endgroup$ Commented Dec 5, 2021 at 15:16
-
1$\begingroup$ (1) Yes. (2) In general, the forces in a collision are not as clear-cut as we make them out to be, and involve deformations of the body (during which work can be done.) I don't know whether energy is conserved in this specific collision, but I would not take it as given that it is conserved. $\endgroup$ Commented Dec 5, 2021 at 15:55
$\begingroup$
$\endgroup$
5
Angular momentum is in general conservated about any point, as long as all influencing torques are included. In your case, you can include the torque due to the pointy-edge-normal-force, and the torque due to gravity and the torque due to any other pushing forces etc. If all is included, then angular momentum is conserved.
Now, note that since we choose the point of rotation to be the pointy-edge itself, then the normal force from that pointy-edge does not cause any torque. You can include it just to be sure, but you will realise that it is zero (because the distance between force and rotation point is zero). So the only relevant torques are that of gravity and that of the any pushing force. Calculate these torques about the pointy edge and all should work out just fine.
-
$\begingroup$ Here they said angular momentum is conserved about edge point , its wrong then right Sir ? youtu.be/hDf0NeYGZzQ at 5:08 $\endgroup$ Commented Dec 1, 2021 at 15:14
-
1$\begingroup$ @Orion_Pax Why would that be wrong? As I stated in my answer her: Angular momentum is in general conservated about any point. Also about the pointy edge. That statement in the video is fully correct. $\endgroup$– SteevenCommented Dec 1, 2021 at 15:58
-
$\begingroup$ Why as such gravity torque is still acting isnt (non zero about edgy point) ? Also for a small dt time N which i depicted in figure give non zero torque isnt? $\endgroup$ Commented Dec 1, 2021 at 16:17
-
$\begingroup$ @Orion_Pax I'm sorry but I don't understand what you are asking. As the ball rolls over the edge, gravity will cause a non-zero torque about that edge. Would you mind clarifying your question? $\endgroup$– SteevenCommented Dec 1, 2021 at 16:25
-
$\begingroup$
$\endgroup$
1
the angular momentum of the ball is made up of two components.
- The spin. The normal contact force on the sphere acts perpendicular to the surface of the sphere. It therefore passes through the middle of the sphere and has a torque of zero.
The force of gravity and the normal force from the ground also act through the middle of the sphere.
Since there is no force producing a torque on the sphere (during rolling or impact), so its spin angular momentum is unchanged.
- However there is a component of angular momentum due to the translational velocity. When the ball leaves the ground, then gravity does change the translational angular momentum of the ball around the point edge.
answered Dec 1, 2021 at 14:54