Why is the angular momentum conserved with relation to this point?

Question

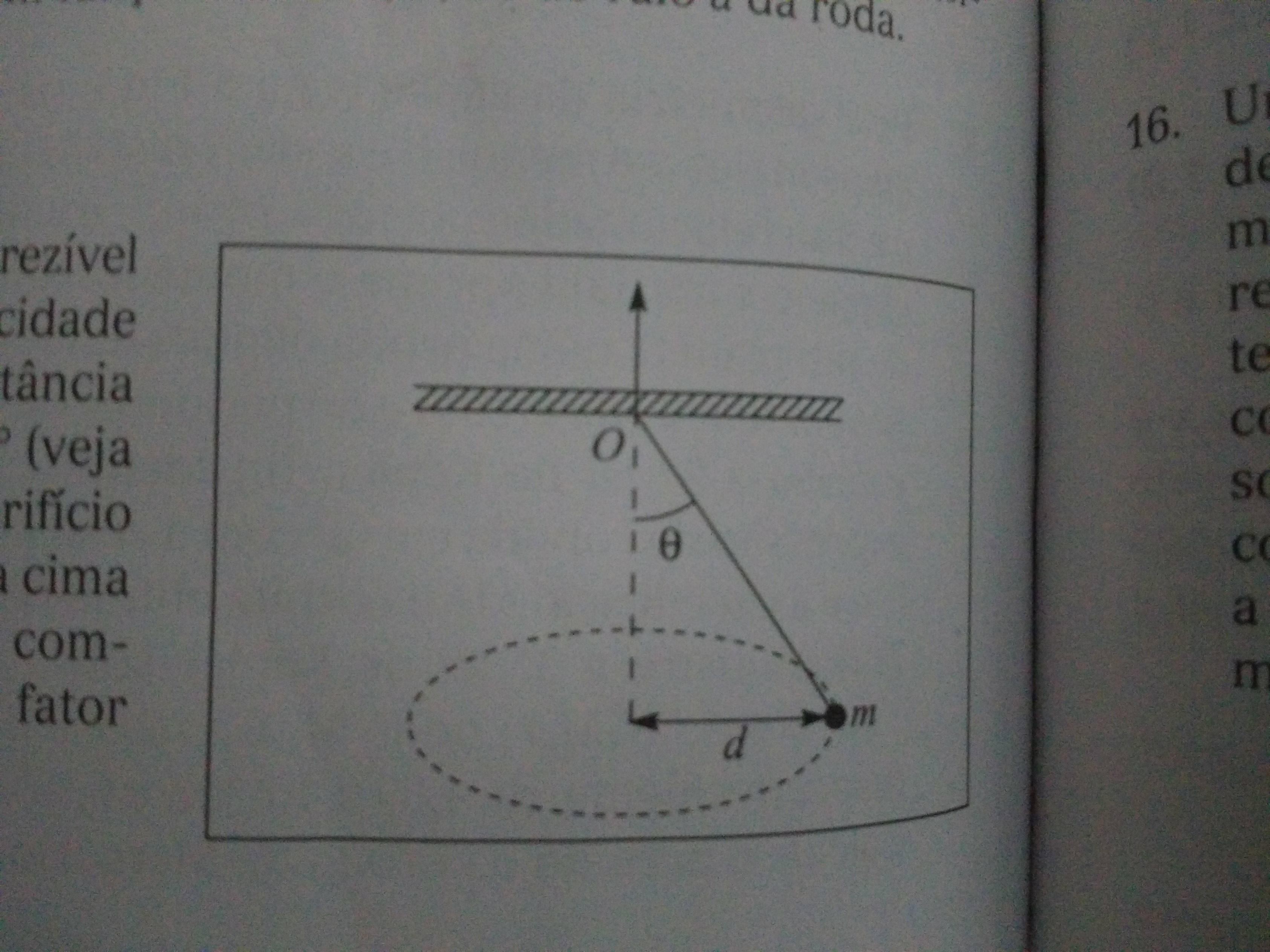
I have this system:

It is a given that the mass $m$ rotates around the vertical axis with constant speed, and that $\theta = 30^\circ$ initially. The massless string is then pulled upwards, until the angle $\theta$ becomes $60^\circ$.

The problem then asks about some quantities, but a key part of the solution is that the angular momentum of the mass $m$ with relation to the point around which it rotates (that is, the center of the dashed circle, not the point $O$ at the ceiling) is conserved. But why is that? That doesn't seem obvious to me at all.

In fact, my understanding is that the angular momentum with relation to $O$ would be conserved: indeed, when we pull the string upwards, we're only creating a tension force $\mathbf{F}$ in the direction of the string. Therefore the vector $\mathbf{r}$ connecting $O$ to $m$, and the vector $\mathbf{F}$, are parallel. That means the torque $\mathbf{\tau} = \mathbf{r} \times \mathbf{F}$ applied to $m$ with relation to $O$ would be zero, so angular momentum must be conserved. What part of that reasoning is flawed? Why is the momentum with relation to the center of the dashed circle (around which $m$ rotates) conserved instead?

Answer 1

The answer is very simple, but only after realising one thing.

The key is that you are not taking the weight into account. The mass does have a weight downwards. Now, the point is that the tension force is such that the resultant force points to the centre of the dashed circle.

That is the idea that is often non mentioned, and it is fundamental to understand this kind of problems. We do know that the ball rotates around the circle. If the ball rotates around it, there must exist one only centripetal force. If there were other forces, the trajectory wouldn't be that circle. Consequently the only force must be the centripetal one, and so, the tension must be adjusted so that the resultant $\vec{F_{tens}}+\vec{F_{weight}}=\vec{F_{cp}}$

Now, if you see that there is only one resulting force, pointing only at the centre of the circle, you can see that $\vec{L}$ is conserved with respect to that point.