The explanation of the banking angle of the road is said to be that one part of the normal force exerted by the road on a moving object neutralizes the object's weight and another part is providing the centripetal force necessary to turn the object in a circular path. So the normal force is greater than the weight of the object. What is the source of this force? Why is this force greater than the weight?
3
-
1$\begingroup$ You should add a picture to it $\endgroup$– Debanjan BiswasCommented Oct 9, 2022 at 7:16
-
1$\begingroup$ That's a nice question, a suggestion: Put "What is the source of this force" in bold. $\endgroup$– GedankenExperimentalistCommented Oct 9, 2022 at 16:58
-
$\begingroup$ @PhilipWood This may help. $\endgroup$– Debanjan BiswasCommented Oct 11, 2022 at 4:47
Add a comment
|
7 Answers
$\begingroup$
$\endgroup$
If the car is on the flat the normal force and the weight of the car are equal in magnitude and opposite in direction.
When going around a corner more force is needed so that there is a contribution from the force to provide the centripetal acceleration.
More force manifests itself as an increase in the normal force so that the vertical component balances the weight (as on the flat) and the horizontal component produces the centripetal acceleration.
$\begingroup$
$\endgroup$
The source of this force is the push of the road itself which makes the car go in a circle when it is in motion trying to go forward (in a straight line) and the force only arises when the car is in motion, not when it is stationary. so the road pushes the car perpendicular to its orientation whose vertical component is equal to the weight of the car and the horizontal component is the centripetal force.
$\begingroup$
$\endgroup$
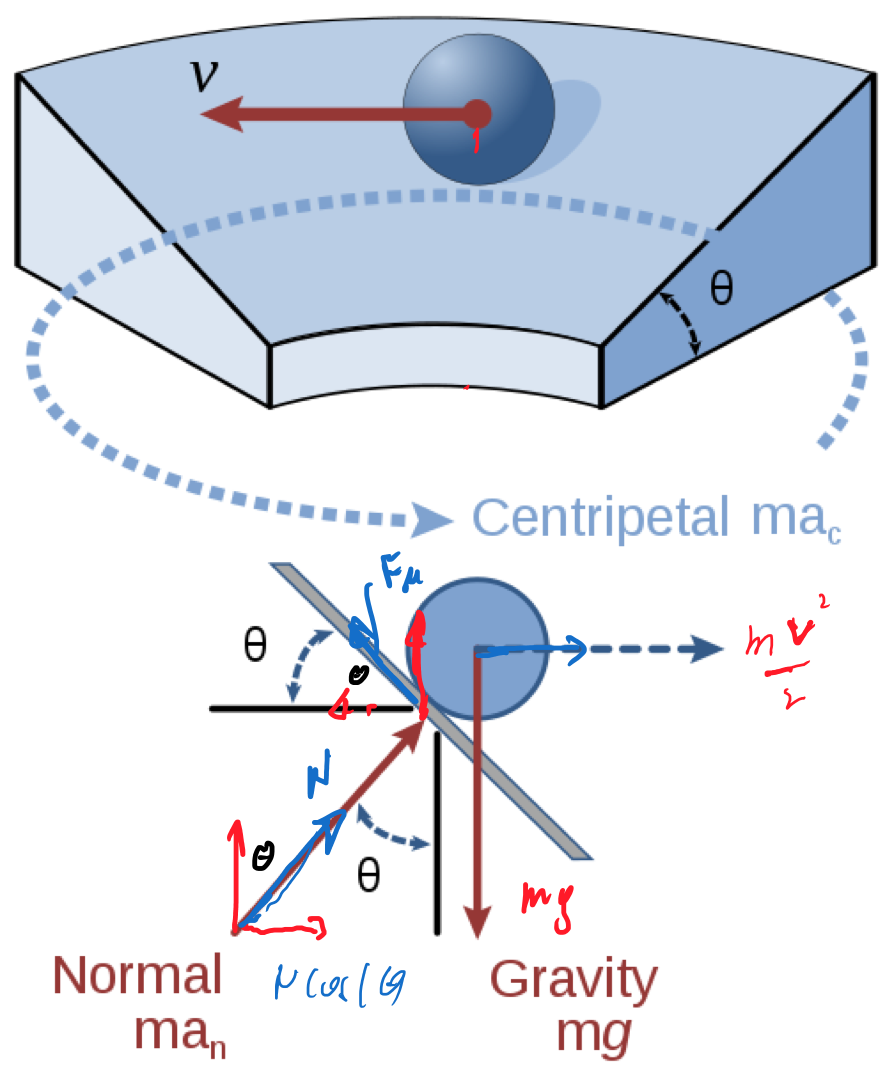
If the bank is on a bend that is an arc of a circle then there must be a centripetal force directed horizontally towards the centre of circle to make the car follow the bend instead of travelling in a straight line. The car's weight cannot provide this centripetal force since the weight acts vertically and has no horizontal component.
If there is no friction between the car and the roadway then the only other force acting on the car is the normal force from the roadway. So the horizontal component of the normal force must equal the centripetal force required to make the car follow the bend. This is what determines the size of the normal force. So the size of the normal force depends on the angle of the bank, as well as the car's mass and speed and the radius of the bend. The relevant equation is
$\displaystyle N_{h} = \frac {mv^2} r$
where $N_h$ is the horizontal component of the normal force, $m$ is the car's mass, $v$ is the car's speed and $r$ is the radius of the bend. If the bank is at an angle $\theta$ to the horizontal then we know that $N_h = N \sin \theta$ and so
$\displaystyle N = \frac {mv^2} {r \sin \theta}$
The vertical component of the normal force may be less than or greater than the car's weight, depending on the angle of the bank. If the vertical component of the normal force is less than the car's weight then the car will slide down the bank. If the vertical component of the normal force is greater than the car's weight then the car will slide up the bank. If the angle of the bank (together with the car's speed and the radius of the bend) is such that the vertical component of the normal force is exactly equal to the car's weight, then the car will stay at the same height on the bend.
The vertical component of the normal force is
$\displaystyle N_v = N \cos \theta = \frac {mv^2} {r \tan \theta}$
In a typical question you are given the car's speed and the radius of the bend, and asked to find the bank angle for which the vertical component of the normal force equals the car's weight. To find the correct angle you must solve
$\displaystyle N_v = mg \\ \displaystyle \Rightarrow mg = \frac {mv^2} {r \tan \theta} \\ \displaystyle \Rightarrow \tan \theta = \frac {v^2} {gr}$
Note that $m$ cancels out in the final equation, so the bank angle for which the car stays at the same height does not depend on the car's mass.
$\begingroup$
$\endgroup$
1
from the free body diagram you obtain two equations
$$N\cos(\theta)=m\,g\tag 1$$ $$N\sin(\theta)=\frac{m\,v^2}{r}\tag 2$$
those equations described a steady state circular motion (velocity v is constant) where the road has a banking angle $~\theta~$
according to equation (1) the source of the normal force $~N~$ is the weight. and with $~N=\frac{m\,g}{\cos(\theta)}~$ , N is grater then $~m\,g~$ because the banking angle $~\theta~$
-
$\begingroup$ A physical explanation will be better rather than mathematical $\endgroup$ Commented Oct 9, 2022 at 17:52
$\begingroup$
$\endgroup$
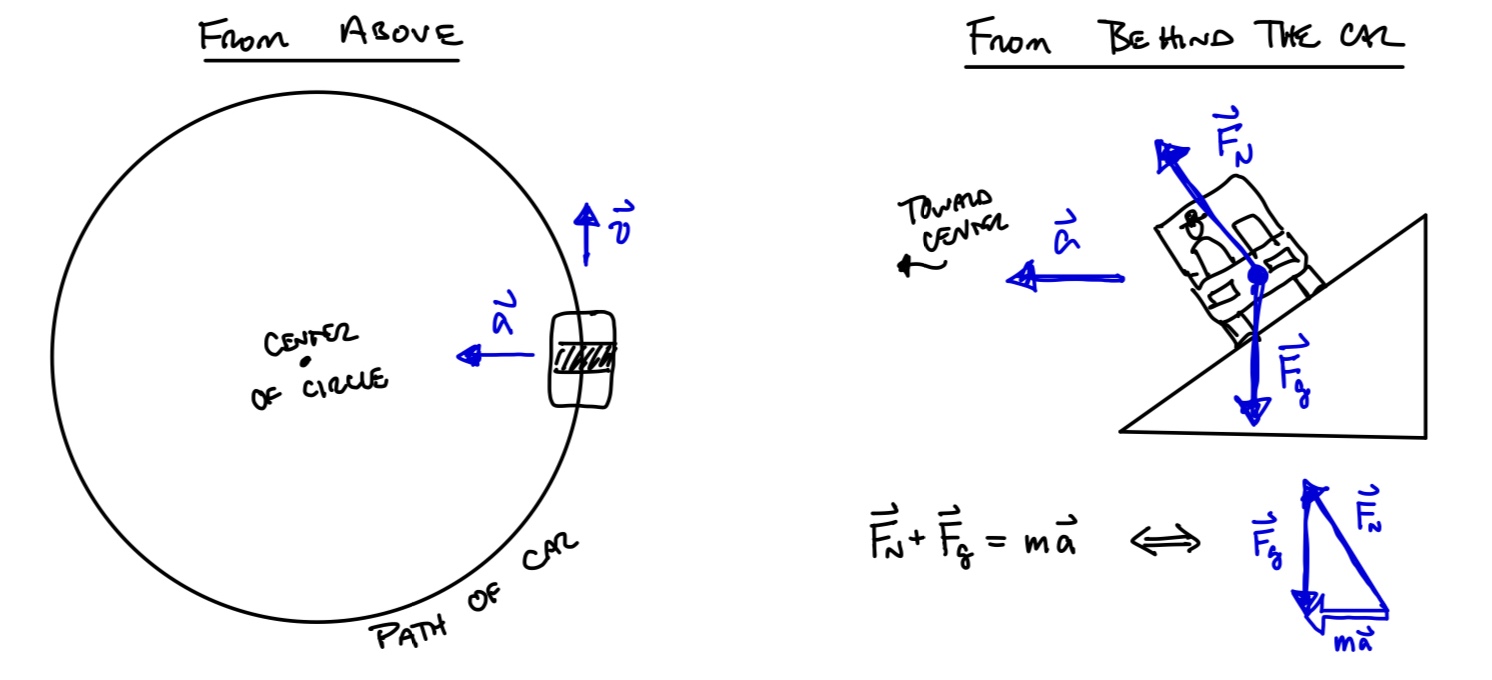
In this problem we assume the kinematics: The car travels at constant speed on a banked curve and its trajectory makes a horizontal circular path, implying that the acceleration vector is also horizontal and points directly into the center of the circle.
If there is no friction on the banked curve then the only forces are gravity and normal and Newton's Second Law tells us that: $$ \vec{F}_N + \vec{F}_g = m \vec{a} $$ Drawing this as a vector addition, the normal force will lie along the hypotenuse of a right triangle with $m\vec{a}$ horizontal and $\vec{F}_g$ vertical:
So we see that the normal force being larger than gravity is a consequence of our requirements about the kinematics of the car: because the acceleration points horizontally inward, the normal and gravitation forces must sum (as vectors) to a horizontal vector.
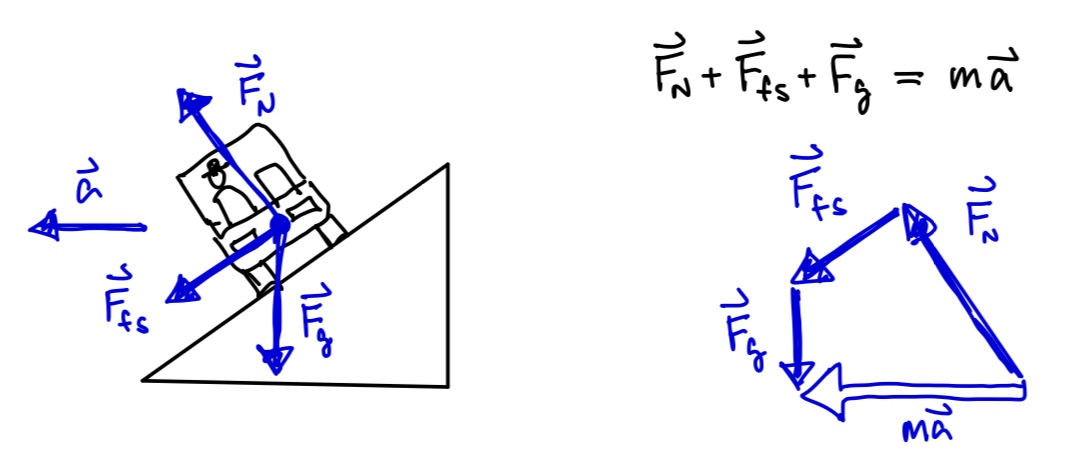
If there is static friction acting on the car, then it is easy to see that the normal force will still be larger than the gravitational force:
Now, to your questions: What is the source of this normal force? Why is it larger than the weight? The source of the normal force is simply from contact with the road. Its magnitude is that which is required for the car to remain on a circular path. The statement about circular motion is: "if an object travels on a circular path, then it has an acceleration that points inward with radial component $v^2/R$." So the normal force has the necessary magnitude to get the assumed inward-pointing acceleration. We started the problem by assuming that the car was traveling on a horizontal circular path. Therefore the acceleration points horizontal and inward. And therefore, by Newton's Second Law (demonstrated in the vector addition above), the normal force must have that relation to the gravitational force.
Here is a simpler example. If you are standing on the ground, the normal force pushing up on your feet is equal to the gravitational force pulling you down. But if you wish to leap up into the air, then you press harder on the ground, which (by Newton's Third Law) increases the normal force on you. Then the normal force on you is larger than your weight and you get an upward acceleration vector that takes you off the ground.
As a side note: For the case of no friction, you can easily see by solving Newton's Second Law while setting $|\vec{a}| = v^2/R$ that, for a road with a given radius of curvature, $R$, there is one single speed, $v$, which can allow the assumed motion. If the car were to drive more slowly it would slide down the bank; if it drove more quickly it would slide up the bank. If there is friction then there is a range of possible speeds, determined by the coefficient of static friction.
$\begingroup$
$\endgroup$
The force is owing to electromagnetic repulsion between stone (or tarmac) road surface and rubber of car wheels. You can tell the force must be there because the car is accelerating in the horizonal direction (towards the centre of a circle as it goes around the corner) and also not accelerating (relative to the ground) in the vertical direction even though there is a vertical gravitational force on it.
answered Dec 2, 2023 at 21:24
$\begingroup$
$\endgroup$
There are many elaborate answer available already but I think you are doing fundamental mistake in your question, you are thinking that one component of force is balancing the weight and other component is providing centripetal force it's not really correct! It's friction which is very important here!
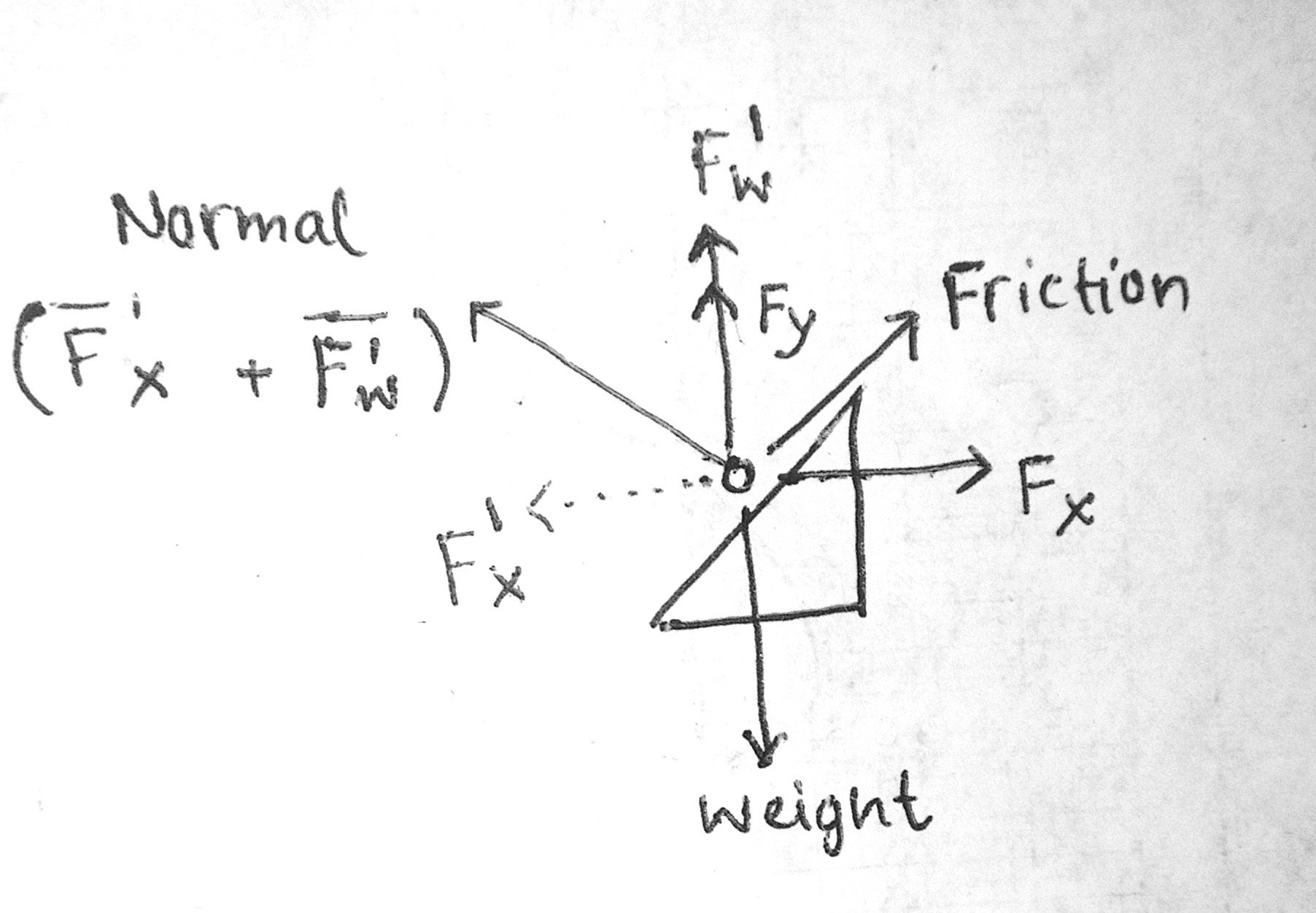
Normal force is reaction force it can't be there without action forces ! Now what are action forces in this scenario? The weight of body and friction between it and road. The friction is acting along the inclined plane, it's one component Fx is along x axis and other Fy along y axis. Weight is solely along negative y axis. Let's assume some normal force balancing the net force. The normal force has two components F'X along negative x axis and F'w along positive y axis. The Fy and F'w should add up to balance weight and F'x should be equal to Fx. So we have magnitude and direction of normal force if we equate the forces.
From doing vector addition you can confirm that why normal force is greater than weight (weight is contributing just one component of normal force so obviously it's going to be smaller than net!)
