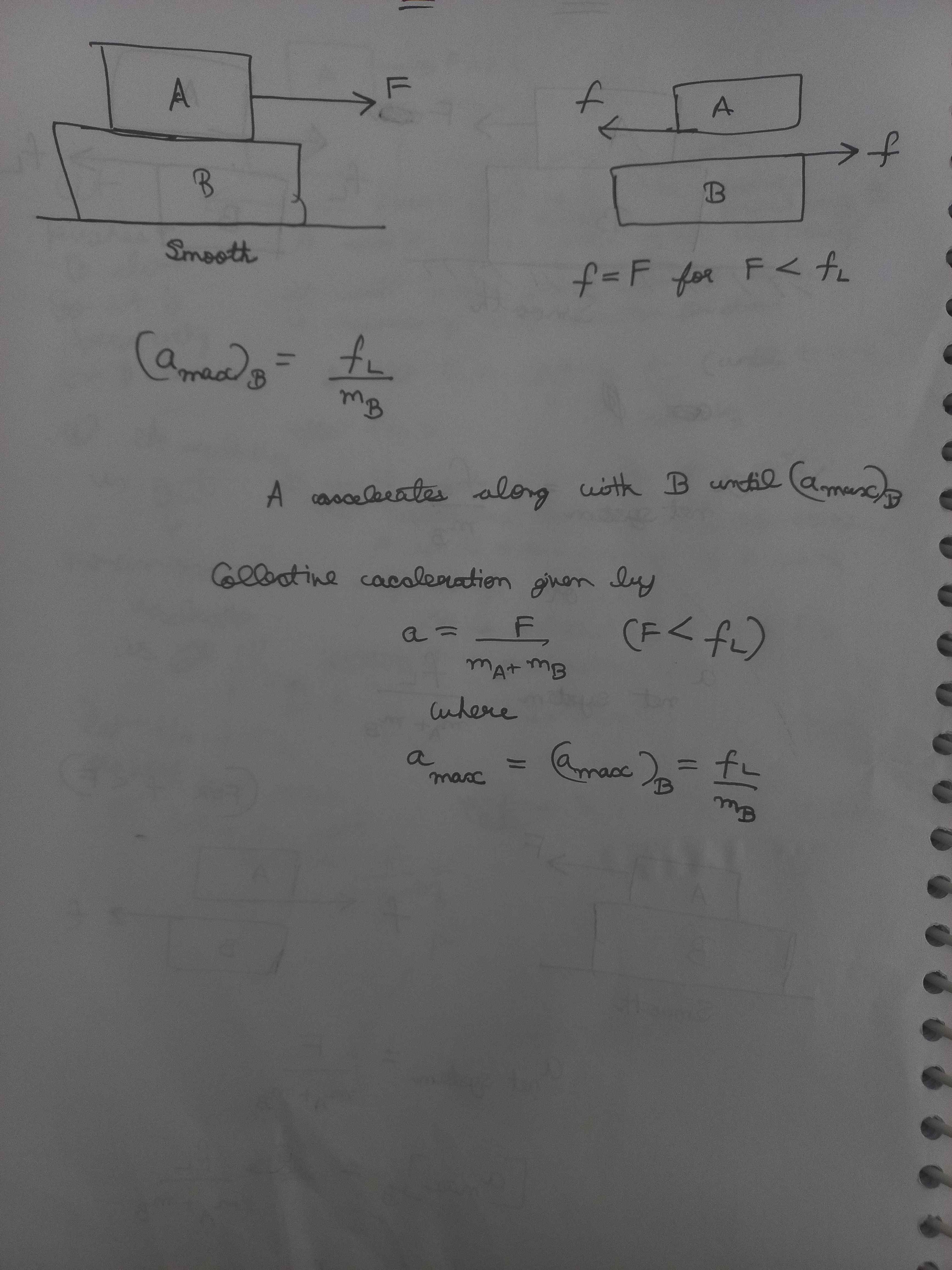
Suppose A and B experience friction with each other but they move on a smooth floor. A is given a force F which is not enough to overcome friction; a frictional force equal to F, thus, acts on B. Now, one would expect both the objects to move together with a common acceleration, due to this friction. In which case, it can be said that
$\frac{F-f}{m_A}=\frac{f}{m_B}={a_A}={a_B}$
But this would imply that the accelerations of A and B are zero as $F=f$ for $F<{f_L}$. So does that mean that a force exerted on A that is less than the limiting value of static friction, will not result in collective acceleration of A and B?
This seems to contradict empirical behavior. For example, in case of a sufficiently heavy object on top of another, the application of a force that is not sufficient for the top object to overcome friction against the bottom object, still results in a collective acceleration.
I have attached a photo containing diagrams for reference.