4
$\begingroup$

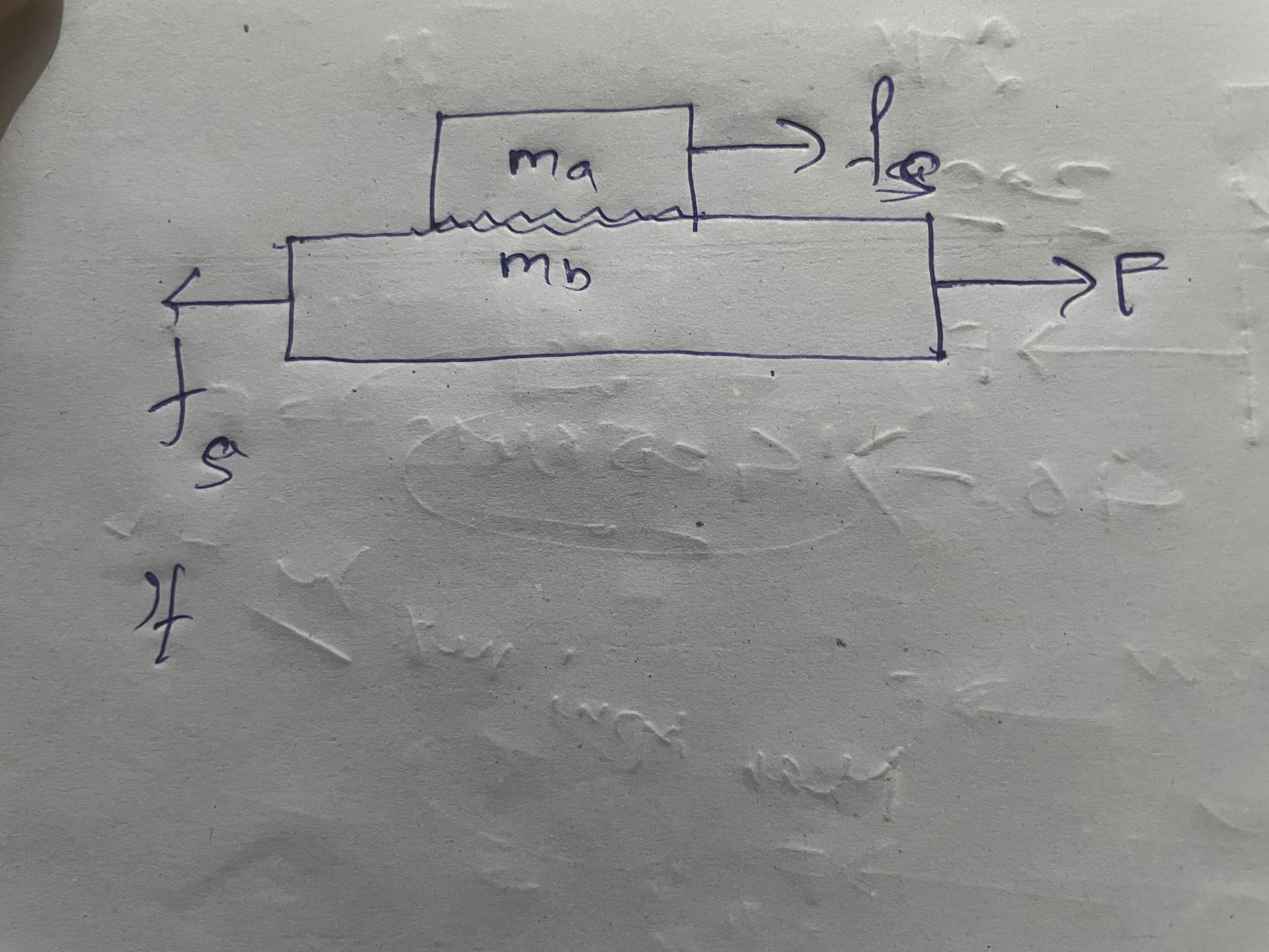
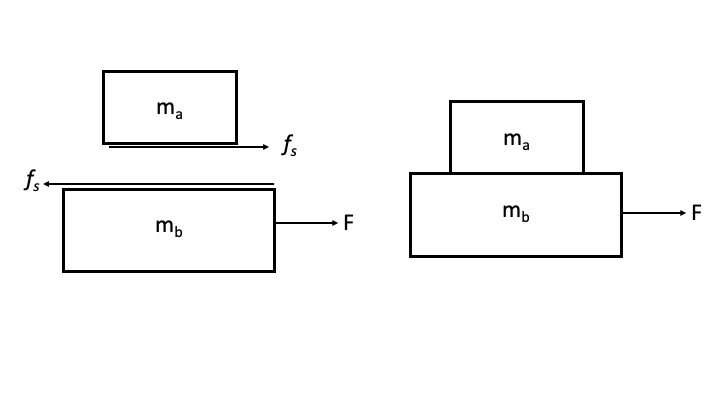
For the two-block problem below, why is the static friction not equal to applied force when both blocks move with same acceleration?

I mean that in physics it’s taught that static friction is always equal and opposite to the applied force, so that the body can’t move due to friction.

But in case of this two block problem, one block on top of the other, the static friction is not the same as the applied force, when the body is not slipping.

enter image description here

My exact question is how can the applied force is not equal to friction force , because the friction force is always equal and opposite in case of static friction , till the applied force is under limiting friction

Improve this question
$\endgroup$

3 Answers 3

Reset to default
3
$\begingroup$

I mean that in physics it’s taught that static friction is always equal and opposite to the applied force, so that the body can’t move due to friction.

You need to be careful in identifying the "applied force" and that the meaning of "can't move" means there can't be relative motion between the two blocks, not that the blocks can't move.

See bottom left figure below (only horizontal forces shown). The static friction force that block $b$ exerts on block $a$ is the only horizontally "applied" force on block $a$. Per Newton's 3rd law, the static force that block $a$ exerts on block $b$ is equal and opposite to this "applied" force.

See bottom right figure below. In this figure the static friction forces are between the blocks and are thus internal to the two block system. The only external "applied" force on the system is $F$. Since (based on your diagram) there is no static friction on the surface below, there is no equal and opposite static friction force to the applied force $F$ which would prevent relative motion between the two block system and the supporting surface.

Hope this helps

enter image description here

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ We seem to enjoy answering the same questions. Your answers are usually better than mine. Cheers! $\endgroup$
    – Mariano G
    Commented Aug 21 at 14:07
3
$\begingroup$

My understanding is that the only friction source is between the two blocks, gravity is included, and there is a friction coefficient $\mu$.

I think your confusion is about how to put forces on a free body diagram (FBD) consistently.

If the bodies accelerate together, you can treat them as one system and write $F = (m_a + m_b) a$ where $a$ is the acceleration of both blocks due to force $F$. In this case, the friction force $f_s$ is internal and does not appear in the FBD at all. But you need to check that the required friction force $f_s$ is less than $\mu m_a g$, or else you have a slipping condition which is inconsistent with your assumption.

You can also separate the two bodies and write two separate FBDs. In that case, each body will see the force $f_s$ in the opposite sense.

In any case, the answer should be the same. The force $F$ has to accelerate both blocks, not just the upper one. So it is not going to be equal to $f_s$ except maybe in some special case $m_b = 0$. Or something like that.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ LIke yours too. +1 $\endgroup$
    – Bob D
    Commented Aug 21 at 14:11
0
$\begingroup$

If the frictional force on the bottom block was equal in magnitude to the applied force and acted in the opposite direction to the applied force, the net force on the block would be zero and the bottom block would not be accelerating but moving with constant velocity or being at rest.

The static frictional forces are there to prevent relative movement between the two block.

As the blocks are not moving relative to one another they must have the same acceleration.

The only force accelerating the top block is the frictional force due to the bottom block to the right with magnitude $f_{\rm s}$ with equation of motion $f_{\rm s} = m\,a$.

There are two forces acting on the bottom block: the applied force to the right, $F$ and a frictional force to the left $f_{\rm s}$ the N3L pair to the frictional force acting on the top block.
The net force on the bottom block $F-f_{\rm s}$ to the right must produce the same acceleration of the bottom block as force $f_{\rm s}$ does on the top block, with equation of motion, $F-f_{\rm s} = M\, a$

Note that you add the two equations of motion $(f_{\rm s} = m\,a)+ (F-f_{\rm s} = M\, a) \Rightarrow F=(M+m) \,a$ which is the equation of motion treating the two blocks as the system.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I knew this , but my question is , as we have studied , in case os static friction , its value is equal to applied force . But here applied force and static friction is different , why so ? Because static friction stop the body moment , till it reach the limiting force $\endgroup$
    – Asit Purohit
    Commented Aug 26 at 11:01
  • $\begingroup$ Static frictional forces try and prevent relative motion between two bodies. $\endgroup$
    – Farcher
    Commented Aug 26 at 19:07

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.