My ultimate goal is to understand why the spatial coherence of a wave increases with distance from the wave source. From what I have read though, there seems to be conflicting definitions of spatial coherence which lead me in different directions.
Over here at RP-Photonics, Spatial Coherence is defined as "a fixed phase relationship between the field at different locations".
In Chapter 12 of Basics of Coherence theory from Purdue (linked at the end of the post), Spatial Coherence is defined as "the correlation of the phase of a wave between different locations in space." The same source then goes on to say that the spatial coherence of a wave refers to the distance over which the wave fronts remain flat. These definitions and the implication seem to contradict each other though. For suppose we have 4 in-phase and monochromatic spherical wave emitters displaced from each by a few wave lengths as shown below.
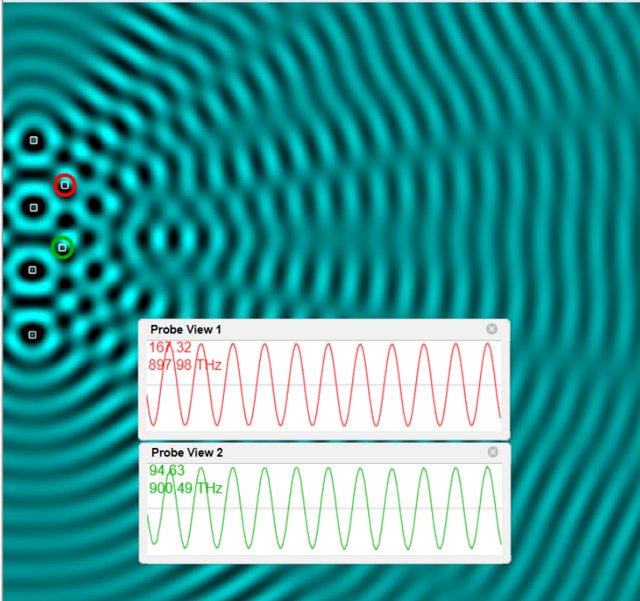
Then for any two points in the wave field (like the two probe points in the image), there will be a "fixed phase relationship". No matter were I place the two probes, I will always get 2 sine waves of the same frequency which may be in-phase or out-of-phase, but the phase difference between them will remain fixed in time provided the probes are kept in place regardless of how far or close they are to the sources or from each other. Thus, if spatial coherence is defined as a fixed phase relationship between two points, then the spatial coherence of a wave does not increase as we move further from its source, it simply stays the same. But notice that if we take a high degree of spatial coherence to mean "the distance over which the wave fronts remain flat", then it is clear that the spatial coherence of the wave in my image does in fact increase with distance from the source. How can this be if for any two points in the field, we will always have a fixed phase relationship?
Given these facts, is my image an example of a spatially coherent wave field? If so, does the degree of its spatial coherence increase the further we move away from the source and why?
Basics of coherence theory link below: https://www.physics.purdue.edu/webapps/index.php/course_document/index/phys322/1460/25/11076#:~:text=1)%20For%20producing%20stable%20pattern,pattern%2C%20coherent%20sources%20are%20required.
