I assume in your figure the red lines represent the phase profile of the wave (the points where the phase is constant), and not the intensity. And that the wave propagates from top to bottom.
Is the following wave spatially coherent? Yes.
If those lines were straight, the wave would be a plane wave, perfectly coherent in time and space.
I consider then your plot as a propagating wave with a periodically undulated phase profile. Again, it's perfectly coherent in time and space.
The definition of coherence which I consider accepted says that a field is coherent where (when) there is a fixed phase relation between the electric field in different locations (times).
Mathematically this is described by the cross correlation of the field at two points in space or time.
Experimentally, we measure the intensity $|E|^2$, not the field $E$, so coherence is tested using interference as follows:
Spatial coherence: you measure the intensity fringe contrast (max-min) in the double slit experiment, as a function of the distance between the slits. The maximum distance between the slits at which there is still some interference is the spatial coherence length of the wavefront (the contrast depends on the phase correlation between two different points in space).
Temporal coherence: in an interferometer with 2 different arm lengths (e.g. Michelson) each point, or beam, of the wavefront interferes with a delayed copy of itself. The maximum delay (difference between the lengths of the 2 arms) at which there is still some interference is the temporal coherence length of the field.
Applying the above 2 criteria to your wave, you can see that it's both time and space coherent. This will hold true if you distort the red lines even more (you can make them random), IF they remain one identical to the others and equispaced (like the figure below, left). To decrease the temporal coherence, you can make the distance between the lines, i.e. the wavelength, more randomly changing (fig. below, middle). In this way, the phase difference $\Delta \phi$ between the field $E(x_o,t)=P1$ and the field $E(x_o,t+\tau)=P'1$ is not constant anymore, and the spectrum is not a delta but a broader peak. To decrease the spatial coherence, while keeping the temporal one, you can change the wavelength across the wavefront (fig. below right).
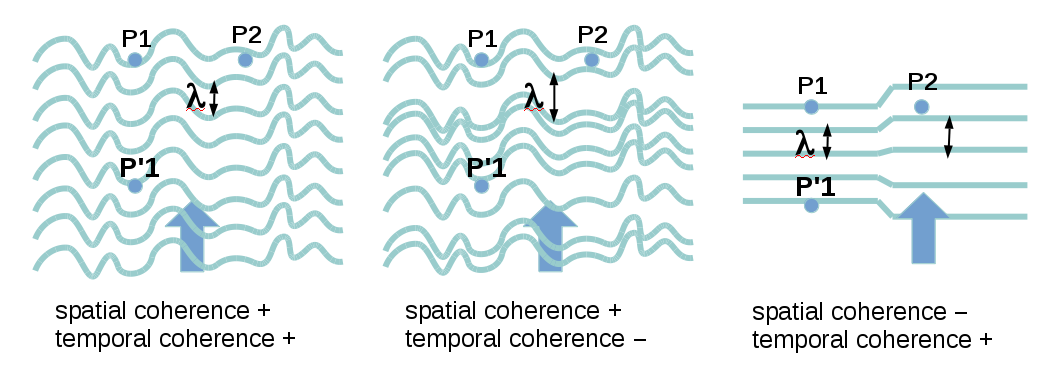
Probably a useful way to see if a wave is coherent is to consider the local wavelength $\lambda(x,t)$, and asking if it constant in $t$ (vertical direction in the figure) and / or in $x$ (horizontal direction in the figure), etc.