Acceleration of points on a wheel

Question

In Halliday's "Fundamentals of Physics", it is said that we can view rolling "as pure rotation about an axis that always extends through the point where the wheel contacts the street as the wheel moves." However, then when calculating acceleration of points from this frame of reference (the axis at a point of contact), I am getting a different and obviously wrong answer, since the acceleration of points (for a wheel rolling at a constant speed) should be the same from all reference frames.

From the rider's reference frame, the magnitudes of acceleration of the points at the top and at the bottom are: $a_{top}=a_{bottom}=\frac{v^2_{COM}}{R}$, and $a_{center}=0$.

However, viewing the rolling as pure rotation, aren't we then supposing that the bottom point is fixed, hence its acceleration is $a_{bottom}=0$. In other words, as viewed by a stationary observer, its momentarily tangent speed is $0$ (since we are considering pure rotation), hence the normal acceleration is also $0$. Similarly, $v_{center}=v_{COM},$ thus $a_{center}=\frac{v^2_{COM}}{R}$; $v_{top}=2v_{COM},$ thus $a_{top}=\frac{(2v_{COM})^2}{(2R)}=\frac{2v^2_{COM}}{R}$ $\implies \impliedby$.

Answer 1

its momentarily tangent speed is 0 (since we are considering pure rotation), hence the normal acceleration is also 0

This is an incorrect argument. A velocity of zero in no way implies an acceleration of zero. Also, the tangential speed and the centripetal acceleration are only related by that simple formula in the frame where the axle is at rest.

To calculate the acceleration you must take $\frac{d^2}{dt^2}x$. There is no shortcut by calculating only $\frac{d}{dt}x$, even for rolling.

For the point $\phi$ on the edge of the wheel the position is $$\left( r \cos(\omega t+\phi)+r\omega t,r \sin(\omega t +\phi) \right)$$

The acceleration and velocity can easily be calculated, but it is clear that the $r\omega t$ term has a non-zero first derivative but a zero second derivative

Answer 2

Using an inertial frame, for any two points P and Q in a rigid body, it can be shown that $$(1) \vec v_P = \vec v_Q + \vec \omega \times \vec r_{QP}$$ and that $$(2) \vec a_P = \vec a_Q + \dot {\vec \omega} \times \vec r_{QP} + \vec \omega \times (\vec \omega \times \vec r_{QP})$$ where the $\vec v \enspace 's$ are velocities, $\vec r_{QP}$ is the position vector from Q to P, and the $\vec a \enspace 's$ are accelerations. [Ref 1]

Consider a wheel rolling without slipping along a level surface with constant angular velocity $\vec \omega = -\omega \hat e_3$ as indicated in Figure 1 below. Point A is at the center of mass (CM) of the wheel, and point B is the point on the rim of the wheel that instantaneously contacts the ground.

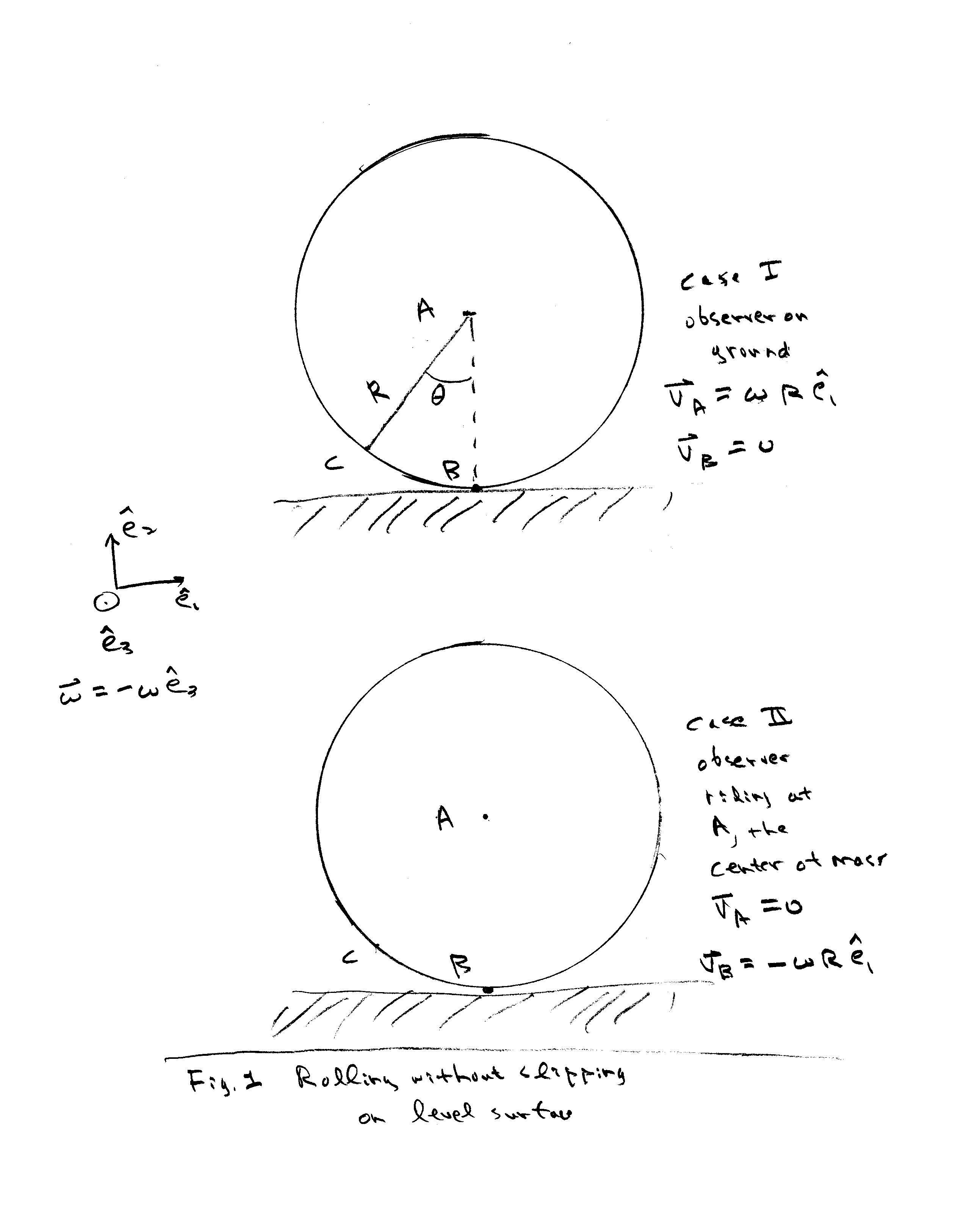
Consider an observer on the ground as indicated in Case I of the Figure. With no slip, point A, the center of mass (CM), moves at constant velocity $\vec v_A = \omega R \hat e_1$.

Consider an observer fixed at point A as indicated in Case II of the Figure. Point A, the center of mass (CM), has zero velocity $\vec v_A = 0$.

For Case I, using (1) with Q as A and P as B, Point B has velocity, $\vec v_B = \omega R \hat e_1 - \omega R \hat e_1 = 0$. For Case II, point B has velocity $\vec v_B = 0 - \omega R \hat e_1 = - \omega R \hat e_1 $.

For this situation, $\dot {\vec \omega} = 0$. Observers in both Case I and Case II are in inertial frames and the acceleration of the CM, point A, is zero for both observers. For either Case I or Case I, using (2) with Q as A and P as B, point B has acceleration $\vec a_B = 0 + 0 + \omega ^2 R \hat e_2$.

Now, consider a point C on the rim of the wheel not in contact with the ground, as indicated in the Figure; C is at $\theta (t)$. For either Case I or Case II, using (2) with Q as A and P as C, point C has acceleration $\vec a_C = 0 + 0 + \vec \omega \times (\vec \omega \times \vec r_{AC}) = \omega ^2 R \enspace sin \theta \enspace \hat e_1 + \omega ^2 R \enspace cos \theta \enspace \hat e_2$. $\vec a_C$ is parallel to $\vec r_{AC}$, points from C to A, and has magnitude $\omega ^2 R$. For any point C on the rim of the wheel, the acceleration is a centripetal acceleration directed from the point to the CM. (This is not the case if the CM of the wheel is accelerating. Also, if the CM is accelerating Case II is no longer an inertial frame.)

As expected, the acceleration of any point is the same for both Case I and Case II since both are inertial frames.

Ref 2. https://ethz.ch/content/dam/ethz/special-interest/mavt/mechanical-systems/mm-dam/documents/Notes/Dynamics_LectureNotes.pdf