Wavefunction Amplitude Intuition

Question

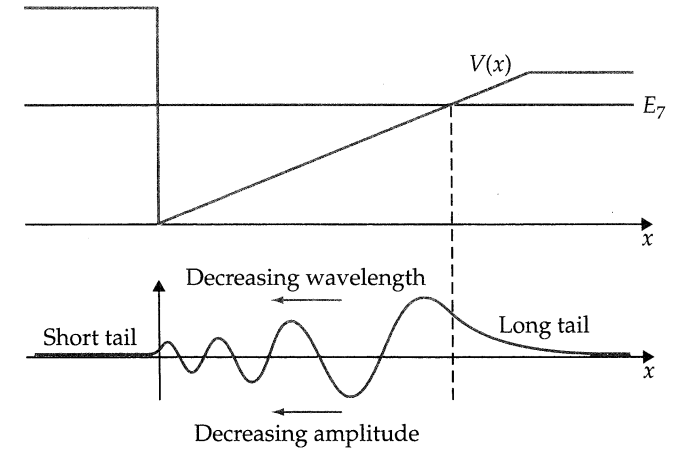
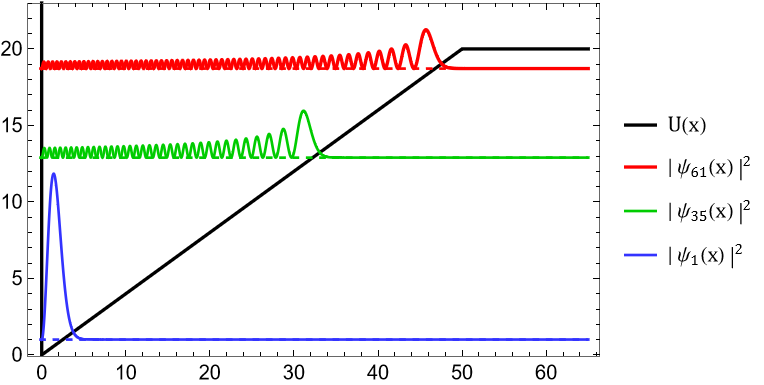
Reading the responses to this question: Contradiction in my understanding of wavefunction in finite potential well it seems people are pretty confident that, e.g., the wavefunction of a particle in a slanted potential well:

makes physical sense, since the system is non-dissipative, so you are more likely to find the particle in a region of higher potential "where its kinetic energy would be lower" in loose terms.

So the probability of finding the particle in some small region near the minimum of the potential is lowest, got it.

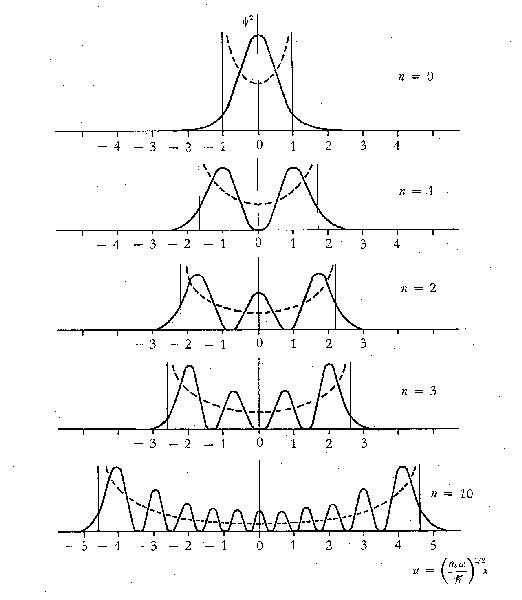
How does this reconcile with e.g. the ground state of the quantum simple harmonic oscillator ($\psi \propto e^{-x^2}$)? In that case we have a situation where the greatest probability of finding the particle is indeed at the minimum of the potential, and so using the idea of classical turning points to determine the maxima of ψ breaks down.

I can't wrap my head around why sometimes the responses to the linked question are fine and dandy, and other times they are manifestly wrong. Is it something to do with my assumption that any state with a given energy would have a higher probability amplitude at higher potential?

Answer 1

Having small or large quantum numbers makes the difference. See also Correspondence principle:

In physics, the correspondence principle states that the behavior of systems described by the theory of quantum mechanics (or by the old quantum theory) reproduces classical physics in the limit of large quantum numbers.

The semi-classical behavior (i.e. the wave function has large amplitude and long wavelength where the classical particle would move slowly) is valid only for large quantum numbers $n$. But usually it is not true for small quantum numbers $n$ (i.e. for the few lowest energy levels). Then the wave function typically behaves very different from a classical particle.

You can see this trend both for the slanted potential well and for the harmonic oscillator.

The particle in the slanted potential well behaves very classical for $n=61$ and $n=35$, but it does not for $n=1$.

The harmonic oscillator behaves quite classical for $n=10$, but it does not for $n=0, 1, 2$.