Applying Gauss' Law to arbitrary conducting surface

Question

In freshman textbooks like Halliday, Resnick and Krane, We derive the electric field on the surface of a conductor by taking a Gaussian surface of infinitesimally small length and infinitesimally small cross-sectional area $dA$, with one face inside the conductor, and then applying Gauss' Law: \begin{align}E=\frac{\sigma}{\epsilon_0}\end{align} My question is that if we do not limit the length of the Gaussian surface, and take it to be a long Gaussian Surface, but of same cross-sectional area $dA$, with one face inside the conductor, then, as the electric field would be constant along the cross section, we would get: \begin{align}E=\frac{\sigma}{\epsilon_0}\end{align} for all locations, which is an incorrect result.

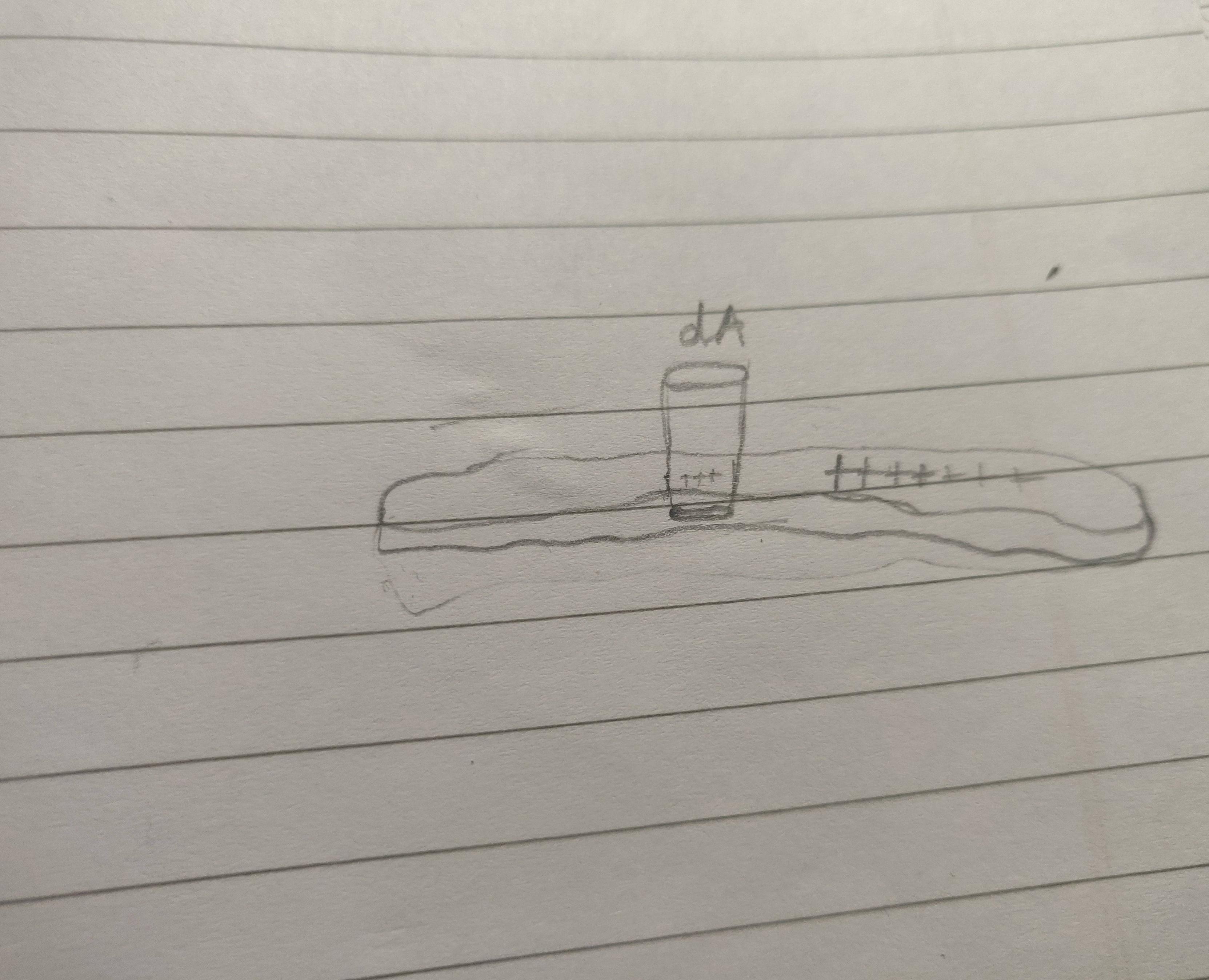
The progress I have made so far is deducing that the contribution to the electric flux from the other parts of the surface of the conductor would be zero in the case of infinitesimal length, as they would be perpendicular to the lateral surface of Gaussian Cylinder, and their resultant would both enter and exit the lateral surface; moreover, as the area of the lateral surface is $dAdl$, the area would be of a smaller order than $dA$ anyway, thus making the flux zero. However, this should also be true for the case of a long cylindrical Gaussian surface. This seems to strengthen the argument that leads to the incorrect result.

Answer 1

If I understand your problem correctly, you're trying to prove what is sometimes called Coulomb's theorem.

If the conducting surface is an infinite plane, stuying its symmetries shows that E is perpendicular to it everywhere in space, so you can take the Gauss cylinder as tall as you want.

Otherwise, E has no reason to be normal to the surface at all, so its flux through the cylinder is hard to compute (often impossible without numerical tools). Hence the bad result if you assume that E is normal to the surface.

However, if you reduce the cylinder to an infinitesimal height, you remain in a small neighborhood of the surface, where the field coming from that point of the surface dominates all the others sources. Then you can locally assume that the field is normal to the surface and get the correct result, but valid only in the immediate vicinity of the surface.