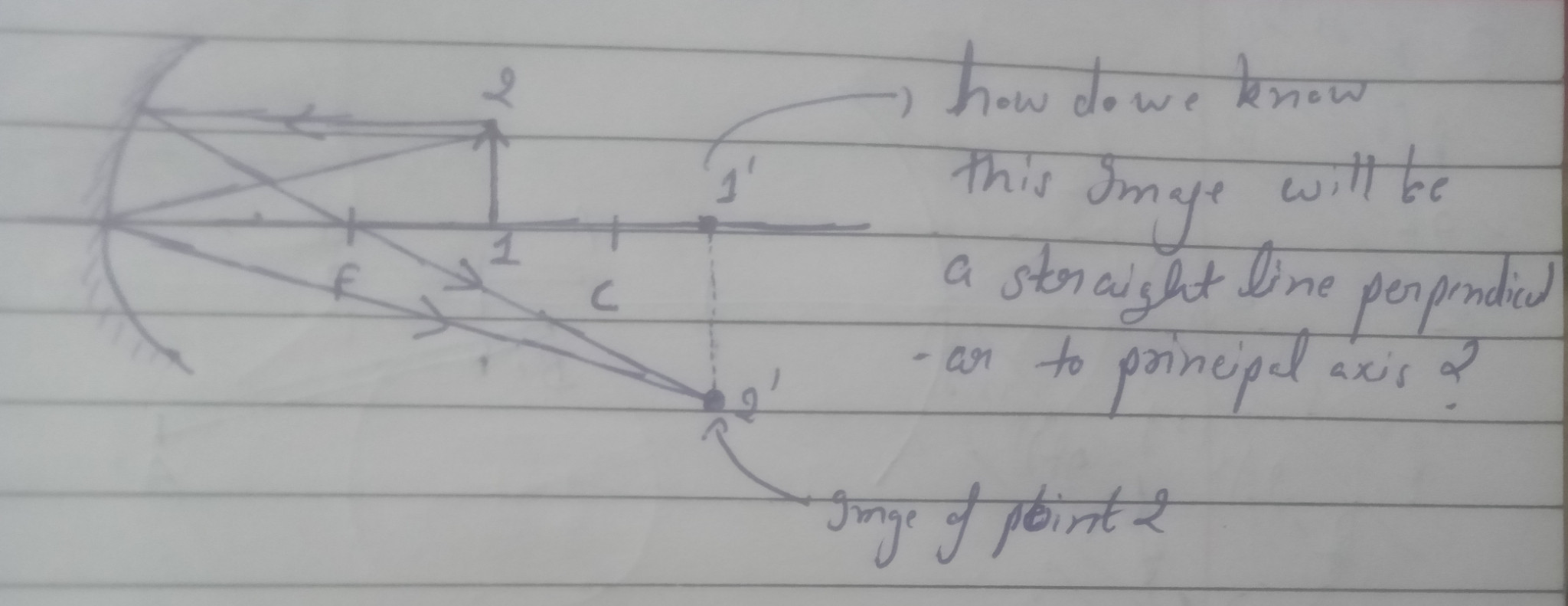
I am a high school student and I am very confused in geometrical optics, In all textbooks the mirror formula is derived by already assuming that the image of any vertical object (perpendicular to the principal axis)formed by a curved mirror will also be a straight line and also perpendicular to the principal axis but why is it true? why the image can't look like what I have shown in the second image? I mean the "x" coordinate of different points of the object can be different, the topmost point can have different "x" coordinate than the bottommost point but in textbooks this thing is not discussed, then how we can say that its gonna be a straight line and also perpendicular to the principal axis? please explain in brief only because I am a high school student.
-
$\begingroup$ Go through the derivation of the Gaussian mirror equation. It makes no assumptions about the shape of the image, and it shows that the image location along the optical axis depends only on the object location along the optical axis and not on the distance from the optical axis. It is worth noting however that this equation relies on the paraxial approximation (all rays are assumed to be almost parallel to the optical axis). In real systems this is not strictly true, and the image of a vertical object won't be exactly vertical due to an optical aberration called curvature of field. $\endgroup$– PukCommented May 15, 2022 at 8:33
-
$\begingroup$ where can i find its derivation? $\endgroup$– Arun BhardwajCommented May 15, 2022 at 13:52
-
$\begingroup$ Any undergraduate textbook on optics will have the derivation. $\endgroup$– PukCommented May 15, 2022 at 17:35
3 Answers
The answer is that all points with the same distance $u$ image onto different points but with the same distance $v$.
You can ask, "ok, but why", and the proof to that is actually not shown in high-school most of the time. I suggest you look for an optics book for undergraduates, where these concepts are often proved explicitly.
It is worth noting also that generally, the statement I made is false for a curved mirror, and there are some assumptions like that the mirror's diameter is much smaller than it's radius of curvature. When these assumptions aren't fulfilled, the image plane doesn't really have an ideal image, and the imperfections are called "aberrations".
-
$\begingroup$ so for now, should I just bare with it ,,,,,however I have made an attempt to solve for this which I want to show here but by posting image of it here ,,I am afraid the my question will get banned. $\endgroup$ Commented May 14, 2022 at 13:51
-
$\begingroup$ posting an image is by itself not a reason to ban a question, only the quality of the question asked (not 'check my soltion' for example) $\endgroup$ Commented May 14, 2022 at 13:58
-
$\begingroup$ but the final result that i got was that it shouldn't be in a straight line ,,,,I did this drawing different principal axis for the topmost point which it perpendicular to its pole and applying the mirror formula to find location of its image on that principal axis,,,I got this result even though I assumed the object should eb very small comapred to the aperture of the mirror so that rays will be paraxial $\endgroup$ Commented May 14, 2022 at 14:00
-
$\begingroup$ farside.ph.utexas.edu/teaching/316/lectures/node137.html try this $\endgroup$ Commented May 14, 2022 at 14:02
-
$\begingroup$ in the link that you have send,,,,they have also assumed that the image of the bottomost point will be in a straight line with the image of topmost point and that line will be perpendicular to the principal axis,,,,my whole concern is that they haven't made ray diagram for the bottomost point to show that its image will form directly underneath the topmost point $\endgroup$ Commented May 14, 2022 at 14:10
You draw it vertical because you are looking at your screen that is vertical (perpendicular to the optics axis).
Edited: it's due to the fact that a lens in the optical limit has a focus $f$ and if your object (now think at a point on the optical axis) is at a distance $p$ from the lens for the lens law: $$ \frac{1}{p} + \frac{1}{q} = \frac{1}{f} $$ you have that the image is at a distance $q$ from the lens.
Remember that in the optical limit:
- your rays are all with little inclination angle, so are almost parallel to the axis;
- your rays go beside the center of the lens, so the curvature of the lens as little (but not null) influence on deflecting rays.
Thanks to these hyphotesis, you have that any point of the object displaced from the optical axis has rays parallel to the point of the object that intersect the optical axis. Parallel rays are, due to these hypothesis, converged to a point at the same distance from the optical axis. I mean, the point of the object which lies on the optical axis will be imaged on the optical axis, but also a point of the object that is $dy$ away from the optical axis will be imaged $dy$ away from the optical axis.
Adding the previous statement to this one has the consequence that every point of a vertical object (so at a distance $p$ from the lens, like the point that lies on the optical axis) that is $dy$ displaced from the optical axis has its image at the same distance from the lens (so at a distance $q$ from the lens, like the point that lies on the optical axis) but displaced of $dy$ from the optical axis. This means that you have a vertical image for a vertical object.
-
$\begingroup$ its not about the screen,,,,according to all the textbooks ,they assume internally that the rays from each part of the object will converge on a vertical line{i.e in one plane} perpendicular to the principal axis ...I am asking for the proof of this statement $\endgroup$ Commented May 14, 2022 at 12:23
-
$\begingroup$ Ok, now I understand your question $\endgroup$ Commented May 14, 2022 at 12:49
-
$\begingroup$ I can also attach the proof of the lens law. $\endgroup$ Commented May 14, 2022 at 12:59
-
$\begingroup$ but its not the proof..... you are using the formula which itself is derived by those assumptions that image is gonna be perpendicular to principal axis and straight line.... $\endgroup$ Commented May 14, 2022 at 13:31
-
$\begingroup$ If you learned how to construct pictures you will find out, but maybe you are more convinced that you get the same picture if you use only part of the mirror. s the left port, das the same as the right part and so on. its not completely true if you use a large spherical mirror, so it is just a good approximation for a real mirror. $\endgroup$– trulaCommented May 14, 2022 at 13:34
So I think what you mean is why is the image assumed to be perpendicular to the surface it is standing on... And it is not slanted or curved as you have demonstrated in your second diagram.
My explanation would be that all the rays are converging at a particular point since it is a real image and therefore tracing the rays of the bottom few points on the object itself in the same way will give you the point at which the bottom converges. Exactly like the way you got the top point by converging two rays.
