0
$\begingroup$

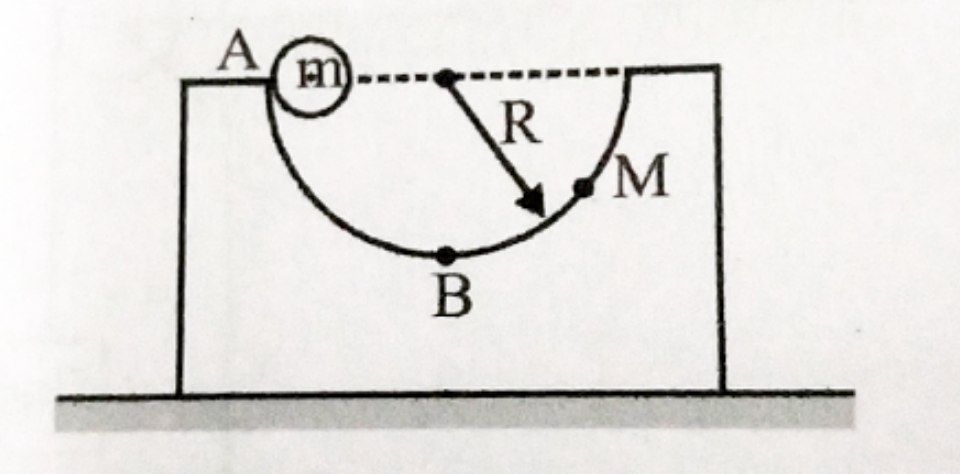
A block of mass $M$ with a semicircular track of radius $R$, rests on a horizontal frictionless surface. A uniform cylinder of radius $r$ and mass $m$ is released from rest at the top point A(see fig). The cylinder slips on the semicircular frictionless track. How fast is the block moving when the cylinder reaches the bottom(point B) of the track?

enter image description here

All the solutions I have seen are using conservation of momentum and mechanical energy. I have no problem in conservation of momentum but in conservation of energy they equate change in kinetic energy of two blocks equal to change in gravitational potential energy of cylinder.
Doubt: I tried it using work energy theorem. Work done by contact force of cylinder and block on cylinder is zero as it is perpendicular to its velocity but work done by that force on block is not zero. So how can I find work done by that force on block? Please guide if I am missing something.

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ "Work done by contact force of cylinder and block on cylinder is zero as it is perpendicular to its velocity". That would be true if the bock is fixed, not if the block can move. $\endgroup$
    – BowlOfRed
    Commented Apr 28, 2022 at 4:01
  • $\begingroup$ The works done on the cylinder by the block and on the block by the cylinder must be equal and opposite. $\endgroup$
    – march
    Commented Apr 28, 2022 at 4:03
  • $\begingroup$ @BowlOfRed I applied it in the frame of block. My mistake. $\endgroup$
    – Shreyansh Kuntal
    Commented Apr 28, 2022 at 4:05
  • $\begingroup$ @march can you please elaborate $\endgroup$
    – Shreyansh Kuntal
    Commented Apr 28, 2022 at 4:05
  • 1
    $\begingroup$ At the point of contact, the component of the displacements along the normal force must be equal, and the forces are equal and opposite, by the third law. The first is harder to justify, but draw a picture of a small displacement, and you should see that this the case. $\endgroup$
    – march
    Commented Apr 28, 2022 at 4:08

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.