What's wrong in my approach towards solving this problem?

Question

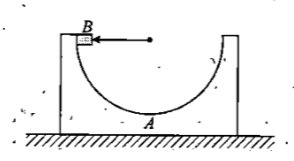
This is the problem that I have to solve:

The figure shows a block $A$ of mass $6m$ having a smooth semicircular grove of radius $a$ placed on a smooth horizontal surface. A block $B$ of mass $m$ is released from a position in grove where its radius is horizontal. Find the speed of the bigger block when the smaller block reaches its bottommost position.

My solution is, let $v_1$ be the speed of the smaller block relative to bigger block when it reaches the bottom-most position and at this instant let $v_2$ be the speed of the bigger block.

Then by conservation of linear momentum in horizontal direction we get $6mv_2 = m(v_1 - v_2)$.

Now by applying work-energy theorem on the smaller block relative to the bigger block, we get

$$ mga = \frac{1}{2}mv_1^2$$ $$ \implies v_1 = \sqrt{2ga}$$

So by subsituting $v_1$ in the linear momentum equation we get $$v_2 = \frac{\sqrt{2ga}}{7} $$

The correct answer given is $v_2 = \sqrt{\frac{ga}{21}} $. I don't know why my approach is wrong. Can anyone point out what is wrong? I think that I didn't include all the work done in the work-energy theorem as the reference frame of the bigger block is non-inertial.

Answer 1

Now by applying work-energy theorem on the smaller block relative to the bigger block

This is the problem. You used the big block as the reference frame, however, the big block itself is accelerated. This complicates the problem. You can't apply work-energy theorem as you did because there would be another fictitious force acting the small block.

The better approach is to use the table as the reference frame. Define $v_1$ and $v_2$ as the horizontal speeds of the small and big blocks respectively with respect to the table. In this case, the energy and momentum conservation consideration gives respectively, \begin{align} \frac{1}{2}m v_1^2 + \frac{1}{2}(6m)v_2^2 &= mga, \\ mv_1 &= 6mv_2, \end{align} where the first equation says that the gravitational potential is transformed to be the kinetic energies of the small and big block. Solving the equations, you will get the correct answer.