Sunscreen protection, SPF, and amount applied: relationship?

Question

In the article How SPF Changes with How Much Sunscreen You Use (last updated Dec 29, 2017), the author says that insufficient sunscreen disturbingly decreases protection exponentially, in other words, that sunscreen application has increasing marginal utility:

The amount used in the sunscreen studies to determine SPF is $2$ milligrams of sunscreen per centimetre of skin.

On average, people apply only a quarter to a half of the recommended amount, and in most studies, it’s closer to $0.5$ mg/cm².

In physics, you’d expect protection to decrease exponentially with less sunscreen. This is based on a relationship called the Beer-Lambert law, and is illustrated as

it’s the middle of the graph that’s disturbing.

Applying half the recommended amount of sunscreen cannot give more than SPF $\mathbf{10}$, even with SPF $50$ sunscreen!

by $0.5$ mg/cm², we’re below SPF $3$ for all products.

Now, intuitively, the initial layer of sunscreen makes a greater difference than any subsequent layer, that is, each layer of the recommended amount of sunscreen is less beneficial than the previous layer; does the theory really contradict this intuition?

Answer 1

1. A sunscreen's sun protection factor (SPF) is defined as $$\frac{\text{minimal erythemal dose in sunscreen-protected skin}}{\text{minimal erythemal dose in non-sunscreen-protected skin}},$$ so its transmittance (transmitted percentage of the incident erythemal radiation) is $$T=\frac1{\text{SPF}}\times100\%.\tag1$$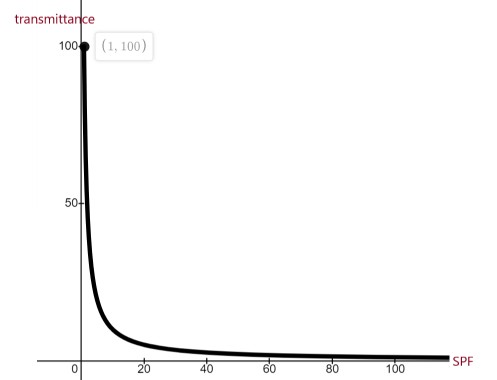

   So, the sunscreen's percentage protection (filtered-out percentage of the incident erythemal radiation) is $$\left(1-\frac1{\text{SPF}}\right)\times100\%\tag2.$$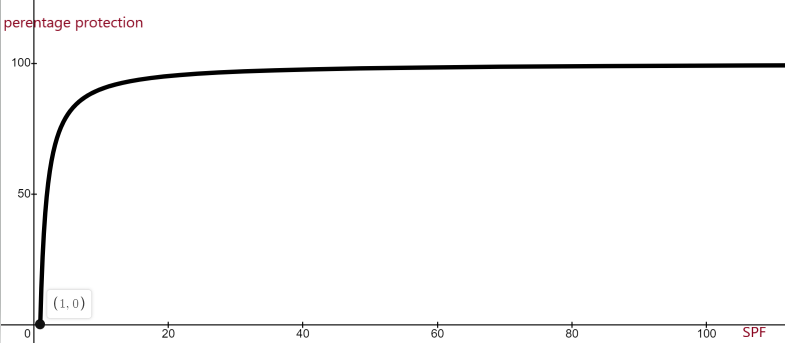

2. Let $s$ be a sunscreen's intended SPF, $x$ be the applied fraction of its prescribed amount, and $f(s,x)$ be its effective SPF.

   Beer's Law predicts that the sunscreen's absorbance $\left(\log_{10}\dfrac1{T(s,x)}\right)$ is directly proportional to $x.$ So, $T(s,x)=e^{-k_sx}$ for some positive $k_s,$ so the sunscreen's transmittance decreases exponentially with $x.$ So, by formula $(1)$, $\large f(s,x)=e^{k_sx},$ so $f$ increases exponentially with $x.$ Thus, since $f(s,1)=s,$ the sunscreen's effective SPF is $$f(s,x)=s^x.\tag3$$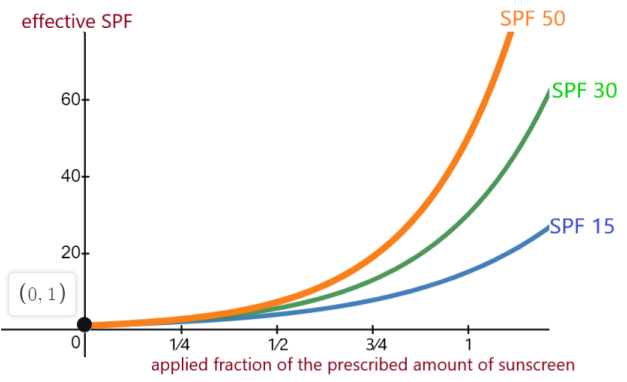

   So, by formula $(2)$, the sunscreen's percentage protection (filtered-out percentage of the incident erythemal radiation) is $$\left(1-\frac1{s^x}\right)\times100\%.\tag4$$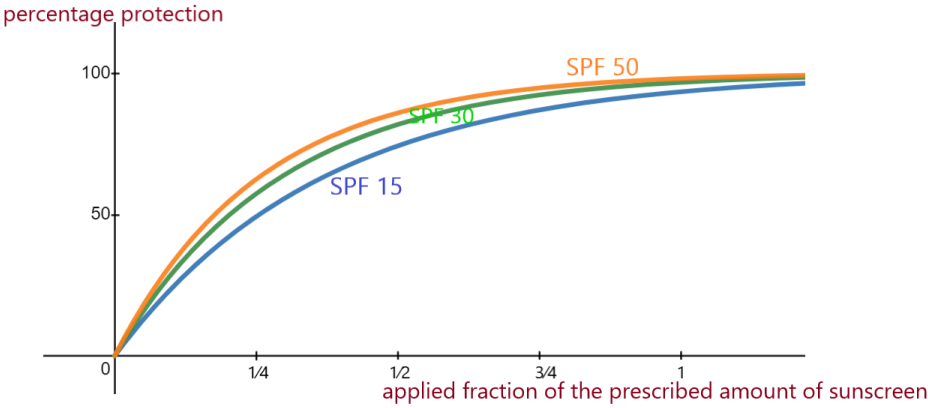

3. In physics, you’d expect protection to decrease exponentially with less sunscreen. This is based on a relationship called the Beer-Lambert law

   From formula $(3),$ Beer's Law predicts that effective SPF—not protection—decreases exponentially with less sunscreen.

   From formulae $(4),$ Beer's Law in fact predicts that percentage protection increases with more sunscreen at a decreasing rate, rather than exponentially.

   To be clear: effective SPF and percentage protection both increase with more sunscreen, but in different ways. The cited article is carelessly conflating these two concepts.

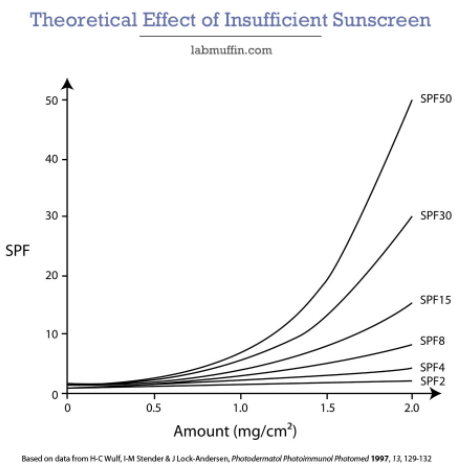
   According to this theoretical relationship, reducing the applied amount by one-quarter will already halve your sun protection if you’re using SPF 30 or above.

   Applying half the recommended amount of sunscreen (1.0 mg/cm²) cannot give more than SPF 10

   applying half the amount of sunscreen would give you less than half the protection.

   To be clear: from formula $(4),$ applying half the prescribed amount of sunscreen actually confers more than half the intended amount of protection, that is, it filters out more than half of what it would have with the full amount of sunscreen.

   For example, half the prescribed amount of an SPF $50$ (i.e., $98\%$ percentage protection) sunscreen effectively attains SPF $7$ (i.e., $86\%$ percentage protection); this is an efficacy decrease of only $12\%.$

   (Similarly, reducing the SPF from $50$ to $25$ decreases the efficacy by just $2\%,$ whereas reducing the SPF from $50$ to $2$ decreases the efficacy by just $50\%;$ see graph $(2).)$

4. it seems like most sunscreens actually give a linear relationship, where half the sunscreen would actually give you around half the protection, like in the purple line below.

   One study even found that there was a logarithmic relationship like in the blue line on the graph, where half the sunscreen would actually give you more than half of the full protection!

   

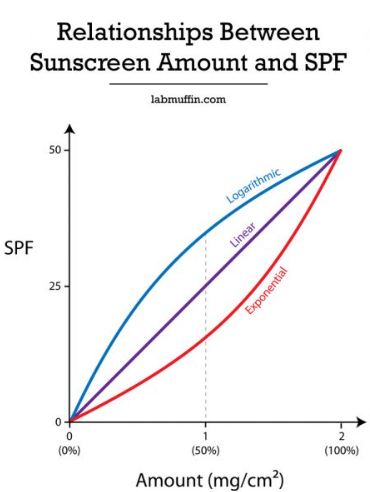
   In all of the studies, the protection achieved was much better than the amounts in the theoretical prediction.

   The proposed reasoning for why the Beer-Lambert law fails is because it assumes an even surface, when skin is actually uneven.

   The red curve ought to be translated upwards by one unit so that its vertical intercept becomes SPF $1;$ this is essentially graph $(3).$

   The purple curve (also to be translated upwards by one unit) gives a better prognosis than the red curve, which, as explained above, already says that half the sunscreen confers over half the protection.

   The purple curve does not say that the amount of radiation filtered out is proportional to the amount of sunscreen applied. (Remember, SPF is neither proportional to nor even linearly related to percentage protection; similarly, by formula $(1),$ SPF is inversely proportional to instead of linearly decreasing with transmittance.) In fact, for SPF $50,$ here are the corresponding quantities for the purple model:

   

   The blue curve (also to be translated upwards by one unit) gives the most concave version of graph $(4).$ N.B. The blue curve—whether corrected or not—is not logarithmic and, incidentally, neither is graph $(4).$

   To be clear: all three models, even the purple and red (the theoretical prediction), indicate that percentage protection suffers diminishing returns as sunscreen amount increases. Beer's Law has not actually failed. The blue model, which is the most optimistic relative to the theoretical prediction, in fact appears to be the least well-supported by studies.

5. The amount used in the sunscreen studies to determine SPF is $2$ milligrams of sunscreen per centimetre of skin. On average, people apply only a quarter to a half of the recommended amount

   Therefore, it would be a boon to public health to replace SPF on sunscreens with a measure that is more realistic (about the typical amount of sunscreen applied) and also more intuitive: say, sun protection index B, defined as $$\text{SPI}_B=100\left(1-\frac1{\sqrt{\text{SPF}}}\right).$$ This index, which is basically formula $(4)$ with $x=\dfrac12,$ varies between $0$ and $100,$ proportionally to the amount of erythemal radiation that the sunscreen filters out when the user applies $1$ mg/cm² instead of the unrealistic $2$ mg/cm².

   Note that, by definition (‘erythemal dose’), both SPF and SPI$_B$ are heavily biased towards measuring UVB screening.

   So, just as PPD (persistent pigment darkening) is the UVA counterpart of SPF, we might analogously define sun protection index A (SPI$_A)$ as SPI$_B$'s counterpart for measuring UVA screening (more accurately, for measuring the screening of pigment-darkening radiation): $$\text{SPI}_A=100\left(1-\frac1{\sqrt{\text{PPD}}}\right).$$ This index—analogously to the above index—specifies the percentage of the pigment-darkening radiation that the sunscreen filters out when the user applies $1$ mg/cm² instead of the unrealistic $2$ mg/cm².

   As an illustration, the $$\text{PPD } 46,\:\:\text{SPF } 50+$$ rating of this sunscreen is equivalent to $$\text{SPI}_A\:85,\:\:\text{SPI}_B\:86+.$$ NB: many countries forbid sunscreens from being labelled with an SPF above $50,$ due to the prevalent temptation to interpret SPF proportionally to level of protection; since my proposed ratings SPI$_A$ and SPI$_B$ are to be read precisely this way, the “SPF $50+$” above will in fact actually revert to the more precise/informative “SPI$_B$ $91$” (say) instead of being converted to “SPI$_B$ $86+$”.