1
$\begingroup$

I am working on an exercise which is asking to find the elements of the symmetry group of the following figure given below: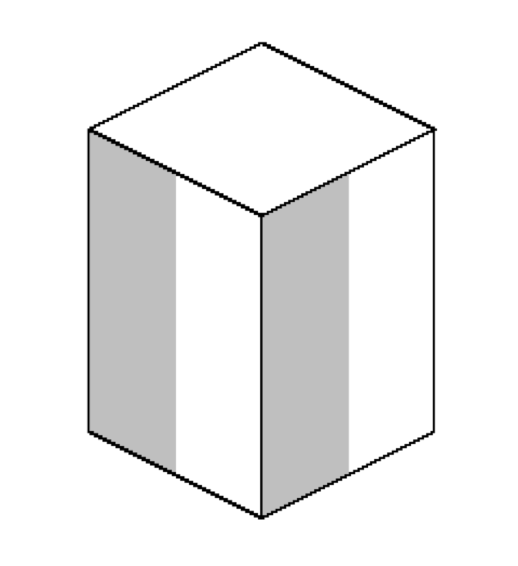Rectangular box with a square base

Note that the rectangular sides of the box all have the exact same pattern where the left half is grey and the right half is white.

If I consider $c$ to be the rotation of the figure around the z-axis (axis being perpendicular to the square base and top of the rectangular box) and $b$ to be the reflection on the $x-y$ plane (plane formed by the $x$ and $y$ axis which are both perpendicular to the vertical rectangular sides), I have figured out that $$c^4=e, b^2=e, (bc^2)^2=e$$.

I thought the element of my group would be $${e,c,c^2,c^3,b,bc,bc^2,bc^3}$$ which is the group $D_4$, but knowing that $bc^2bc^2=e$ I can only find $cb=c^3bc^2$ and I cannot find an expression to commute $c$ and $b$. That mean that I am missing elements on the group.

What elements would form my group and which group would it be?

Improve this question
$\endgroup$
10
  • $\begingroup$ Seems more like a question for Math.SE $\endgroup$
    – Nihar Karve
    Commented Apr 3, 2022 at 15:20
  • $\begingroup$ Just working out an example, it seems to me that $c b = b c^3$. In other words, looking at the square from the top, a reflection followed by a clockwise rotation, is equivalent to three clockwise rotations (or one counter clockwise rotation) followed by a reflection. $\endgroup$
    – Andrew
    Commented Apr 3, 2022 at 15:22
  • $\begingroup$ I think you are refering to a reflection on a different plane from mine. If I do the operations you mentioned on my definition of reflection (see above I reflect over the $x-y$ plane), I do not get that the operations you said are equal. @Andrew $\endgroup$
    – god_operator
    Commented Apr 3, 2022 at 15:30
  • $\begingroup$ sorry but i do not have enough score to post an image on Math SE @NiharKarve $\endgroup$
    – god_operator
    Commented Apr 3, 2022 at 15:30
  • 1
    $\begingroup$ The reflection and rotation don't commute if the reflection is through the $xz$ or $yz$ plane. That is the case where $cb=bc^3$. The reflection and rotation do commute if the reflection is through the $xy$ plane. This is not difficult to prove. Put labels "a, b, c, d" on the corners of the top plane of the box, and "A, B, C, D" on the corners of the bottom plane of the box, and try doing a few examples. $\endgroup$
    – Andrew
    Commented Apr 3, 2022 at 16:56

0

Reset to default