Velocity gradient in a liquid

Question

When we consider the motion of fluid in terms of many thin layers sliding over each other , we say that layer at a top of a layer forces it to move forward while layer below a layer forces it to move backwards by applying a resistive force $f_v$ where $$f_v=\eta A \frac{\text{d}v}{\text{d}h}$$

If velocity gradient is constant then each layer is pushed by a force $f_v$ forward and by an equal force $f_v$ backwards implying net force on a layer is zero ( with some external force on top layer to keep it moving with constant velocity) and hence every layer moves with constant velocity.

Since each layer has net force zero on it and it moves with constant velocity then why there is a decrease in velocity of layers as we move deeper in the liquid, is this due to some interaction other than viscous forces?

P.S. - I am a high school student who has learned only a basic definition of viscosity.

Answer 1

I think you are unaware of the no-slip boundary condition. In simple words, as you're a high school student,

When a stream of fluid flows over a horizontal surface, the layer of fluid just above the surface comes to rest due to friction which resists the motion of the fluid layer. This is known as the no-slip boundary condition as there is no relative motion between the boundary and the fluid layer right next to the boundary. This layer at rest then applies resistive viscous forces on the top consecutive layers which cause a positive velocity gradient in the upward direction. This force between consecutive fluid layers has the formula that you've correctly mentioned and is the viscous force.

For many general cases, the no-slip boundary condition is valid and even if it's not in some cases, you still know that the external force that initiates the velocity gradient is the friction due to the boundary.

So, you must think just the opposite of what you've written in the question i.e. why does the velocity increase as we go upwards?

Edit: Imagine that there is a stream of fluid with each layer having a constant velocity. Suddenly the flowing fluid encounters a surface at the bottom. The lowermost layer of the fluid will immediately come to rest as it flows over the surface. This layer will pull the layer just above with the force $f_v$ where $$f_v=\eta A \frac{\text{d}v}{\text{d}h}$$. This will cause the above layer to decrease in speed and hence the effect of friction of the surface propagates upwards causing the speed of the above layers to reduce. This is what causes the velocity gradient.

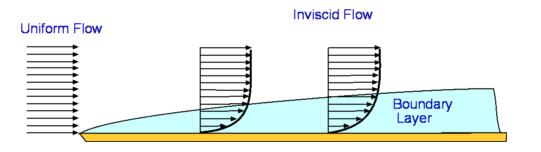
The arrows denote magnitude of velocity at that point.

See how on the left, each layer has same velocity. But now that it encounters a boundary, velocity becomes zero at surface and increases upwards. At each point between two layers, the viscous force would be related to velocity gradient at that point.

Answer 2

Here is one way to look at this. I think your problem is you are forgetting that this setup has two boundary constraints: 1) the velocity at the top of the "stack" of thin fluid layers is moving with a specific free-stream velocity, and 2) the layer closest to the wall at the bottom of the stack is moving with velocity zero. Try the derivation again including the v = 0 constraint at the wall and see what develops.

Answer 3

It has to do with how momentum is transferred between the fluid layers. The fluid layers close to the wall feel more resistance than the top ones. Imagine collisions between two bodies at a high velocity difference and at a low velocity difference. The fluid layer very close to the wall has zero velocity, so the next layer to that would feel the maximum resistance force compared to the above layers.