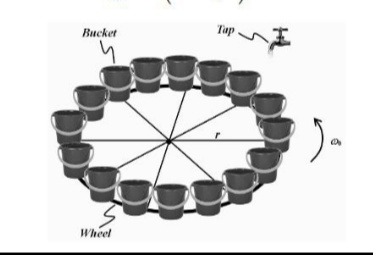
A horizontal wheel with light buckets fixed on its circumference revolves about a frictionless and vertical axis. Water falling from a fixed tap with a uniform rate of mass $m$ per unit of time is collected by the buckets. Denote $I$ and $r$ as the initial moment of inertia of the entire system about the axis and the radius of the wheel respectively. Is it necessary that the mass rate at which water falls on the buckets will be constant over time'?
As the question says its what happening so how can one do that?
My reasoning is like as the angular velocity of water is changing with time so the rotation speed is changing so it would take less/more time compared to initially for water to fill $m$ mass isnt ? And am i right in my interpretation of what will happen during the process: the water will have some vertical speed which will reduced to zero as soon as it meets any bucket but it will instantly gain the speed of that bucket at that instant so its getting a force impulsive from the walls of the container which is varying with time?