If two unlike forces act on two different points of a rigid body, won't the body just start to rotate?

Question

Question:

Two unlike parallel forces $P$ and $Q$ $(P>Q)$ act at $A$ and $B$ respectively. If P and Q are both increased by R, show that the resultant will move through a distance $d=\frac{R}{P-Q}$.

My book's attempt:

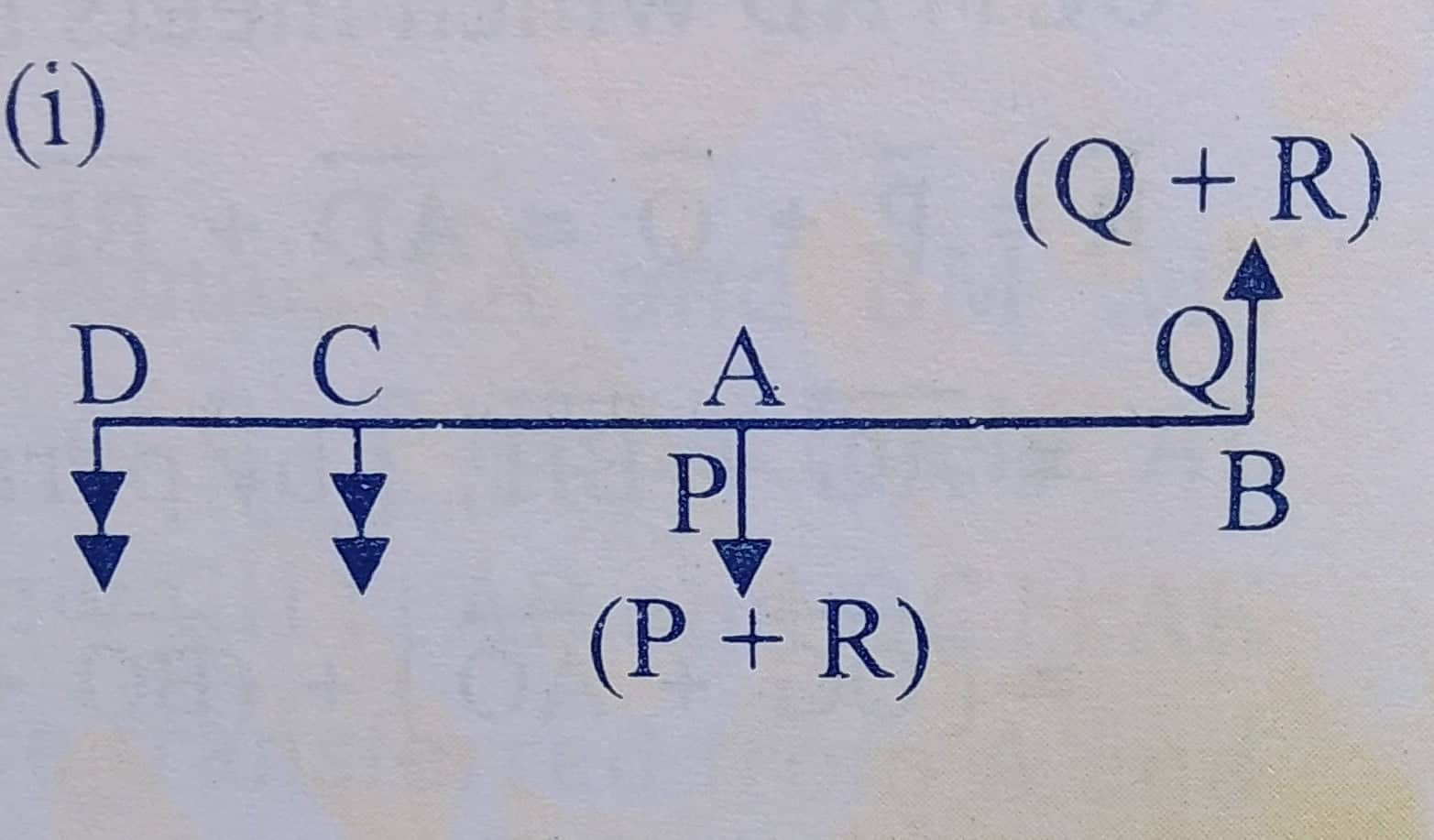
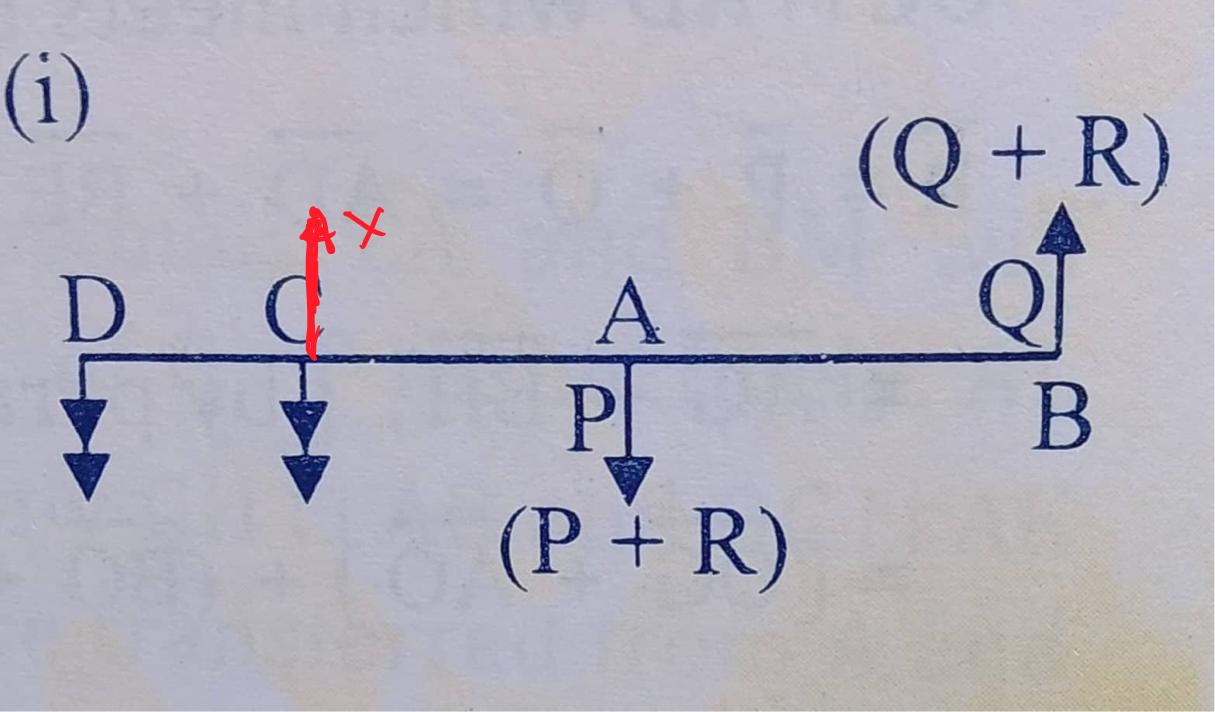
Let the resultant of two unlike parallel forces $P$ and $Q$ act at $C$.

$$\frac{P}{BC}=\frac{Q}{AC}=\frac{P-Q}{AB}$$

[Each force is proportional to the distance between the points of application of the other two.]

From 2nd and 3rd ratios,

$$AC=\frac{Q}{P-Q}AB\tag{1}$$

Let when $P$ and $Q$ are increased to $P+R$ and $Q+R$, their resultant act at $D$, where $CD=d$.

$$\frac{P+R}{BD}=\frac{Q+R}{AD}=\frac{P+R-Q-R}{AB}$$

[Each force is proportional to the distance between the points of application of the other two.]

From the 2nd and 3rd ratios,

$$AD=\frac{Q+R}{P-Q}AB\tag{2}$$

$(2)-(1)$,

$$AD-AC=\frac{Q+R-Q}{P-Q}AB$$

$$d=\frac{R}{P-Q}AB\ (\text{showed})$$

My comments:

My book assumed that the forces $P$ and $Q$ acting at $A$ and $B$ respectively will have a resultant force $P-Q$ that will act at $C$. I take issue with this. I would've agreed with the book if P and Q were both acting at the same point; then the resultant would've been $P-Q$, which would've acted at the same point as $P$ and $Q$. However, that's not the case here. P and Q are acting at two different points in opposite directions. I think what will happen is that the body that the two forces are acting on will experience a net torque and start rotating.

My question:

1. Isn't my book's attempt wrong?

Answer 1

The center of mass of the board will accelerate given a non-zero net force, and the board will rotate about the center of mass given a non-zero net torque, but the problem asks to find the effective movement of the point of application of the "resultant". For this problem, the "resultant" is a simply a single force that would cause the same motion as the set of actual forces.

Sometimes a single effective force is insufficient to model the problem; for example, equal forces that cause rotation, and a "couple" can be used in this case. A couple is a system of forces whose vector sum is zero.

It can be shown that every system of forces is equivalent to a single force through an arbitrary point plus a couple (either or both of which may be zero). [Symon, Classical Mechanics]

Resolving a system of forces into single force and a couple is common in engineering mechanics textbooks, but not so much in physics mechanics textbooks.

The problem provides insufficient information to evaluate the motion. To evaluate the motion (translation and rotation) you need the forces (magnitude and points of application), the mass and length of the board, and the density of the board as a function of length. Then you can calculate the motion of the center of mass from the net force and the rotation about the center of mass from the net torque.

Answer 2

Without doing any calculation, I see things that make me think your book is right.

First, here is a post that shows how the think about this kind of problem. Toppling of a cylinder on a block

First P and Q can be resolved into two separate things: A force with magnitude $(P-Q)$ that accelerates the stick downward. And a pair of equal and opposite forces of magnitude $Q$ that rotate the stick.

The book says that a single resultant force acting at C would do the same thing. A force at C of magnitude $(P-Q)$ would pull the stick downward just like P and Q do. A force to the left of the center would rotate the stick counterclockwise just like P and Q do.

If you add the same force R to P and Q, you can repeat this analysis. You should find the resultant pulls the stick downward just as hard. But the bigger torque rotates the stick more. The resultant should be the same magnitude, but move to the left.

Answer 3

Imaging pushing up at one end and down at the opposite end of a rod like this. With no other forces acting. Will the rod rotate or move or both?

We can know this by using Newton's 2nd law in its translational version and also in its rotational version. For the translational version, the forces don't have to be pushing at the same point - for a rigid object, a force exerted at one point will propagate through the entire body. The law then only deals with the net force on the object as a whole.

By applying these two laws, we will find from the translational version that the net force is zero whereas the rotational version shows a non-zero net torque. So, the rod will not move but it will rotate.

Now to your scenario. We could ask the same question: Will the rod move or rotate or both? It will turn out that it will do both. But your question only asks for the displacement, so the simultaneous rotation is just ignored.

Answer 4

you have two equations

sum of the torque about point A $$\left( P-Q \right) {\it AC}=Q{\it AB}$$ sum of the torque about point D $$\left( P+R \right) {\it AD}= \left( Q+R \right) \left( {\it AB}+{ \it AD} \right) $$

from here you obtain the distance $~AC~,AD~$

$$AC={\frac {Q{\it AB}}{P-Q}}\\ AD={\frac { \left( Q+R \right) {\it AB}}{P-Q}}$$

your question

for static equilibrium you obtain

$$ P-Q=0\\ P\,AC-Q\,BC=0$$ but if $~P\ne Q~$ you still have two equations

sum of the forces $$P-Q-X=0$$ and sum of the torque about point A

$$X\,AC-Q\,AB=0$$

form here you obtain

$$X=P-Q\\ AC={\frac {Q{\it AB}}{P-Q}}$$

Analog put the unknown force X at point D again you have two equations with the unknowns X and AD