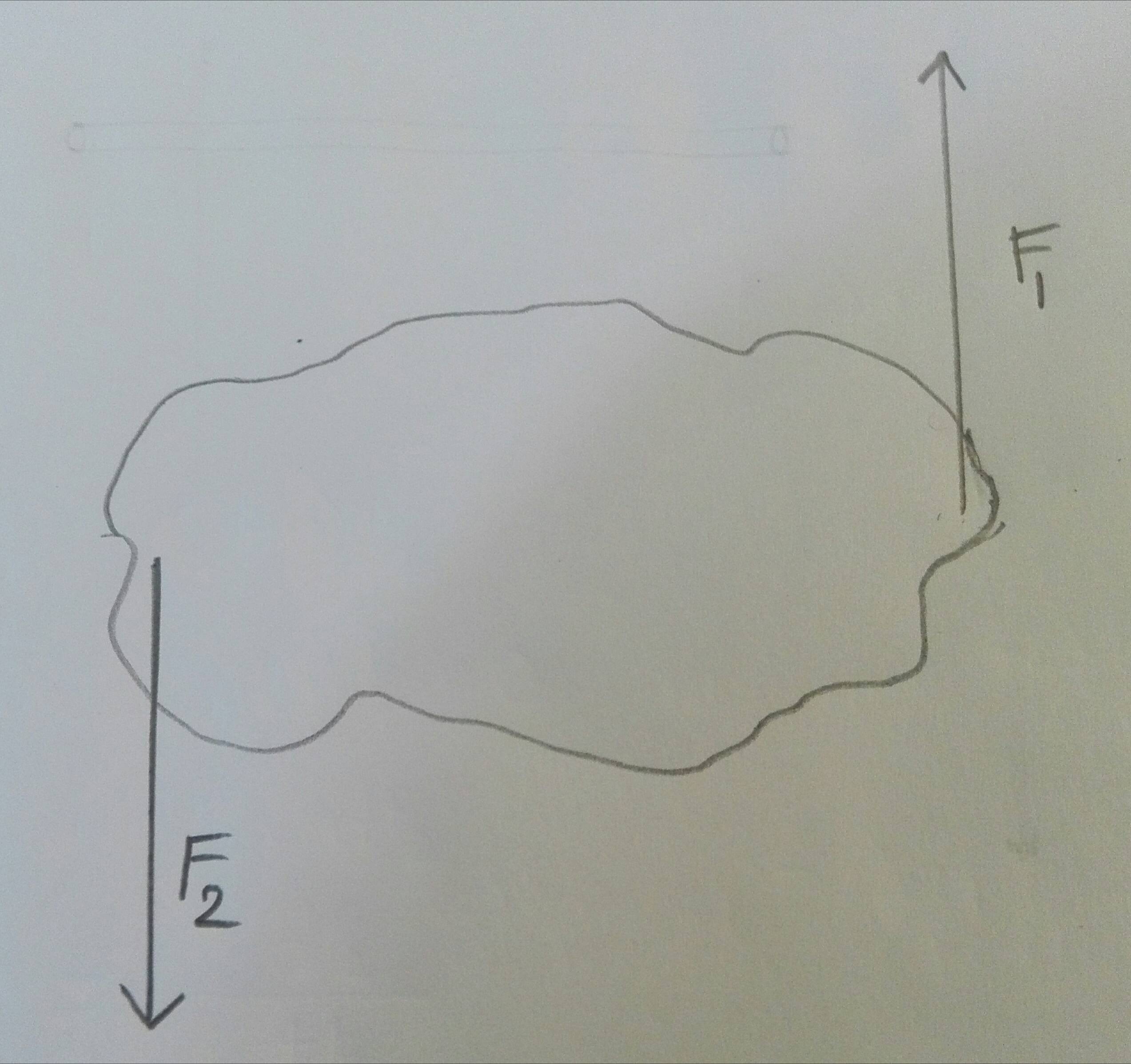
What will be the effect on a rigid body if two unlike unequal parallel forces act on the body?

Question

What will be the effect on a rigid body if two unlike unequal parallel forces act on the body? Will the body translate or rotate or both? If they rotate, with respect too which point will they rotate?

Answer 1

Answer: both.

Now, to determine the point about which they rotate, calculate the linear acceleration (of center of mass) and the angular acceleration (using the torque equation about the center of mass). Then, the acceleration of any point on the body would be the vector sum of linear acceleration and distance times the angular acceleration. The required point is the one for which this vector sum vanishes.

Answer 2

The same laws of motion apply here too. Get the net force to see how the center of mass moves and the net torque about the center of mass to see how the body rotates.

So unless the net torque is zero about the center of mass, the body is going to translate and rotate at the same time.

Consider the general case of two unequal opposite forces $F_1$ and $F_2$ each at a distance $d_1$ and $d_2$ from the center of mass at opposite sides. The force act along the y axis and we track the vertical acceleration of the center of mass $\ddot{y}$, as well as the rotational acceleration $\ddot{\theta}$. Finally we find the center of rotation along the x-axis relative to the center of mass as $ r= - \frac{\ddot{y}}{\ddot{\theta}} $.

1. Linear Motion

$$ (F_1) + (-F_2) = m \ddot{y} \;\Rightarrow\; \ddot{y} = \frac{F_1-F_2}{m} $$

2. Rotational Motion

$$ (d_1) (F_1) + (-d_2) (-F_2) = I_C \ddot{\theta} \; \Rightarrow \; \ddot{\theta} = \frac{d_1 F_1 + d_2 F_2}{I_C} $$

_{Where $m$ is the mass and $I_C = m \kappa^2$ is the mass moment of inertia about the center of mass, and $\kappa$ the radius of gyration.}

3. Center of Rotation

$$ r = - \frac{\ddot{y}}{\ddot{\theta}} = -\frac{ (F_1-F_2) I_C }{ (d_1 F_1 + d_2 F_2) m} = -\frac{(F_1-F_2) \kappa^2}{d_1 F_1 + d_2 F_2} $$

Now lets consider the fringe cases:

1. Rotation about the center of mass, $r=0$. This happens only when the two forces are equal in magnitude $F_1 = F_2$.

2. Pure translation, $r = \infty$. This happens only when the net torque about the center of mass is zero $d_1 F_1 + d_2 F_2 =0$.

In any other case you have simultaneous translation and rotation about the center of rotation.

---

There is a shortcut to finding the center of rotation. Consider $d$ the distance where the combined forces act through. Essentially $\sum M = d \sum F$, or $$ d = \frac{d_1 F_1+d_2 F_2}{F_1-F_2} $$

Now consider the mass moment of inertia $I_C$, and the radius of gyration $\kappa$. They are related by $I_C = m \kappa^2$.

The center of rotation is found by

$$ r = -\frac{I_C}{m d} = -\frac{\kappa^2}{d} $$

The negative sign here means that $r$ is located on the opposite side of $d$. This means that the center of rotation is purely a geometric quantity as only relates to distances and the radius of gyration. All of these quantities are a function of the shape of the body only.

Stated backwards, if the center of mass is a distance $r$ from the pivot point (center of rotation) then the axis of percussion (axis where momentum needs to be applied to stop the motion) is at a distance $ d = \frac{\kappa^2}{r} $ from the center of mass in the opposite side.

That is why a baseball bat (approx. cylinder of length $\ell$ with center of mass at $r=\frac{\ell}{2}$) has its axis of percussion (sweet spot) at a distance $d$ from the handle of

$$ d = r + \frac{I_C}{m\,r} = \frac{\ell}{2} + \frac{\ell}{6} = \frac{2 \ell}{3} $$

Answer 3

This answer applies equally to your related question in which the two forces are parallel and unequal but act in the same direction.

The pair of forces can be replaced by a resultant force $F$ and a torque $T$. The resultant motion is a combination of :

1. acceleration of the centre of mass in a straight line, according to $F=ma$, where $m$ is mass and $a$ is linear acceleration; and
2. rotation about the centre of mass according to $T=I\alpha$ where $I$ is moment of inertia about the CM and $\alpha$ is angular acceleration.

In the anti-parallel case shown here, in which the forces point in opposite directions, the resultant force and anti-clockwise torque are given by $$F=F_1-F_2$$ $$T=F_1 x_1 + F_2 x_2$$ assuming $F_1 \ge F_2$. For the parallel case replace $F_2$ by $-F_2$.