0
$\begingroup$

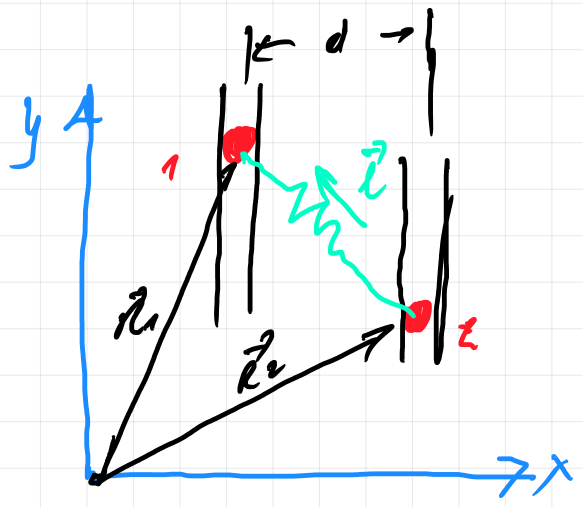
Imagine two masses connected with a spring of elastic constant $k$ set up such that they can move freely in the vertical direction but are connected horizontally. They also cannot move in the horizontal direction, such that they are always at a (horizontal) distance $d$. There's $N=2$ degrees of freedom.

Obviously the spring is going to exert some force between the masses if you disturb them from their original position. But how do you determine this force, what would its potential be? It obviously can't be $F=-kx$ since the spring can't stretch in this direction. Are we to assume that these kinds of ideal spring simply do not exert force in this way and can stretch vertically infinitely?

Improve this question
$\endgroup$
3
  • $\begingroup$ $x$ doesn’t refer to any particular Cartesian axis but to the deflection (which you might calculate with trigonometry in this case). $F=-kx$ still holds, where $F$ and $x$ are now vectors. Does this answer your question? If not, please consider adding a diagram for clarity. $\endgroup$
    – Chemomechanics
    Commented Mar 2, 2022 at 23:35
  • $\begingroup$ @Chemomechanics Alright, so Hooke's law just takes into account the "stretching" of the spring regardless of the coordinates, and therefore you could just calculate this using trigonometry, right? $\endgroup$
    – agaminon
    Commented Mar 2, 2022 at 23:40
  • $\begingroup$ That’s right. You can use the diagonal distance between the spring connection points as the new length. $\endgroup$
    – Chemomechanics
    Commented Mar 2, 2022 at 23:58

1 Answer 1

Reset to default
0
$\begingroup$

enter image description here

starting with the position vectors to the masses

$$\mathbf R_1=\begin{bmatrix} x_1 \\ y_1 \\ \end{bmatrix}\quad , \mathbf R_2=\begin{bmatrix} x_2 \\ y_2 \\ \end{bmatrix}$$

and the constraint equation

$$x_2-x_1=d$$

the spring force is:

$$\mathbf F=-k\,(\parallel\mathbf l\parallel-l_0 )\,\mathbf{e}_s$$

where $$\mathbf l=\mathbf R_2-\mathbf R_1\\ \mathbf e_s=\mathbf{\hat{l}}$$

and $~l_0~$ is the spring preload length

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.