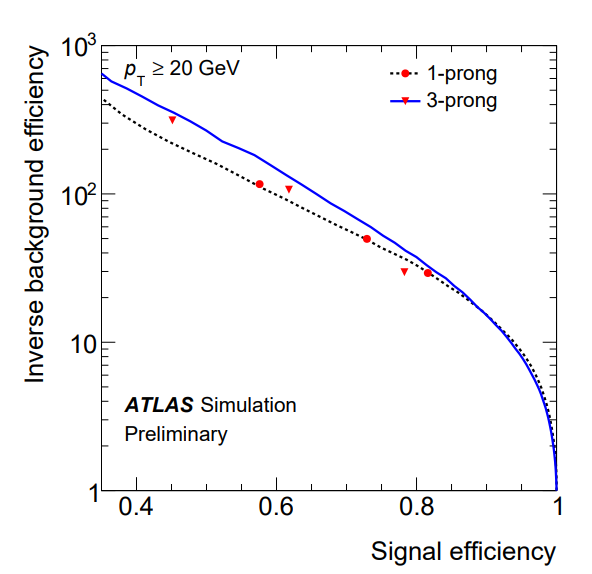
"Inverse [background] efficiency" (also known as "[background] rejection") is calculated as the reciprocal of [background] efficiency.
In more detail (using $\tau$ identification as example), let's say you have a collection of objects, a portion of which are true taus and the remaining portion of which are not taus. Apply your $\tau$ identification procedure; then the "signal efficiency" would be calculated as
\begin{equation}
\varepsilon_\tau = \frac{\text{number of true $\tau$ identified as a $\tau$}}{\text{total number of true $\tau$}}
\end{equation}
However, there will also be some fraction of the not-taus in the collection of objects that could look like a tau, and thus be identified as a tau. So similarly there will be a "background efficiency"
\begin{equation}
\varepsilon_b = \frac{\text{number of not-$\tau$ identified as a $\tau$}}{\text{total number of not-$\tau$}}
\end{equation}
and from this, the rejection value is calculated as $1/\varepsilon_b$.