1
$\begingroup$

For a very long time I never questioned the idea that sunrays hitting Earth are to all practical purposes 'approximately parallel'.

This is confirmed by posts like this one and this one.

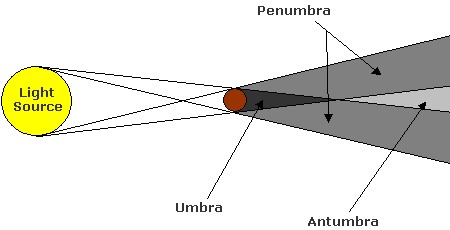
However, if this were true, I would also expect an object hit by sunlight to cast a sharply defined shadow, not a shadow like the one we get from a light bulb, where there is a darker central area and a sort of hazy contour all around.
But I thought: surely the angle formed by the rays that come from the 'top' and 'bottom' of the Sun with a point on an object is so minute that it does not matter.

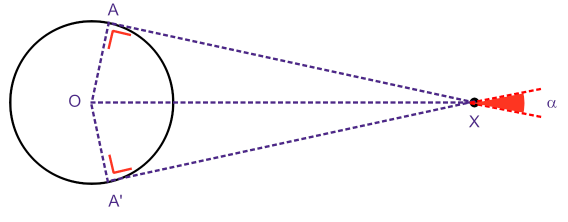
So I went and checked what that angle was:

enter image description here

If the Sun is the sphere centered in $O$, and the point on Earth that is hit by its rays is $X$ (obviously not at the correct scale in this picture), according to my calculations:

$\alpha = 2 \cdot \arcsin \frac {OA}{OX}$

Given that:

$OA \approx 7 \cdot 10^5 \ km$
$OX \approx 1.5 \cdot 10^8 \ km$

$\alpha = 2 \cdot \arcsin \frac {7 \cdot 10^5}{1.5 \cdot 10^8} \approx 2 \cdot 0.0047 \ rad \approx 0.53 \ deg$

So OK, the angle is pretty small.
But still one might be able to detect some haze around the contour of the shadow, or am I wrong?
Is there any phenomenon that can be observed on Earth that shows that? Like hinted to here maybe?

The other thing that occurred to me when I looked at this was that OK, we have an angle $\alpha$ coming 'out of the back' of the point, but then how spread out the projection is (i.e. how observable it is) depends on the distance between the point and the surface on which the shadow is projected.
As an example, I am sitting now in a room lit by a light bulb of about 7 cm in diameter, located 4 m away from me, so the ratio $\frac {OA}{OX}$ is only about twice the one calculated for the Sun, and so is $\alpha$.
However, if I move my hand and look at the shadow it casts on the table next to me, I can clearly see how the hazy area increases as the hand is farther away from the table.
Am I mixing things up here? Does the distance of an object from its shadow have the same effect for sunlight as for a hypothetical light bulb that has the same $\frac {OA}{OX}$ ratio as the Sun vs the Earth's surface?

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ In the wave picture of light you'll always have some diffraction such that shadows aren't completely sharp, too $\endgroup$
    – Quantum Mechanic
    Commented Feb 26, 2022 at 18:58
  • $\begingroup$ Thanks, interesting point. I imagine that kind of haze would always be very small, though, when the objects we consider are within the range we can see(?). $\endgroup$
    – user251959
    Commented Feb 27, 2022 at 8:13
  • $\begingroup$ Yes for sure, the main concepts when you're talking about the sun and shadows are rays $\endgroup$
    – Quantum Mechanic
    Commented Feb 27, 2022 at 17:40

3 Answers 3

Reset to default
2
$\begingroup$

An extended light source causes a shadow consisting of umbra, penumbra and antumbra

enter image description here

Only in the umbra (the core of the shadow) is the light source completely blocked. The other regions are partially lit be the light source. And you can see from the graphic that it depends on where you put your screen to observe the shadow. If you place it right behind the object casting the shadow, the latter will consists solely of an umbra (i.e. creating a dark, sharp shadow). If you go further away from the object, the umbra will become smaller and eventually disappear altogether, whilst the penumbra (the diluted shadow) becomes bigger.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks, this is interesting! The case I was considering was of a comparatively small object hit by sunlight on Earth. Your spherical orange/brown object here could be what I am thinking of, but given the actual Sun vs Earth scale, we could not observe its shadow on a screen that is significantly farther from the Sun than the object itself. If I hold up a hand 1 m from the ground or 0.1 m from the ground, it is in both cases still 150 million km from the Sun. So my question really is: can we ever observe the penumbra in the shadow of an object on Earth? $\endgroup$
    – user251959
    Commented Feb 27, 2022 at 8:24
  • $\begingroup$ @user6376297 But you calculated yourself in your question that the angle the sun makes is not much smaller than that of a light bulb from a couple of meters. The actual distance is irrelevant here. It is only about the relative angles the light source and the shadow casting object make as seen from the screen. $\endgroup$
    – Thomas
    Commented Feb 27, 2022 at 9:10
1
$\begingroup$

Imagine you hold an object in front of the Sun. Now slowly move it away so the sun becomes visible. The Sun will appear bit by bit (she's no point source). The amount of light you receive increases bit by bit, corresponding to a shadow diminishing bit by bit, i.e. an unsharp shadow.

Improve this answer
$\endgroup$
0
$\begingroup$

"For a very long time I never questioned the idea that sunrays hitting Earth are to all practical purposes 'approximately parallel'."

Consider the rays from point A on your diagram. @Thomas' diagram is not drawn to scale for the case of the source being the sun but because of the distance to the sun, the two rays from A would be very close to parallel. Likewise for the two rays from B. But the rays from A are not parallel to the rays from B.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.