When point light shines onto sphere, it creates circular shading. Are point light, centers of all shading circles and sphere collinear?

Question

Suppose a point that emits light in all direction. Some of the light rays hit a sphere and create circular shading on the sphere (in the figure, the shading is exaggerated a little through posterization). Is there a single line that goes through the point light, the centers of all the shading circles and the center of the sphere?

Edit: Okay, maybe the tag "shadow" might have caused some confusion so I removed it, even though at least in art the dark shading on an object, not the shadow that it casts onto another surface, can also be called "shadow". I'm not concerned about the shadow on the ground, I'm concerned only about the dark shading on the sphere itself.

Answer 1

The brief answer to your main question "Are point light, centers of all shading circles and sphere collinear? is "yes".

This is because the light cones defined by where the light intercepts the surface of the sphere at a tangent must have centres that lie on the line joining the light source to the centre of the sphere, due to the symmetry of the situation.

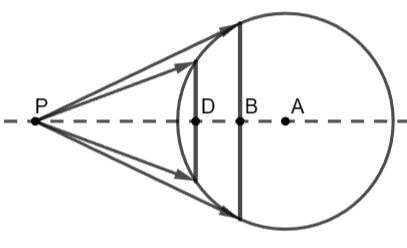
Each shade circle receives progressively less light going away from the source, not only because the light energy received reduces by a factor of $1/d^2$ (where d is the distance from the source to the point on the surface of the sphere) but because the angle subtended by the sphere surface also reduces, until in this case anything to the right of shade circle B is in total darkness. The subtended angles are the main reason that regions near the poles receive less solar radiation than regions near the equator.

If the light source emits W Joules/Second of light energy in all directions in the form of a sphere, then the power per unit area emitted is $$P = \frac{W}{4 \pi r^2}$$

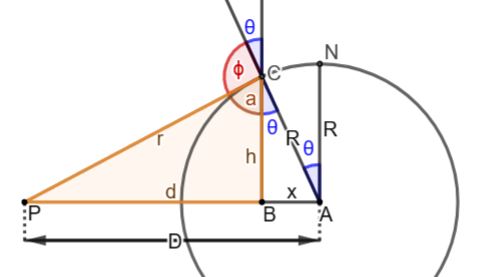
The amount of light energy per unit area (L) received at a point (C) on the surface of the illuminated sphere is given by:

$$P' = \frac{W}{4 \pi r^2} \cos(\phi) $$ where $\phi$ is the angle between the incoming light ray and the normal of the illuminated surface. (A similar equation is used to calculate how much light energy a horizontal solar panel receives depending on latitude.)

After some geometry, the amount of light energy received per unit time, per unit area is:

$$L = - \frac{W \cos^2 (a)}{4 \pi \cos^2(\theta) R^2} \cos( a + \theta)$$

where $$a = \tan^{-1}\left( \frac{D/R - \sin(\theta)}{\cos(\theta)} \right)$$

Only 4 parameters are required. The power of the light source (W), the radius of the illuminate sphere (R), the distance of the light source (D) from the centre of the illuminated sphere and the angle ($\theta$) from North of the point on the surface of the sphere.

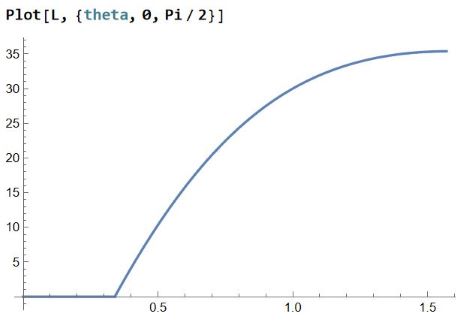
A plot of L versus the angle from North $\theta$ is shown below. It can be seen that there is angle where the light is cut off completely (around $\theta \lt 0.34$ in this example calculated using D = 3R).

Answer 2

The circumference of the sphere and the point source define a circular cone. If the shadow falls on a (possible curved) surface which is not perpendicular to the center of the cone, defining the center of the shadow may be a problem.