Can't understand Resonance in an Air Column

Question

In this image about resonating air column in my book, they say that resonance occurs at those specific lengths marked in the diagram. However,

• its also said that natural frequency of air column decreases with increase in length.
• resonance occurs when the natural frequency of tuning fork is equal to the natural frequency of the air column.

But in the figure, the frequency is same for $\frac{\lambda}4$, $\frac{3\lambda}4$ and $\frac{5\lambda}4$. How is that so? Shouldn't the tuning fork resonate with the air column at only one length, as the natural frequency of the air column is different for different lengths? Why is it not like the picture below for different lengths?

Answer 1

An air column of given length has many resonant frequencies. These are called the fundamental and the harmonics. The fundamental has the longest wavelength that can fit in the column and satisfy the right conditions at the two ends. In this example one end has to have no air oscillation (at the closed end) and the other has to have a maximum air oscillation (at the open end).

The fundamental thus has a longer wavelength and therefore a lower frequency as the air column gets longer.

The image shows three cases which all have the same wavelength and thus the same frequency: the fundamental of an air column of length $l_1$, then the first harmonic of an air column of length $3 l_1$ and the second harmonic of the air column of length $5 l_1$. Those longer air columns do have lower fundamental frequencies, but the person drawing the diagram was interested in the fact that a tuning fork of some given frequency will resonate with many different air columns, when the tuning fork hits the note of one of the harmonics.

The term 'natural frequency' is normally used for the fundamental.

Answer 2

Shouldn't the tuning fork resonate with the air column at only one length
No, as what in effect what is happening is that you are fitting in standing waves of a particular frequency (and hence wavelength) into tubes of differing lengths.

In the tube of the type that you have drawn when resonance occurs a displacement node is created at the closed end and a displacement antinode is created at the free end.
The diagrams you have drawn show this together with a representation of the standing wave which is formed in the tube.
So your left top diagram shows one node and one antinode separated by a quarter of a wavelength. The next two diagrams show the same frequency standing wave but more of it, 2 nodes and two antinodes, three modes and three antinodes.

Your bottom two diagrams show two standing waves with the left hand one having a smaller wavelength and hence a higher resonant frequency than the right hand one.

Answer 3

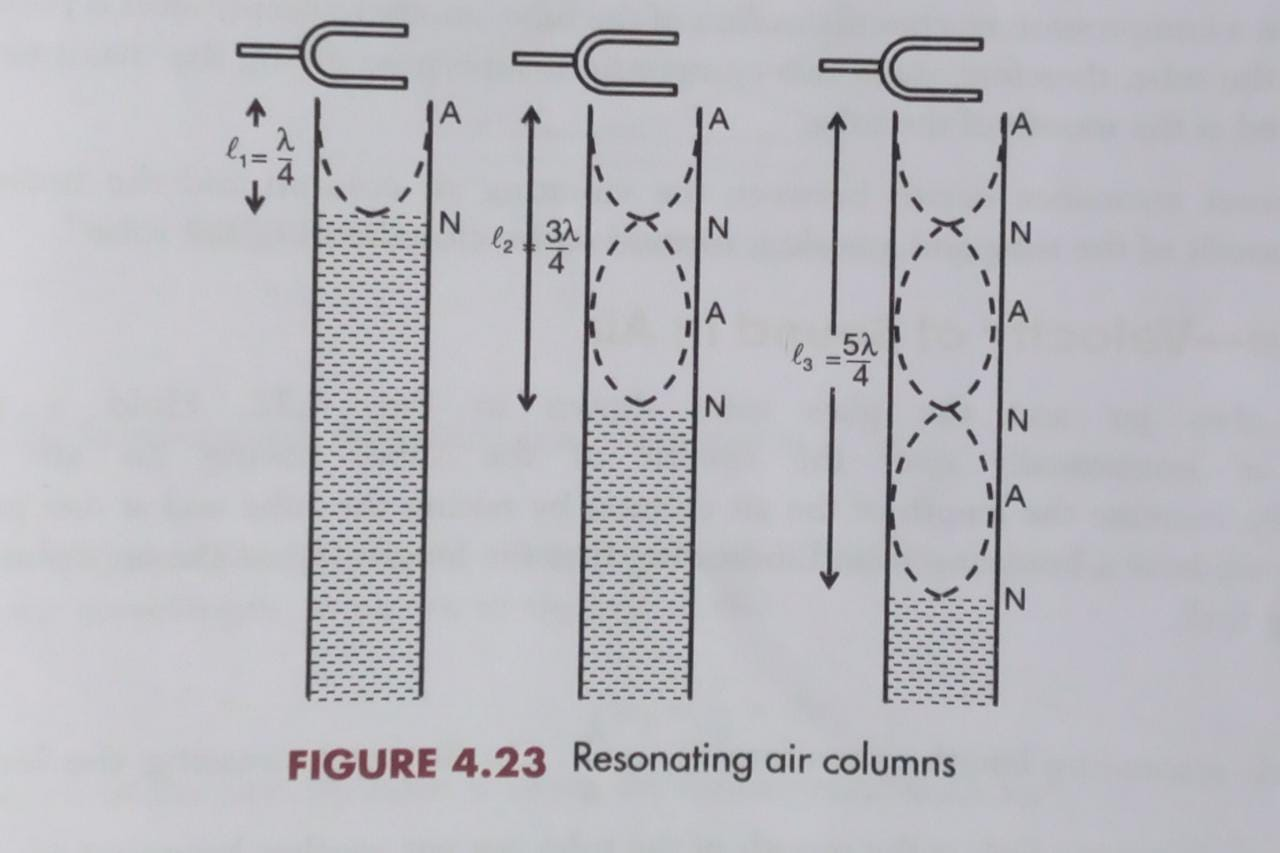
For a given length of air column, the maximum wavelength(or minimum frequency) that can produce resonance is known as fundamental mode, and the frequency at this length is referred to as the natural frequency.

Basically, the open end has to be a displacement anti-node and the other closed end is a displacement node. Separation between a consecutive anti-node and a node is $\lambda/4 $. Hence, waves which have wavelength $\lambda$ in an air column of length $l$ will produce resonance if $\lambda/4=l$, $3\lambda/4=l$ or $5\lambda/4=l$ and so on. Therefore the largest possible wavelength that would cause resonance is when $\lambda/4=l$.Wavelengths above this will not be able to resonate.

Furthermore, if speed of sound remains unchanged for different lengths the natural frequency will have an inverse proportionality with the length of the air column.

$f=\frac{v}{2l}$

$l$ is length of air column, v is speed of sound and f is natural frequency.

Now, if we keep the frequency same by using a tuning fork to produce sound waves, and change the length of the air column, then for that particular frequency $f$ there is particular wavelength $\lambda$ associated with it(as speed of sound is constant). For this wavelength there are different lengths$l_1, l_2, l_3....$ and so on which resonate so that $l_1=\lambda/4$; $l_2=3\lambda/4$; $l_3=5\lambda/4$.

The length which would be resonating at natural frequency would be $l_1$.Although, $l_2$ and $l_3$ would also be resonating but not at the length's natural frequency since for $l_2$ and $l_3$ the length is not quarter of the wavelength, which is the condition required for fundamental mode of resonance.