I took the following image from the book Sears and Zemansky's University Physics with Modern Physics by Young and Freedman.
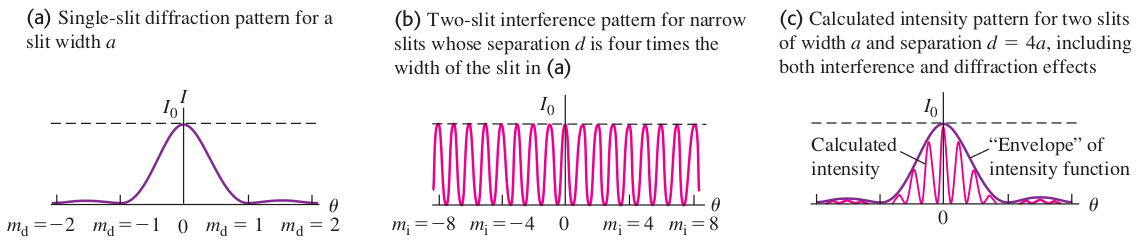
I don't understand why the relation $d = 4a$ makes the peak intensities in images (a) and (b) equal.
I took the following image from the book Sears and Zemansky's University Physics with Modern Physics by Young and Freedman.

I don't understand why the relation $d = 4a$ makes the peak intensities in images (a) and (b) equal.
I think there is some misunderstanding. Images (a), (b) and (c) are not drawn for the same intensity of light being shone on the slits. $I_0$ is just an arbitrary normalisation.
What these three images are meant to explain is the following. Unless a single slit is narrow enough (width $a$ is supposed small) you won't have a wide enough diffraction pattern. Image (a) shows a diffusion pattern with a single slit, and just calls $I_0$ the intensity at the center, for some intensity of light arriving on the slit.
If the single slit was much much narrower, you'd have a much wider pattern. Of course, since less light can get through a narrower slit and the light is diffracted on a wider area, you'd have even less light on the central area of the screen. Having the same $I_0$ would mean a much, much higher intensity incoming to the slit. This is not represented here, but it would be a horizontal line at some amplitude. Why not again $I_0$ ? One does not say the incoming intensity is the same ! The horizontal does not mean it goes to infinity, just much wider than on (a).
With tow very narrow slits you get pattern (b). It does not extend to infinity, but the idea is again that it extends much wider than (a). Again it is for a different intensity, just adjusted for the same $I_0$ at the center.
Finally two slits, distance 4$a$, but with width $a$. The overall shape is the same as (a). The interference pattern is the same as (b). The combined effect is (c) : an interference pattern limited under an envelope shaped like (a). But all the intensities for the incoming illumination are different for the same $I_0$.
I think the diagrams here only demonstrate relative intensity graphs. This means $I_{0}$ in (a) is not the same as $I_{0}$ in (b). The only thing they're interested in here is the shape of the intensity function, the location of the peaks/valleys, and the widths of the graphs.
The information $d = 4a$ here is only relevant to the angular widths of the two graphs in (a) and (b), and it is only relevant to how you obtain graph (c) by multiplying function (a) by function (b).
If I'm not mistaken, they don't ever go into the detail of how to calculate absolute intensity (which is possible but intro physics books leave it out because it is a very difficult topic), so there's not as much meaning to $I_{0}$ as you think there is.