At first, coin is spinning in own fixed reference frame (along axis which goes through the center of coin). So given this fact, it not differs much from spinning humming-top rotated by $90^{\circ}$. Both systems would be stable due to angular momentum conservation $\vec L = \vec r \times \vec p = \text{const}$, if no dissipation forces would be acting upon them (such as friction).
why does it start to move in circles
It's because gravity on coin COM, which has slope $\theta \gt 0$ from ground normal, induces centripetal acceleration.
what is the minimum angular velocity (dimensionally determined) for it to start this kind of trajectory
Very good question. At particular time $t$ coin moves in a trajectory with a curvature radius $R$ defined as :
$$ R = \frac {v^2}{g \sin \theta} $$
Where $v$,- coin tangential speed along trajectory curvature point. So from formula it can be seen that when coin acquires inclination angle $\theta \gt 0$ - it instantly starts to move along some curvature, with radius $R$. Coin can move technically in a straight line if and only if inclination angle from ground normal $\theta = 0$. (Put zero angle in a formula, and you'll get $R=\infty$, that's a metrics for a straight line.
As about experimental coin movement patterns,- there can be some distinct cases, as pictured below :
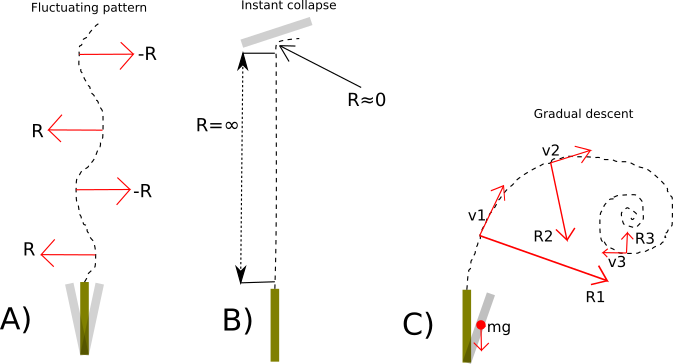
In case A) coin acquires random micro inclinations from ground normal and starts fluctuating in a repeating patterns of curvature radius $[-R,R]$.
In B) variant coin starting inclination is zero, so it initially moves in a straight line, but as it approaches zero speed, instantly random inclination is amplified by gravity and system collapses. In the last C) case initial speed is great and coin also has an initial non-zero inclination, so it starts to move in a spiral - with gradually decreasing curvature radius.
So the final answer is that coin will begin to move in a curved-way, when it will acquire inclination angle. Now, if you would ask when or in what conditions it will have inclination angle greater than zero - the answer is I don't know. This may depend on bunch of unknown factors, such as initial speed of coin, surface static and dynamic friction coefficients, air drag force, cross-section of coin, did or did not coin had an initial inclination angle (i.e. you gave it yourself by pushing coin), and etc.

