The resultant amplitude of $n$ equal oscillators

Question

There is a summation related to adding effect of many equal equally spaced oscillators.
I was reading the first few lines of Feynman's lectures on physics (chapter 30) and the way Feynman did the summation $\sum^{n-1}_{r=0}\cos(\omega t+r\phi)$ became a problem. He used a geometrical approach and this seems totally fine to me.

As I compute this summation algebraically, what I got is $$ \sum^{n-1}_{r=0}\cos(\omega t+r\phi)=\frac{\sin\left(\frac{n\phi}{2}\right)}{\sin\left(\frac{\phi}{2}\right)}\cos\left(\frac{n-1}{2}\phi\right) $$

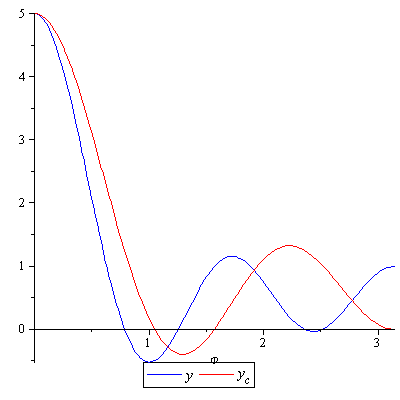
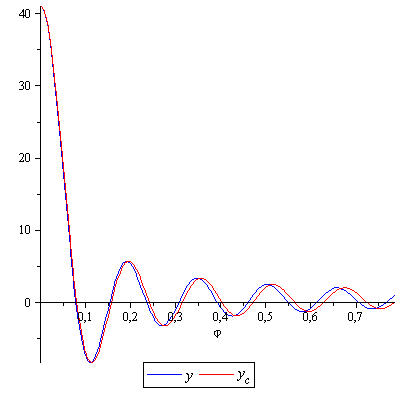
However, as suggested by Feynman, it should be $$\frac{\sin\left(\frac{n\phi}{2}\right)}{\sin\left(\frac{\phi}{2}\right)}$$ He has a perfect geometrical interpretation for that but the result is just different. So I used desmos to draw them, the green curve in the picture is his answer, the overlapped purple and red curves are the summation itself and my answer respectively.
Did I misundertood something? Anyway I just don't know what is going on. If Feynman's geometry method is wrong, why is that? My peaceful weekend has already been destoryed by this contradiction.

Answer 1

Algebraically you are correct: $$ \sum_{r=0}^{n-1}\cos (\omega t +r \phi)= {\rm Re}\left\{ \sum_{r=0}^{n-1}e^{i( \omega t +r \phi)}\right\}\\= {\rm Re}\left\{ e^{i\omega t} \frac{1-e^{in\phi}}{1-e^{i\phi}}\right\}\\ = {\rm Re}\left\{ e^{i\omega t}e^{i(n-1)\phi/2} \frac{\sin (n\phi/2)}{\sin (\phi/2)}\right\}\\ =\cos(\omega t+ (n-1)\phi/2) \frac{\sin (n\phi/2)}{\sin (\phi/2)},$$ but I think that he is referring to the length of the phasor, rather than its projection. In other words he is thinking that the sum is
$$ \cos(\omega t+phase) \frac{\sin (n\phi/2)}{\sin (\phi/2)}, $$ and is focussing on the amplitude of the oscillation which is $$ \frac{\sin (n\phi/2)}{\sin (\phi/2)}. $$

Answer 2

this is the referent solution

$$y=\sum_{r=0}^n\,\cos(r\,\varphi)$$

your summation is

$$y_c={\frac {a\sin \left( 1/2\,\varphi \,n \right) \cos \left( 1/2\, \left( n-1 \right) \varphi \right) }{\sin \left( 1/2\,\varphi \right) }} $$

where a is the amplitude a $~\varphi=0$

thus

$$y(\varphi=0)=y_x(\varphi=0)\quad\Rightarrow\\ a=\frac{n+1}{n}$$

compare $~y~$ with $~y_c$

n=4

n=40

thus for n >> you obtain the same result