Reaction force and normal force for falling and sliding rod

Question

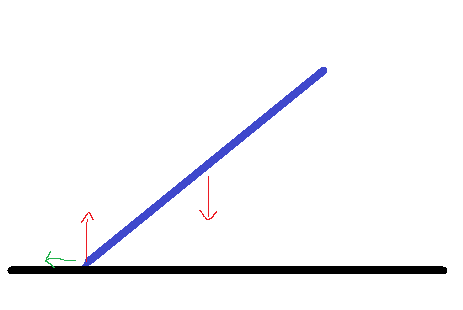
In the following picture, the blue bar represents a rod that is falling from the sky and the black line represents the ground. Hence, in the picture, the moment of the impact is shown. We assume the ground to be frictionless. Now, when time goes on, the rest of the rod will move towards the ground. However, while doing so, the rod will slide on the ground to the left (green arrow). At the contact point, there will be a normal force (red arrow). The gravitational force is also depcited by a red arrow.

Are the normal force and the gravitational force equal in magnitude and opposite (as indicated in the picture)? And where is the reaction force to the force acting in the direction of the green arrow?

Answer 1

Vertical forces. The reaction to the normal force from the ground on the rod is the normal force from the rod on the ground. The reaction to the gravitational force from the Earth on the rod is the gravitational force from the rod on the Earth. While the normal force and gravitational force are opposite in direction here, there's no reason they must be equal in magnitude.

Horizontal forces. The surface is frictionless; hence, there are no horizontal forces, i.e. no forces acting in the direction of the green arrow.

Motion of the rod. Since there are no horizontal forces acting on the rod, momentum is conserved in the horizontal direction. This means the trajectory of the center of mass of the rod follows a straight vertical line. However, you're still right that the left end of the rod will accelerate to the left when it impacts the ground. This doesn't contradict anything we've established, since internal forces are responsible for the acceleration of this piece of the rod. Note that the right end of the rod accelerates with the same magnitude to the right when this happens, so the horizontal acceleration of the center of mass of the rod remains zero.

Answer 2

The acceleration of the center of mass of the rod is the net force on the rod divided by the mass of the rod.

In reality the rod will bounce as it impacts the ground, but let's assume it does not to address your question.

The net force downward at the instant of impact is the force of gravity minus the normal force and has no sideways component, so the center of mass moves only vertically downward; sideways motion of the center of mass does not occur due to the rod slipping sideways to the left as shown by your green arrow. There is no force sideways on the rod; just sideways slipping of the rod.

Answer 3

Assume the rod to be of mass $m$, uniform and completely rigid. The floor is also perfectly rigid and there's no friction between floor and rod, which prevents any sideways motion on or after impact.

The collision is therefore perfectly elastic.

The translational kinetic energy is completely converted to rotational kinetic energy, for rotation about the CoG:

$$\frac12 mv^2=\frac12 I\omega^2$$ where $v$ is the translational velocity of the rod at impact. $$\frac12 mv^2=\frac12 \frac{1}{12}mL^2 \omega^2$$ $$v^2=\frac{1}{12}L^2\omega^2$$ $$\omega=2\sqrt{3}\frac{v}{L}$$

Answer 4

the Energy is:

$$E=\frac 12\,m{v}^{2}+\frac 12\,I_{{D}}{\omega}^{2}+m\,g\,h=0$$

at $~t=0$

$$E_i=E\left(v=0~,\omega=0~,h=h_0\right)$$ and at the final position

$$E_f=E\left(h=\frac{L}{2}\,\sin(\alpha)\right)$$

with $~E_i=E_f~$ you obtain the velocity of the center of mass at the final position $$v_{\text{CM}}^2=-{\frac {I_{{D}}{\omega}^{2}+m\,g\,L\sin \left( \alpha \right) -2\,m\,g\,h_{{0 }}}{m}} $$

the velocity at the contact point $~P~$ is:

\begin{align*} \vec v_P= \begin{bmatrix} w_x \\ w_y \\ w_z \\ \end{bmatrix}= \begin{bmatrix} 0 \\ v_{\text{CM}} \\ 0 \\ \end{bmatrix}+ \begin{bmatrix} 0 \\ 0 \\ \omega \\ \end{bmatrix}\times \frac L2\begin{bmatrix} \cos(\alpha) \\ \sin(\alpha) \\ 0 \\ \end{bmatrix} \end{align*}

the collision equations

$$m\,(v_y-w_y)=dp_y\\ m\,(v_x-w_x)=0\\ v_y=-w_y$$

from here you obtain the impuls $~dp_y~$ integrating you obtain the reaction force $~F_y$

$$F_y=\int dp_y\,dt=-\omega\,L\cos \left( \alpha \right) m+2\,\sqrt {-m \left( I_{{D}}{ \omega}^{2}+m\,g\,L\sin \left( \alpha \right) -2\,m\,g\,h_{{0}} \right) } \,t$$