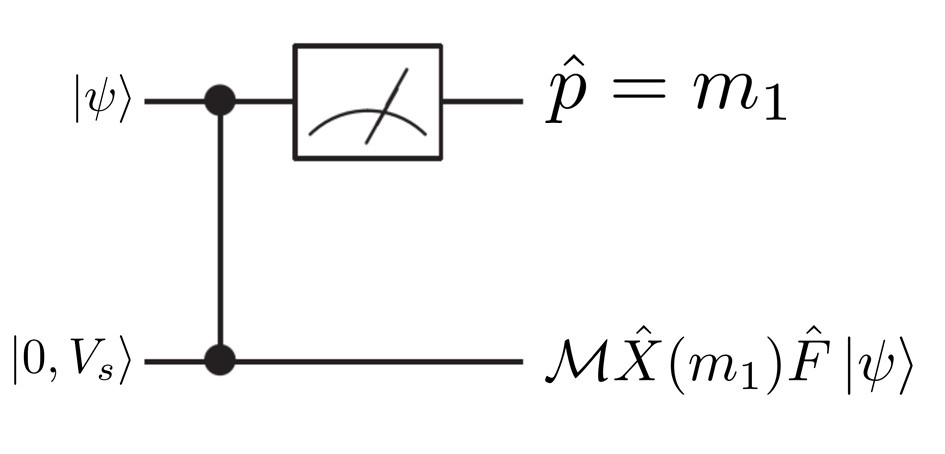I am studying the continuous variable teleporting circuit using a vacuum squeezed state showed by Weedbrook, pag. 39-40. Section: Gaussian errors from finite squeezing; in that, they propose an arbitrary state $\left|\psi\right\rangle$ (which we pretend to teleport) and a squeezed vacuum state $\left|0,V_{s}\right\rangle$ as the inputs of the following quantum circuit:
These states are expanded in position basis according to (all integrations span from -$\infty$ to $\infty$)
$$\left|\psi\right\rangle = \int ds_{1}~ \psi(s_{1}) \left|s_{1}\right\rangle_{q}, \tag{1}$$ $$\left|0, V_{s}\right\rangle = (\pi V_{s})^{-1/4}\int ds_{2}~ e^{-(s_{2})^2/2V_{s}} \left|s_{2}\right\rangle_{q}, \tag{2}$$
where $V_{s}$ is a positive parameter associated with the variance of the state and the $ \left\lbrace \left|s_{i}\right\rangle_{q}\right\rbrace$ is the position quadrature basis which is related with the momentum basis $ \left\lbrace \left|s_{i}\right\rangle_{p}\right\rbrace$ through a fourier transform (units of $\hbar=2$):
$$\left|s_{i}\right\rangle_{q}=\left(2\sqrt{\pi} \right)^{-1}\int dp ~e^{-i qp/2} \left|s_{i}\right\rangle_{p}, \tag{A}$$ $$\left|s_{i}\right\rangle_{p}=\left(2\sqrt{\pi} \right)^{-1}\int dp ~e^{i qp/2} \left|s_{i}\right\rangle_{q}. \tag{B}$$
Hence, the input state is the product
$$\left|\psi\right\rangle \left|0, V_{s}\right\rangle = (\pi V_{s})^{-1/4}\int ds_{1} ds_{2}~ \psi(s_{1}) e^{-(s_{2})^2/2V_{s}} \left|s_{1}\right\rangle_{q} \left|s_{2}\right\rangle_{q}. \tag{3}$$
Then, according to the circuit, we apply a CPHASE gate (the vertical line joining the two inputs) to the initial state, this gate is defined as $\hat{C}_{z}=e^{i \hat{s}_{1} \hat{s}_{2}/2}$; therefore we have
$$\hat{C}_{z}\left|\psi\right\rangle \left|0, V_{s}\right\rangle = (\pi V_{s})^{-1/4}\int ds_{1} ds_{2}~ \psi(s_{1}) e^{-(s_{2})^2/2V_{s}} e^{-i s_{1} s_{2}/2}\left|s_{1}\right\rangle_{q} \left|s_{2}\right\rangle_{q}. \tag{4}$$
Now, according to the diagram of the circuit, we perform a momentum measurement on the first mode (the top wire of the circuit) through the projector $\hat{P}_{m_{1}}=\left|m_{1}\right\rangle \left\langle m_{1} \right| $, hence we obtain the result $m_{1}$; therefore, the quantum state of the system after this measurement is
$$ \hat{P}_{m_{1}}\hat{C}_{z}\left|\psi\right\rangle \left|0, V_{s}\right\rangle=\left(\pi V_{s} \right)^{-1/4}\int ds_{1}ds_{2}~\psi(s_{1})e^{-s_{2}^{2}/2V_{s}} e^{is_{1} s_{2}/2} \left|m_{1}\right\rangle_{pp}\left\langle m_{1} \right|\left. s_{1}\right\rangle_{q}\left|s_{2}\right\rangle_{q} \\ =\left(\pi V_{s} \right)^{-1/4}\left(2\sqrt{\pi} \right)^{-1}\int ds_{1}ds_{2}~\psi(s_{1})e^{-s_{2}^{2}/2V_{s}} e^{is_{1} s_{2}/2} e^{-im_{1}s_{1}/2}\left|m_{1}\right\rangle_{p}\left|s_{2}\right\rangle_{q}, \tag{5}$$
where we have used $\left\langle m_{1} \right|\left. s_{1}\right\rangle_{q}=\left(2\sqrt{\pi} \right)^{-1} e^{-im_{1}s_{1}/2}$. Hence, the state on the second mode (the bottom wire) is
$$\left(\pi V_{s} \right)^{-1/4}\left(2\sqrt{\pi} \right)^{-1}\int ds_{1}ds_{2}~\psi(s_{1})e^{-s_{2}^{2}/2V_{s}} e^{is_{1}\left(s_{2} - m_{1} \right)/2}\left|s_{2}\right\rangle_{q}=\mathcal{\hat{M}}\hat{X}(m_{1}) \hat{F}\left|\psi\right\rangle, \tag{6} $$
where we identify the Gaussian distortion $$ \mathcal{\hat{M}} = \left(\pi V_{s} \right)^{-1/4} \int e^{-\left(s_{2}'\right)^{2}/2V_{s}} \left|s_{2}'\right\rangle_{qq} \left\langle s_{2}' \right|, \tag{C} $$ the Fourier gate $\hat{F}=\exp\left[i \pi (\hat{q}^2 + \hat{p}^2)/8 \right]$, which acts on the quadrature eigenstates according to $$ \hat{F} \left|s \right\rangle_{q} =\left|s \right\rangle_{p}, ~~~~\hat{F}^{\dagger} \left|s \right\rangle_{p} =\left|s \right\rangle_{q}, \tag{D} $$ and the displacement gate $\hat{X}(m_{1})=e^{-im_{1} \hat{p}/2}$ acting on the quadrature eigenstates as follows $$\hat{X}(m_{1})\left|{s}\right\rangle_{q}=\left|s + m_{1}\right\rangle_{q}, ~~~~\hat{X}(m_{1})\left|{s}\right\rangle_{p}=e^{-im_{1} s/2}\left|s\right\rangle_{p}. \tag{E}$$
Hence the quantum state of the second wire is written as $\mathcal{\hat{M}}\hat{X}(m_{1}) \hat{F} \left|\psi\right\rangle$ (see the diagram of the circuit); we give an inverse proof as follows:
Let us take the arbitrary state $\left|\psi\right\rangle$ expanded in position basis: $\left|\psi\right\rangle=\int ds_{1} \psi(s_{1}) \left|s_{1}\right\rangle_{q}$. Applying the Fourier gate $\hat{F}$ (see Eqs. (D)) we have $$ \hat{F}\left|\psi\right\rangle=\int ds_{1} \psi(s_{1}) \hat{F}\left|s_{1}\right\rangle_{q}=\int ds_{1} ~\psi(s_{1}) \left|s_{1}\right\rangle_{p}, \tag{7} $$ applying the displacement gate $\hat{X}(m_{1})$ (see Eqs. (E)) to the state $\hat{F}\left|\psi\right\rangle$ we have $$ \hat{X}(m_{1})\hat{F}\left|\psi\right\rangle=\int ds_{1} ~\psi(s_{1}) e^{-im_{1}s_{1}/2}\left|s_{1}\right\rangle_{p}, \tag{8} $$ expanding $\left|s_{1}\right\rangle_{p}$ in terms of position basis $\left|s_{2}\right\rangle_{q}$ (see Eq. (B)): $\left|s_{1}\right\rangle_{p}=\left(2\sqrt{\pi}\right)^{-1}\int ds_{2}~ e^{is_{2}s_{1}/2} \left|s_{2}\right\rangle_{q}$ the state $\hat{X}(m_{1})\hat{F}\left|\psi\right\rangle$ can be written as
$$\hat{X}(m_{1})\hat{F}\left|\psi\right\rangle=\left(2\sqrt{\pi} \right)^{-1}\int ds_{1}ds_{2} ~\psi(s_{1}) e^{is_{1}\left(s_{2} - m_{1} \right)/2}\left|s_{2}\right\rangle_{q}, \tag{9}$$
then, finally, we apply to the last state the Gaussian distortion given by Eq. (C), obtaining
$$ \hat{\mathcal{M}}\hat{X}(m_{1})\hat{F}\left|\psi\right\rangle=\frac{\left(\pi V_{s} \right)^{-1/4}}{\left(2\sqrt{\pi} \right)}\int ds_{1} ds_{2} ds_{2}'~ \psi(s_{1}) e^{-\left(s_{2}'\right)^{2}/2V_{s}} e^{is_{1}\left(s_{2} - m_{1} \right)/2}\left|s_{2}'\right\rangle_{qq}\left\langle s_{2}'\right.\left|s_{2}\right\rangle_{q} \\ =\frac{\left(\pi V_{s} \right)^{-1/4}}{\left(2\sqrt{\pi} \right)}\int ds_{1} ds_{2} ds_{2}'~ \psi(s_{1}) e^{-\left(s_{2}'\right)^{2}/2V_{s}} e^{is_{1}\left(s_{2} - m_{1} \right)/2}\left|s_{2}'\right\rangle_{q}\delta(s_{2}'-s_{2}) \\ =\left(\pi V_{s} \right)^{-1/4}\left(2\sqrt{\pi} \right)^{-1}\int ds_{1} ds_{2} ~ \psi(s_{1}) e^{-s_{2}^{2}/2V_{s}} e^{is_{1}\left(s_{2} - m_{1} \right)/2}\left|s_{2}\right\rangle_{q}. \blacksquare \tag{10} $$ The last line of the above equation is equal to the state obtained on the second mode (see Eq. (6)) of the circuit after the projective measurement.
Therefore, my questions are:
How to recover as much as possible the original state $\left|\psi\right\rangle$?. Since the output from the second wire after the measurement is $\hat{\mathcal{M}}\hat{X}(m_{1})\hat{F}\left| \psi \right\rangle $ and since $\hat{\mathcal{M}}$ is clearly a non-unitary operator: $\hat{\mathcal{M}}^{\dagger}\hat{\mathcal{M}}\neq \hat{\mathbb{I}}$, we cannot do $\hat{F}^{\dagger}\hat{X}(m_{1})^{\dagger}\hat{\mathcal{M}}^{\dagger}\hat{\mathcal{M}}\hat{X}(m_{1})\hat{F}\left|\psi\right\rangle$ in order to recover $\left|\psi\right\rangle$. Besides, in the article (bellow Eq. (176)), the authors say (if I understand correctly) that what we really have in the second wire is the original state with a Gaussian distortion which have zero mean and variance $1/V_{s}$. I cannot see this argument directly from the output state $\hat{\mathcal{M}}\hat{X}(m_{1})\hat{F}\left|\psi\right\rangle$; so, can someone give me some hint to mathematically prove this argument?.
Is my Gaussian distortion (Eq. (C)) wrong?. I am a little confused with Eq. (176) of the article, where is showed the effect of $\hat{\mathcal{M}}$ on $\left|\psi\right\rangle$:
$$ \hat{\mathcal{M}}\left|\psi\right\rangle\propto \int dq~ e^{q^2 V_{s}/2} \left|q\right\rangle \left\langle q\right| \left. \psi \right\rangle. $$
From this equation, it seems to come the argument that the output state in the second wire is a Gaussian distortion with variance $1/V_{s}$ applied to the original state $ \left| \psi \right\rangle$. From this equation I can see $\hat{\mathcal{M}}=\int dq~ e^{q^2 V_{s}/2} \left|q\right\rangle \left\rangle q\right|$, which is not coincident with my Gaussian distortion given in Eq. (C).