Is an Anti-positron actually only a relativistic effect on the observed helicity of an electron?

Question

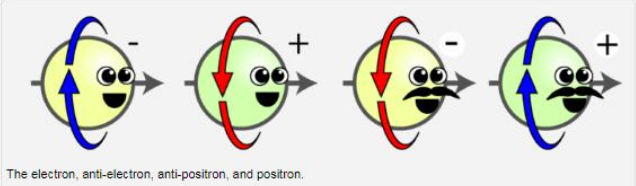
I have read quite recently this article here:

https://www.quantumdiaries.org/2011/06/19/helicity-chirality-mass-and-the-higgs/

in which it is explained that an Anti-positron is actually a different discrete particle from an electron.

This confused me because I thought an "Anti-positron" being an alternative name for the electron.

Is an Anti-positron really a discrete different particle from an electron or just the same electron particle as relativistically observed by a faster moving observer frame of reference, overtaking the electron and therefore observing a flip on its helicity for example from left handed (left helicity) to right handed (right helicity)?

Both of the Anti-positron and electron have the same charge invariance namely, charge -e.

Also what happens to the chirality of these two types of particles (could be the same particle)? Is chirality an invariant in this case, meaning the left chiral electron due to a relativistic observer, observed as a Anti-positron is still regarded of being left-chiral? Or for example because it has flipped relativistically its helicity from left to right has now also a right chirality?

Illustration source credits: https://www.quantumdiaries.org/2011/06/19/helicity-chirality-mass-and-the-higgs/

(gray arrow is the momentum direction of the fermion particles)

warning: the above illustration retrieved from the above mentioned source link comparing the relative helicities of electron and positron particles with their Anti-particles versions could not be entirely correct. Curled arrows indicate the spin's rotational direction and with red indicating a right-handed particle (right helicity) and with blue a left-handed particle (left heliity).

Answer 1

The article you link is choosing to use non-standard terminology to explain how the weak force can couple to particles with a certain handedness but not others. I do something similar with "electron-1" and "electron-2" particles in this answer of mine - it's a pedagogic trick, not a claim about things being "really" different particles.

Standard usage is to call both the left-handed and the right-handed versions of a negatively charged lepton with the mass of an electron "electron", and both the left-handed and the right-handed versions of a positively charged lepton with the mass of an electron "positron". Since the electron is massive, the two versions with different handedness couple to each other - the equations of motion for a massive Dirac field mean that a left-handed solution does not stay purely left-handed and likewise for the right-handed versions. So, ordinarily, it is natural to not view the states of different handedness as "different particles" since they almost always occur in a mixture anyway. Therefore, standard usage only speaks of "the electron" and "the positron" - a particle that evolves into another particle just by being left alone (with no decay products or anything) isn't usually what we think of as a distinct particle.

In the context of the weak force (or any other hypothetical chiral interaction) however, it is only the parts with a certain handedness that interact, and since we usually say that particles either interact or don't interact (and not that "one half of a particle interacts" or whatever), in this context it can be useful to conceptualize the chiral parts of the full Dirac electron as "different particles". This doesn't change anything about what real electrons and positrons are (namely almost always mixtures of these two chiral states), it's just a different way of speaking about them.