What is the meaning or definition of 'correlation length' in the context of Anderson localization?

Question

I was reading a paper that talks about Anderson localization. It mentions the quantity called 'correlation length' or 'localization length' but no formal definition is given as to what it actually means. I tried browsing the web but the definitions do not make sense in the context of Anderson localization.

Answer 1

For the Anderson localisation-delocalisation transition, the localisation length and the correlation length are used interchangeably.

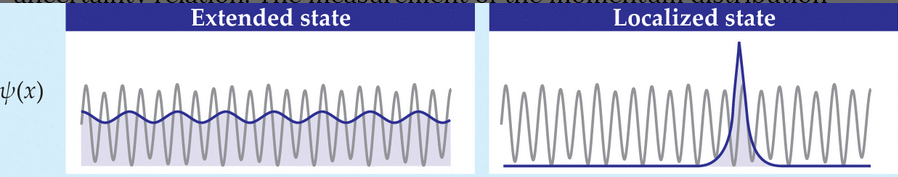
The whole point of a (disorder-induced) localised state is that, by definition, it is not extended, that is it has a finite spatial extent, usually confined/centred onto a single lattice site (image below):

If this central site has coordinate $x_0$ (in 1D for simplicity), then the probability density of the localised wavefunction will look something like this:

$$|\psi(x)|^2 \sim \exp \left ( -\frac{|x-x_0|}{\xi} \right ), $$

where $\xi$ is the localisation length, i.e. the spatial extend of the wavefunction.

Like the correlation length for conventional phase transitions, this one also follows a critical-exponent-governed relationship such as:

$$ \xi \sim \frac{1}{|E-E_c|^\nu},$$ though for the Anderson case (random disorder) the above relation is only true in 3D, because in $d<3$ there is no critical energy $E_c$ - any amount of disorder leads to localisation.

A disorder-induced localised phase cannot and does not thermalise, hence it cannot be described by any thermodynamic equilibrium ensemble, rendering common techniques of statistical mechanics powerless. You cannot show that the heat capacity (for instance) is discountinous, in order to pinpoint the transition. Hence the localisation length is a useful "alterative" to an "order parameter" in that it has a different value for the localised and extended phase: finite $\xi$ for the former (and decreasing $\xi$ for stronger disorder), and $\xi \rightarrow \infty$ for the latter, where the limit is taken in the thermodynamic limit.