Is this a Dirac delta function in disguise?

Question

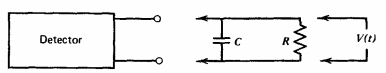
Consider a typical detector equivalent circuit, where the detector can be seen as an ideal current generator $I(t)$. Since $I = I_C + I_R$ (currents through the capacitor and resistor), $I_R R=V$ and $\frac{I_C}{C}=\frac{dV}{dt}$, the equation of the circuit is:

$$\frac{dV}{dt}+\frac{V}{RC}=\frac{I}{C}.$$

When $C$ goes to $0$, the equation should read $V=IR$ as it happens in detectors with a low $RC$ constant. But if I wanted to send $C$ to zero in the solution of the differential equation, which is $V(t)=\int_0^t \frac{I}{C}e^{\frac{t'-t}{RC}}dt'$, to obtain the same exact result, I would be brought to think that $e^{\frac{t'-t}{RC}}$ is kind of double a Dirac delta function. Substituting in the integral: $$V(t)=\int_0^t \frac{I}{C}2\delta(t'-t)RCdt'=I(t)R.$$

Is it actually a delta? Is it correct to reason this way?

Answer 1

1. Yes, OP has essentially constructed a one-sided Dirac delta distribution $$\delta_{[0,\infty[}(x)~=~\lim_{\varepsilon\searrow 0} \frac{1}{\varepsilon} e^{-x/\varepsilon}$$ on the positive half line $[0,\infty[$ via a generalized function, so that $$\int_{[0,\infty[} \mathrm{d}x~\delta_{[0,\infty[}(x)~f(x)~=~f(0) $$ for all test functions $f:[0,\infty[\to \mathbb{R}$.

2. Notice that OP's distribution is different from the usual two-sided Dirac delta distribution $\delta_{\mathbb{R}}(x)$ on the real line $\mathbb{R}$, which satisfies $$\int_{\mathbb{R}} \mathrm{d}x~\delta_{\mathbb{R}}(x)~f(x)~=~f(0) $$ for all test functions $f:\mathbb{R}\to \mathbb{R}$.

3. This also explains why OP's last formula has a correction factor of 2.

Answer 2

A more mathematical treatment

We have an ordinary differential equation (ODE): $$\alpha^{-1}y'(t) + y(t) = f(t).$$ In our case, $y(t)=V(t),$ $\alpha=\frac{1}{RC},$ $f(t)=RI(t).$

The ODE can be solved by finding a Green's function $G_\alpha(t)$ satisfying $$\alpha^{-1}G_\alpha'(t) + G_\alpha(t) = \delta(t)$$ and then get the solution $y(t)$ as $$y(t) = (G_\alpha*f)(t) = \int_{-\infty}^{\infty} G_\alpha(t-t')\,f(t')\,dt'.$$

A Green's function that only gives a response for $t>0$ (so that it's causal) is given by $$G_\alpha(t) = \alpha e^{-\alpha t} H(t),$$ where $H(t)$ is the Heaviside step function.

Distributions and convergence as such are defined by how they "act" on, or work in an integral when multiplied with, a test function $\varphi\in C^\infty_c(\mathbb{R}).$ Therefore, to show that $G_\alpha(t) \to \delta(t)$ in the sense of distributions, when $\alpha\to\infty,$ we need to show that $$ \lim_{\alpha\to\infty} \int_{-\infty}^{\infty} G_\alpha(t)\,\varphi(t)\,dt = \int_{-\infty}^{\infty} \delta(t)\,\varphi(t)\,dt = \varphi(0) $$ for all $\varphi\in C^\infty_c(\mathbb{R}).$

That is easy. Using the variable change $t=s/\alpha$ we get $$ \int_{-\infty}^{\infty} G_\alpha(t) \, \varphi(t) \, dt = \int_{-\infty}^{\infty} \alpha e^{-\alpha t} H(t) \, \varphi(t) \, dt = \int_{0}^{\infty} \alpha e^{-\alpha t} \, \varphi(t) \, dt = \int_{0}^{\infty} \alpha e^{-s} \, \varphi(s/\alpha) \, ds/\alpha \\ = \int_{0}^{\infty} e^{-s} \, \varphi(s/\alpha) \, ds \to \int_{0}^{\infty} e^{-s} \, \varphi(0) \, ds = \int_{0}^{\infty} e^{-s} \, ds \, \varphi(0) = 1 \cdot \varphi(0). $$