I understand standard RC circuits perfectly well, but I don't understand what the $R$ term in the capacitor charge equation for RC circuits represents in more complex situations. The actual problem I'm solving is below:
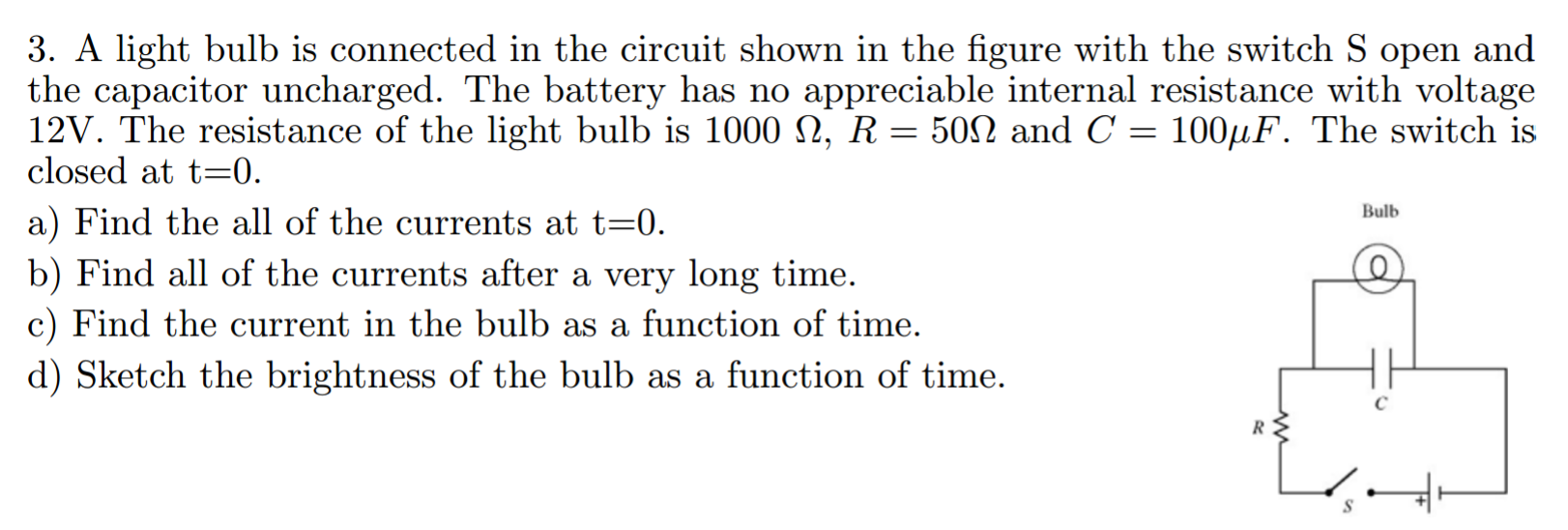
Part C is the part that I'm finding tricky. I know that $\Delta V$ across the bulb is equal to $\Delta V$ across the resistor, and I know that:
$$I_c = \frac{Ve^{\frac{-T}{RC}}}{R}$$
I also know that $\frac{I_c}{V_c}$ is going to be the effective resistance of the capacitor, and that I can find this using the equation above. From here, I know that I can proceed to easily work out all the voltages and currents.
The roadblock I'm running into is figuring out what R is in the formula for $I_c$. I know that it's the resistance in the circuit, but the resistance of what part of the circuit? My instinct is to say "just the resistance of the resistor" but I know this isn't true because changing the resistance in the lightbulb (e.g. making it zero or infinite) would obviously affect the rate at which the capacitor charged. At the same time, there is no obvious way that I can see to account for the resistance of the lightbulb nor a way to create an equivalent circuit that is more straight-forward.
Thank you for you help!
