A photons energy is given by $E=h *f$ and momentum $p=E/c$ (spin?) but the photon has no (rest) mass! Therefore it is the ultimate probing tool for looking at any mass position and velocity because mass transfer is not involved only momentum.But to calculate/ measure the particles exact position by scattering a photon from the mass into a detector dictates some of the photons momentum has been transferred to the mass i.e. the mass has to move (is disturbed from it initial position by some delta). This is intuitively obvious ;I would like to see how Heisenberg quantified(proved) this uncertainty hopefully without referring to an ansatz wave function.
$\begingroup$
$\endgroup$
1
-
1$\begingroup$ The "uncertainty" you're describing is not the same uncertainty that appears in Heisenberg's principle. Maybe that's the answer you're looking for, and if so I'll post it as such, but before it comes to that I would suggest reading some of the related questions linked at the right, such as this one, and seeing whether they can help you clarify your question. $\endgroup$– David ZCommented May 19, 2013 at 7:06
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
Take a billiard ball sitting on a table. Glance off it another billiard ball. Part of the energy of the moving ball will be taken up by the scattered, and the resulting energy and momentum conservation will be subject to measurement errors, rotational possibilities, friction on the table and the balls etc. These are the normal measurement errors.
Now if you take a photon and scatter it off an electron, it can Compton scatter , and the energy of the photon, and therefore its frequency will be less, whereas the electron will move in a trajectory. There are measurement errors similar to billiard ball errors, how well we know the frequency/energy of the photon, how well we measure the energy of the outgoing electron.
In this bubble chamber picture we see the electron scattered and could measure its momentum, with measurement errors not different in concept than classical measurement errors. If we had the interaction from which the photon came we might also know the impinging photon's energy from the energy balance of the reaction, again all classical measurement errors.
The Heisenberg Uncertainty Principle , HUP, is something different . It tells us that no matter how well we can measure the (x,y,z) and momentum of a particle the position and momentum are controlled by an intrinsic probabilistic uncertainty,
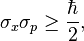
that cannot be overcome by any means. The better we know the momentum, the less we know the position or the converse. These numbers substituded in the dimensions of the bubble chamber measurements are in any case fulfilled, because the bubble chamber measurement accuracy is microns and the momentum accuracies are in the keV range, so HUP is fulfilled . It is when one wants to explore very small regimes that HUP becomes important.
