0
$\begingroup$

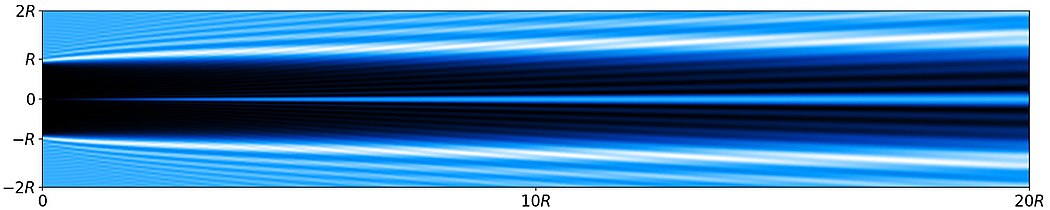
I want to combine the set-ups of the Arago spot (small disk in the light path, diffraction pattern imaged directly behind it) with the set-up of the Airy disk (small aperture in the light path, diffraction pattern imaged far away). That is, I have a fairly small solid sphere in the light path (radius $R = 30\lambda$), and I'm imaging far away ($d = 4000 R$). What should I expect to see on the screen? I'd like to start with the assumption that the light source is infinitely far away, although if it's easy to extend it to the case of light rays that aren't exactly parallel, that would be neat too.

(Background, I'm a mathematician by background but have ended up working with optical systems.)

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

This Numerical simulation by GONDRAN Alexandre is for $R=10\lambda$ enter image description here
The figure on p.5 here goes out to 30R.

At $4000R$ you are out of the Fresnel regime. (See the condition on the Fresnel number in Wikipedia). I can't find an image of Fraunhofer diffraction from a disk, but by Babinet's principle it looks (roughly) like the negative of the Airy disk from an aperture of the same radius.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Do you mean that you wouldn't expect to not be able to see the shadow from the object at all? That doesn't seem to be true in practice. Or do you mean that you would expect it to look pretty uniform? To clarify, I wasn't expecting to see the Arago spot, I just figured it would be the most concise way of describing the set-up I have ("small disk in a long optical path"). $\endgroup$
    – Elena Yudovina
    Commented Jun 10, 2021 at 20:43
  • $\begingroup$ See here about Fraunhofer diffraction and Fresnel diffraction regimes. I think you are in the former. $\endgroup$
    – Keith McClary
    Commented Jun 10, 2021 at 21:44
  • $\begingroup$ p.5 here "The patterns of a circular aperture and a opaque disk are complements of each other." So you should be able to morph from (negative) Arago to Airy, as this old video does by varying the width of a slit. $\endgroup$
    – Keith McClary
    Commented Jun 11, 2021 at 3:56
  • 1
    $\begingroup$ By Babinet's principle you should see the negative of p.3 here. $\endgroup$
    – Keith McClary
    Commented Jun 11, 2021 at 16:18
  • $\begingroup$ Thank you! I think that is what I was looking for. If you add it to your answer, I'll formally accept it. $\endgroup$
    – Elena Yudovina
    Commented Jun 11, 2021 at 22:10

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.