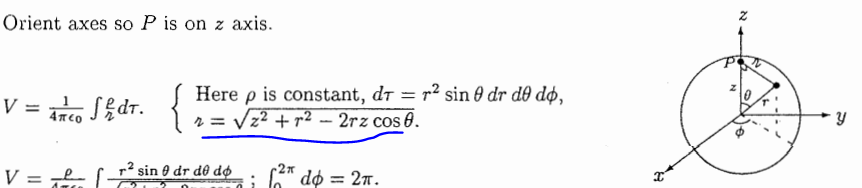0
$\begingroup$

This is a question of electrostatics from Griffiths.

Question: Calculate the potential inside a uniformly charged solid sphere of radius $R$ and total charge $q$

In the solution manual, the answer is given as this:

enter image description here

I want to know why don't we take the r (in the denominator) simply as the radius for a differential equation as the distance of any point in a sphere is equal to the radius of the circle having that point.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ It’s the distance from the infinitesimal element of charge $dq=\rho d\tau$ located at $(r,\theta,\phi)$ to the point where you are computing the potential it creates. All the charge of the ball is not at the origin; it is uniformly distributed throughout the ball. $\endgroup$
    – G. Smith
    Commented Jun 1, 2021 at 6:17
  • $\begingroup$ the radius for a differential equation What does a differential equation have to do with this integral? $\endgroup$
    – G. Smith
    Commented Jun 1, 2021 at 6:33

2 Answers 2

Reset to default
0
$\begingroup$

It's not a right-angle triangle as $(r,\theta)$ is changing. For example, see the triangle when $\theta=\pi/2.$

They draw the right angle by mistake, I think.

Improve this answer
$\endgroup$
0
$\begingroup$

The problem has cylindrical symmetry - the potential at $P$ will depend on the distance $z$ between $P$ and the centre of the sphere, but it will not depend on the angle by which the sphere is rotated about the axis through $P$. So cylindrical coordinates are a natural choice for framing the first integral for $V$. Griffiths then converts from cylindrical coordinates to spherical coordinates so that he can use the fact that $\rho$ takes a constant value in the region $0 \le r \le R$ i.e. within the sphere. The diagram with the right angled triangle is simply illustrating the conversion from cylindrical coordinates to spherical coordinates.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.