The nice Veritasium video about a wind-powered vehicle that can go downwind faster than the wind itself

Question

Today Derek Muller posted a video about a wind-powered vehicle that can go downwind faster than that downwind itself.

The vehicle is custom made for that case only: the case of going straight downwind.

The vehicle has three wheels, I estimate about 5 meters from front wheel to back wheels, and and a two-bladed propellor is mounted about 5 meters up.

The mechanical connection between the propellor and the wheels is such that when the vehicle is rolling forward the propellor is moving air from the front of the vehicle to the rear of the vehicle.

The vehicle is as lightweight as possible for its size, so it's quite flimsy. This is definitely not a practical device, it is a proof-of-concept device.

In the video Derek himself indicated that he was not quite confident that he understood the physics of it. I anticipate that questions will start coming in on physics SE, so I present this case as a self-answer.

How can this vehicle, when going straight downwind, go faster than the wind itself?

Previous question about that vehicle:
Details about mechanics of directly-downwind-faster-than-wind vehicle

Answer 1

Here is another way which may help you understand the physics here.

It is well-known that a land yacht (essentially a sail-powered go kart) can make its way over the ground at a speed which exceeds the speed of the wind, if the wind is blowing sideways across the path of the yacht.

This is also true of sailboats, and the key thing here is that the wind passing over the sail shapes it into a wing whose angle of attack can be trimmed in such a manner that the lift it generates furnishes the thrust needed to propel the vehicle.

By continuously trimming the AoA as the vehicle's speed builds, the relative wind is accomodated in such a way that the vehicle speed can and will exceed the velocity of the wind in a nonmoving frame.

Now, in the case of a windmill, the same ideas apply- only in this case, the wind does not have to be blowing sideways to the course of the vehicle, and if traveling straight into the wind, the vehicle's speed can exceed that of the wind in a nonmoving frame by the same factor as in the case of a sailboat or land yacht moving sideways relative to the wind.

Answer 2

The way this vehicle can go downwind faster than the wind itself is really neat.

If that vehicle would only harvest wind energy then it cannot go downwind faster than the wind. Instead of harvesting just wind energy the vehicle harvests from the difference in velocity between the air mass and the earth mass.

As the vehicle starts moving the wheels are driving the fan, and the fan rotates such that it builds an air cushion behind the vehicle. The wind from behind is pushing against that air cushion.

The fact that the wheels have traction is essential.

If you release a kite to fly free then the maximum velocity of the kite is the wind velocity itself. But the wheels have traction, and that gives the vehicle the leverage to harvest energy from the difference in velocity between the air mass of the wind and the earth mass.

In order to move forward into a headwind the wind must turn the blades and the blades must turn the wheels.

In the video the power transfer is from the wheels to the blades. The wheels have traction, the blades turn against the rear wind.

I expect that if that vehicle is at standstill and then a rear wind comes up, and if that rear wind is turning the blades, then the vehicle will start moving backward. It could be that the vehicle has some mechanism in place to prevent it from moving backward. Or maybe when a rear wind comes up it's enough to hand push it into a slow forward velocity.

Answer 3

I saw the same video and started thinking about it myself, because it felt so unintuitive that such a thing is even possible. However I am not a physicist so the exact workings of Blackbird are a bit hard for me to conceptualize, especially the turbine part of it. That is why I came up with the design for Whitemole.

Whitemole is a vehicle without an engine and with wheels on the top and bottom. This vehicle is not wind powered, but "surface powered". What I mean by that is that it moves between two flat surfaces that move relative to another, similar to the wind and the ground for Blackbird. To make things less abstract we can imagine for Whitemole a static ceiling (the ground) and a moving conveyor belt (the wind). The goal of Whitemole is to move faster than the conveyor belt it is standing.

Let $v$ be the velocity of the conveyor belt, then we want Whitemole with a factor $s$ that speed. To figure out how to do this it is best to start from the back by assuming that it achieves its goal of moving at $s\cdot v$. If it does then that means that the top wheels must have a rotational speed of $s \cdot v$. The bottom wheels are on the conveyor belt and will move at $v$ even if they are not rotating, that means that they should have a rotational speed of $(s-1)\cdot v$.

Now the trick is (like with Blackbird) to connect a wheel at the top with one at the bottom in such a way that if one rotates ate the speed you want, that the other will too. So the top wheel rotates at $s\cdot v$ if and only if the bottom wheel rotates at $(s-1)\cdot v$. Clearly the wheel on top rotates faster than the one at the bottom, so we cannot simply make the wheels touch another, however they do rotate in opposite directions, which means that if the top wheel was slower then it could touch the bottom wheel. We cannot an do not want to slow down the top wheel, but we can attach a smaller wheel to it with the same center of rotation and will get the same angular velocity ($\neq$ rotational speed). The smaller wheel will rotate at a speed that depends on its circumference relative to the bigger wheel. Let $C_{i}$ be the circumference of the smaller inner wheel and $C_{o}$ of the bigger outer wheel. If we want Whitemole to move at $s\cdot v$ speed, then $C_i / C_o = 1-\frac{1}{s}$ or $C_o / C_i = 1+\frac{1}{s-1}$ must be true.

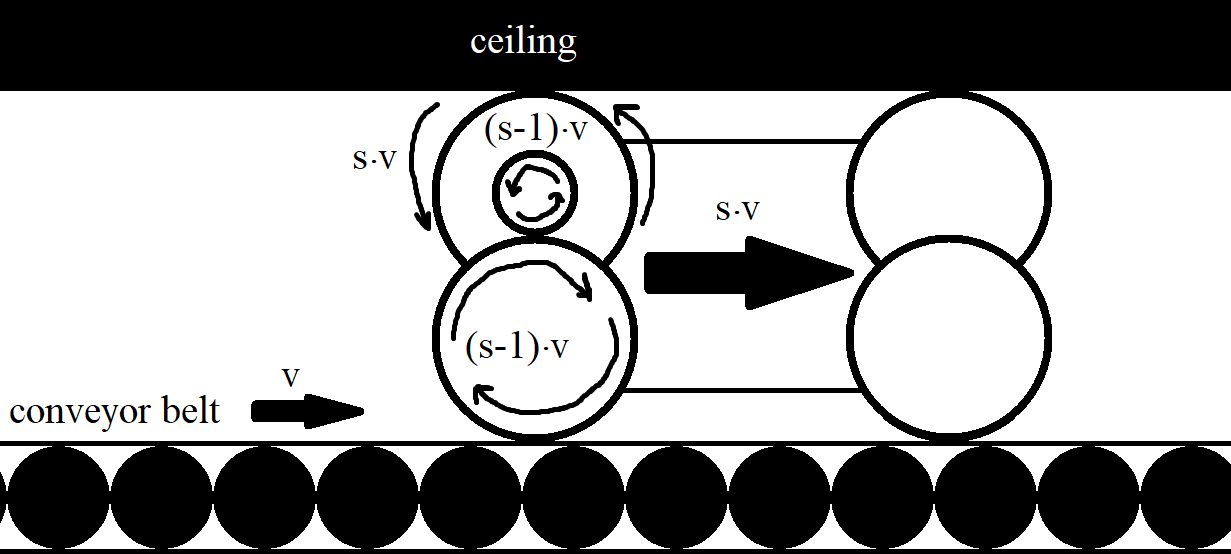
In other words if you choose the smaller inner wheel to have half the circumference of the outer bigger wheel, then Whitemole will travel double the speed of the conveyor belt. If you want ten times the conveyor belt speed, then the smaller circumference must be $\frac{9}{10}$ the size of the larger circumference. In fact there seems to be no upper bound to the factor $s$, because it approaches infinity as the smaller wheel approaches the exact size of the bigger wheel. Also in general as long as the smaller inner wheel has a circumference that is larger than 0 and is smaller than the bigger outer wheel, then Whitemole will be faster than the conveyor belt.

The setup of Whitemole might be different from Blackbird, but in essence they operate on the same principle. The frame of reference of Whitemole is the ceiling and for Blackbird the ground. Whitemole uses the conveyor belt to move faster than the conveyor belt and Blackbird uses the wind to move faster than the wind.

After thinking like this I came to the conclusion that the ceiling/floor is vital to get those higher speeds. In more general terms, if you want a vehicle to be faster than thing A using A's speed to propel it, then A must move relative to a thing B. Both thing A and B are needed for the vehicle to be faster than thing A. In other words an airship might not be able to move faster than the wind, unless maybe if it could move in between two opposite airstreams.

Answer 4

One should note the difference between reaching faster-than-the-wind travel and being able to maintain faster-than-the-wind travel (i.e. is the vehicle in steady-state when going faster than the wind).

The difference is in whether energy can be continuously harvested from the air (i.e. the wind) once the vehicle has reached the downwind speed, and passed it, or whether the vehicle depends at the point on energy that it had accumulated when it was traveling slower.

IMHO the energy at that point is no longer coming from the air stream, it is coming from the kinetic energy stored in the propeller. As long as it keeps rotating it is able to accelerate the vehicle, much like a propeller accelerates an aircraft. However, since this propeller is not powered, it is losing speed, due to mechanical friction and air resistance. Additionally, the propeller may transfer energy directly to the wheels through a proper gear ratio to allow the wheels to "extract" the kinetic energy of the rotating propeller and accelerate the vehicle through traction with the ground.

TL;DR: It probably goes faster than the wind due to the propeller acting as a flywheel once the vehicle reaches wind speed.