I'm modelling a football shot as a projectile - is the air resistance force proportional to the speed or speed squared?
$\begingroup$
$\endgroup$
3
-
$\begingroup$ Drag is proportional to squared of the speed. $\endgroup$– DKNguyenCommented May 26, 2021 at 22:53
-
$\begingroup$ Are you referring to the elongated shape of an American "football" or the spherical shape in other nationalities? (It doesn't really effect the answer I would write - only what data or figures I would use). $\endgroup$– D. HalseyCommented Jun 22, 2021 at 22:07
-
$\begingroup$ It depends on the speed, For lower speed, the linear term will be enough. For greater speed, you have to include the quadratic term. Under case of high-speed, both linear term and guadratic term are needed. Either cases, they are approximations. $\endgroup$– ytluCommented Jun 23, 2021 at 4:38
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
Neither.
The air resistance (or drag) of a football would be proportional to the speed squared only if the drag coefficient were constant. It would be linear only if the coefficient were proportional to the inverse of the speed.
Neither of these trends is actually the case. The viscous characteristics of the flow depend strongly on the Reynolds number (which is a function of speed), and cannot be characterized by such simple functions.
If you are referring to an American football, then the variation of the coefficient would be similar to the top curve in the following figure from Hoerner's Fluid Dynamic Drag
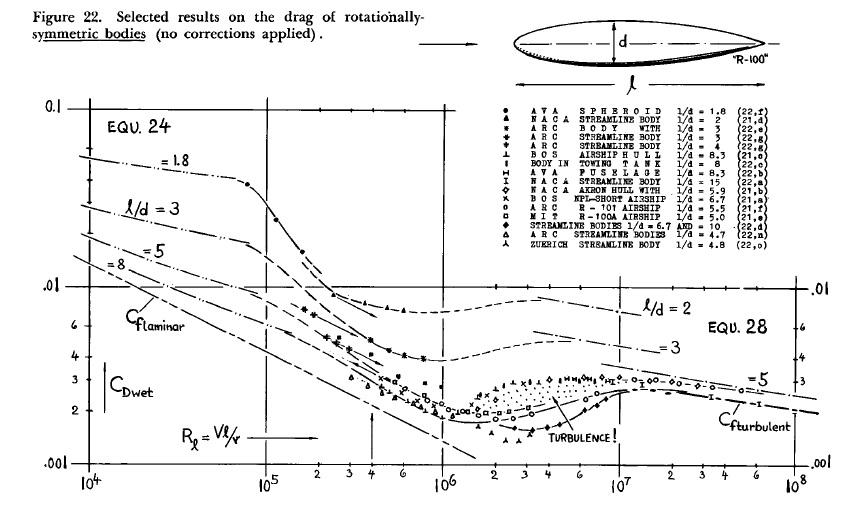
If you are referring to what the Americans call a "soccer ball," the coefficient would vary as shown in the figure from NASA (https://www.grc.nasa.gov/WWW/K-12/airplane/socdrag.html)
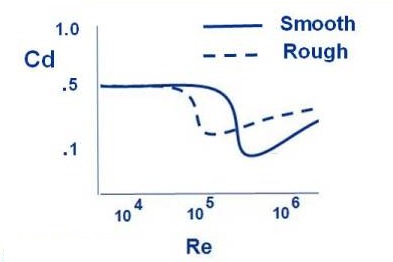
Note that I'm assuming the football is going straight through the air. If it is inclined and creating a lift force, the drag coefficient would be varying in even more complicated ways.
answered Jun 22, 2021 at 22:54
$\begingroup$
$\endgroup$
I think you should do quadratic air resistance because typical football velocities involve turbulent flow.
$\begingroup$
$\endgroup$
Abd al rahman shebani.
The FORCE of air resistance acting on a projectile is given by the formula: $F_D = (1/2)(C_D)(A)(\rho)(v^2)$
If we only consider the variables $F_D$ and $v$ we can arrive at:
$F_D$ is proportional to $v^2$
Hope this helps. Message me if you have any queries.
