About two photon interference in nanophotonics

Question

Within the standard model, photons are point particles, i.e., with no spatial distribution.

On the other hand, classical electromagnetic modes have field distributions.

Suppose there are two different single mode nanophotonic waveguides. The two waveguides are gradually bent and become adjacent to each other to form a coupler.

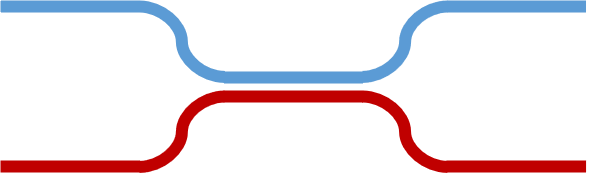
Two classical electromagnetic waves propagating along the two waveguides can couple to each other. For two photons, can they show the two photon interference effect in this structure?

If they can, how to calculate the probability that exactly one photon is detected at each exit, taking into consideration that the two waveguide eigenmodes have different field distributions?

I notice that, in the second quantization formalism, the creation operator is $$\hat{A}^{\dagger}(\alpha)=\int_{0}^{\infty} \mathrm{d} \omega \alpha(\omega) \hat{a}^{\dagger}(\omega),$$ where $\alpha(\omega)$ is the frequency distribution function of the excitation. Does this mean that the classical electromagnetic field distribution doesn't matter for quantum optics and the only important property is the frequency?

Update: In this paper, the probability that exactly one photon is detected at each exit is calculated as $$P\left(1_{\mathrm{a}}, 1_{\mathrm{b}}\right)=|t|^{4}+|r|^{4}+\left[t^{2} r^{* 2}+r^{2} t^{* 2}\right] I,$$ where $t$ and $r$ are the complex transmission and reflection coefficients. These coefficients can be calculated using the coupled mode theory which certainly takes the (classical) electromagnetic field distribution into consideration. This looks like a feasible formalism.

However, this calculation should be equivalent to some second-quantization formalism that involves the spatial field distribution, because this calculates the two photon interference possibility which should be a purely quantum mechanical quantity.

I wonder if there has been any literature that thoroughly established something like "waveguide quantum optics" in the language of second quantization.

Answer 1

Firstly, the optical field can have a spatial distribution, even though we observe them as point particles called photons. The spatial distribution is the wave functions that gives us a probability amplitude to observe a photon at a certain location. For multi-photon state, the situation can become a bit more complicated when we start to make correlated observations, because of the possibility for entanglement. The wave function of the photon represents its spatiotemporal properties.

For the waveguide structure, one can assume that it behaves as a beamsplitter. If we can assume that the probability for a photon to jump to the other guide is equal to the probability to stay in the guide in which it arrived, then one should be able to observe Hong-Ou-Mandel (HOM) interference, which is a form of two-photon interference. The effect of the HOM interference depends on the spatiotemporal properties of the wave functions. In a single-mode guide, the spatial properties are fixed and only the temporal properties would play a role.

If the spatiotemporal properties in the two guides are exactly the same, one would find that both photons would always exist from the same guide. On the other hand, if they have different spatiotemporal properties, one can find that two photons can exit from the two different guides. There combined state would be an entangled state, the singlet Bell state. Due to the entanglement, this state is not a classical state and can therefore not be described by the classical optics.

Not sure I've answered all your questions. Please let me know if I need to add anything.