What is physically changing from velocity or acceleration to force and their vector components?

Question

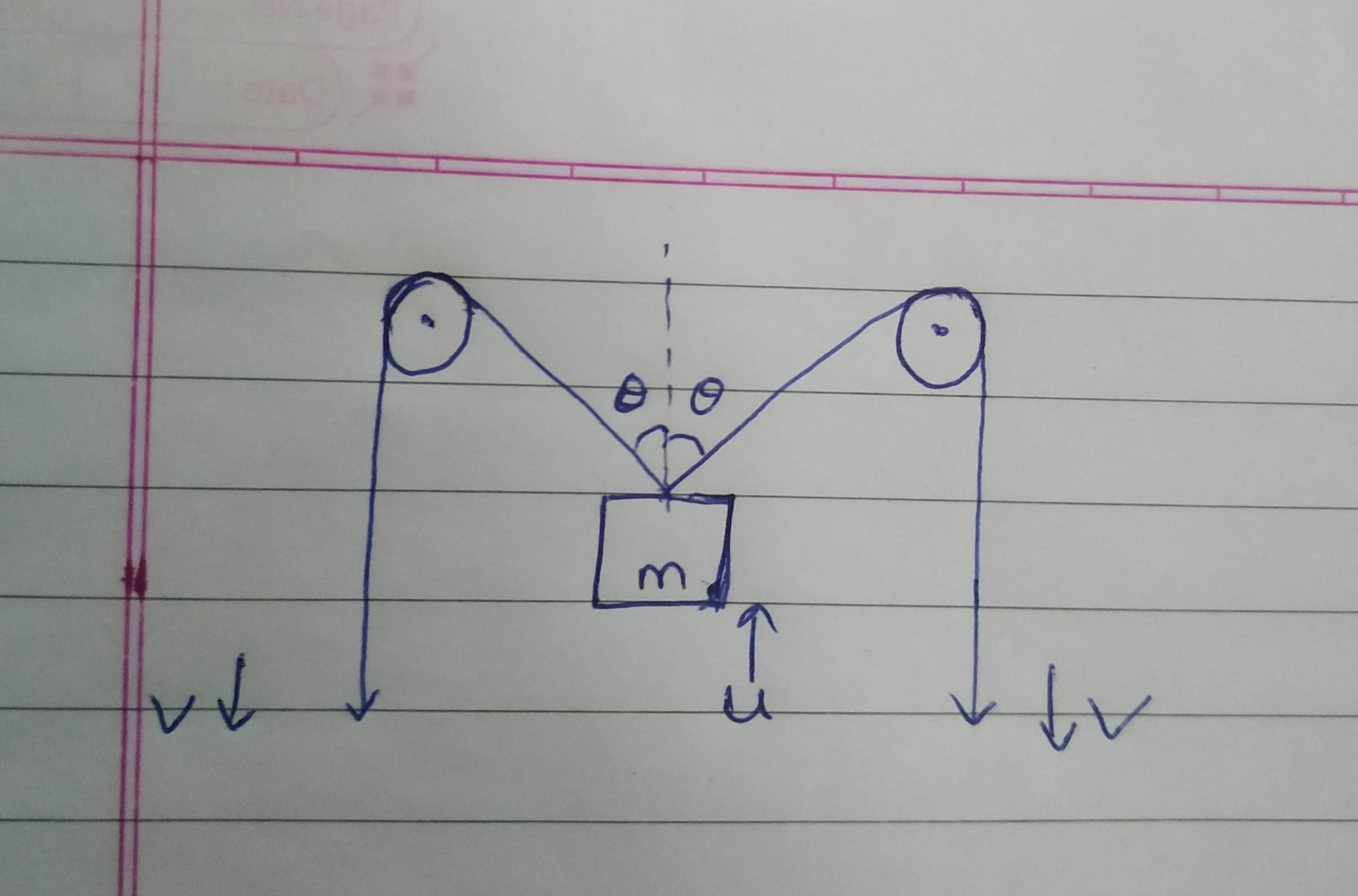
Here is question with two pulleys. https://physics.stackexchange.com/questions/342861/why-is-velocity-of-the-mass-v-cos-θ-why-not-2v-cos-θ

Its clear that $u =\frac{v}{\cos \theta} \tag{1}$

But now consider same arrangement with at both ends instead of $v$ are being pulled by force $F$ and the upward force acting on mass will be $F'$. Here

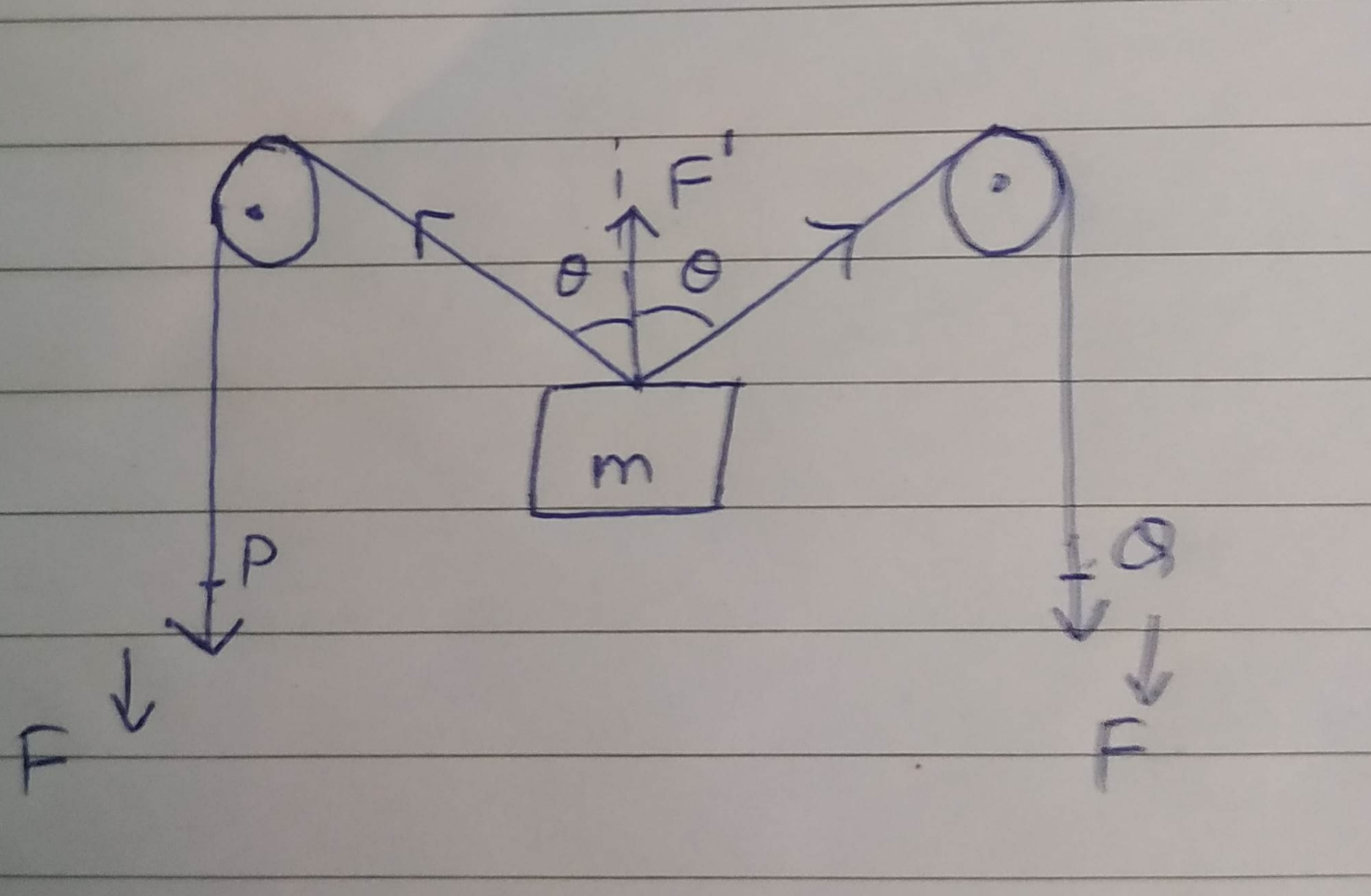
$F'=2F\cos\theta \tag{2}$

If we consider velocity or acceleration of point $P$ compared to that of mass, it shall be given by equation $(1)$.

So, my question is, what is changing in both scenarios that we give force on mass is component of force by rope but in both cases velocity of rope is component of velocity of mass? Because mass is a scalar quantity, so why is the nature of force different than that of acceleration (or velocity)?

Answer 1

Consider the energy or power balance of the phenomenon simplified by assuming uniform speeds $u$ and $v$ during the analyzed motion, neglecting power losses due to the friction and assuming that the mass of the rope and pulley system vanishes. In both cases we have from the geometry and thus kinematics that $u=\frac{v}{\cos\theta}$ and from Newton's second law of motion that $F'=2F\cos\theta$. Notice that in what follows, we do not explicitly applied Newton's second law of motion to the point mass but recover it from the law of conservation of mechanical energy (or the more dependable statement of the work-energy theorem). Applying the work-energy theorem we have $\int_0^t F' \cdot u(t) - mg \int_0^t \frac{d}{dt} u(t) \; dt = 2 \int_0^t F \cdot \frac{v(t)}{\cos \theta} - mg \int_0^t u(t) \; dt = \frac{1}{2} m (u(t)^2 - u(0)^2) = 0$, where the motion occurs over the time period $[0,t]$. This equation then recovers the consistent Newton's second law of motion $(F' - mg) \cdot u = 0$ or $F' = mg$ and $2F \cdot v - mg \cdot \frac{v}{\cos \theta} = 0$ or $F \cos \theta = \frac{mg}{2}$, as applied to the point mass. An interpretation which expresses this consistency of the mathematical description of the phenomenon is that the factor $\cos \theta$ shifts from the force to the speeds to preserve the consistency.