The position is given by $x=A\cos \omega t$ and $y=B\cos 2\omega t $. Show that it describes an arc of a parabola [closed]

Question

A particle moves in the $xy$ plane and its position is given by $x=A\cos \omega t $ and $y=B\cos 2\omega t$.

What do you mean by showing that it describes a parabolic arc?

I am working on harmonic oscillators.

Can you give me a suggestion? Got me a little confused.

Answer 1

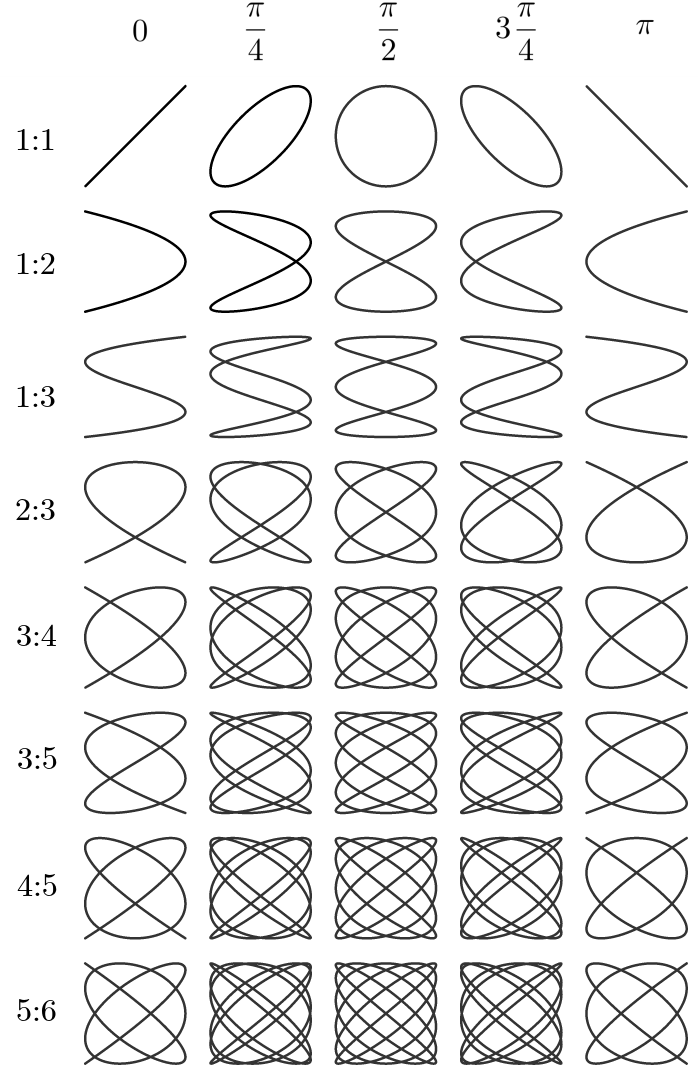
The parameter equation \begin{align} x & = A \cos(\omega t)\\ y & = B \cos(2 \omega t). \end{align}

You may draw this curve in an $xy$ plane for each pair $(x(t), y(t))$, the trajectory will resemble a parabolic curve. Or, you can eliminate the parameter $t$ to get the implicit trajectory:

\begin{align} y &= B \cos(2 \omega t) = B \left(2 \cos^2(\omega t) - 1 \right)\\ &= B \left( 2\left(\frac{x}{A}\right)^2 - 1 \right) \\[6pt] \Rightarrow\frac{y}{B} &= 2 \left(\frac{x}{A}\right)^2 - 1 \end{align}

This is why the trajectory $y(x)$ is a parabolic.

Answer 2

This is a well known Lissajous figure, with a frequency ratio of 1:2 and phase of 0: