Most computer monitors aren't capable of displaying any spectral color. Some of the RGB monitors could display at most three of them: some red wavelength, some green and some blue. This is because the gamut of the human vision is not triangular, instead it's curved and resembles a horseshoe:

In the image above, the black curve represents the spectral colors, with the wavelengths in nm denoted by green numbers. The colored triangle is the sRGB gamut, the standard gamut that most "usual" computer monitors are supposed to have.
As you can see, the black curve doesn't even touch the triangle, which means that sRGB monitors can't display any of the corresponding colors.
This doesn't mean that you can't see any good representation of the visible spectrum. You can e.g. display what the spectrum would look like if you took a gray card and projected the spectrum onto it, thus getting a desaturated version. CIE 1931 color space, via its color matching functions, lets one find, for each spectral color, corresponding color coordinates $XYZ$, which then can be converted to the coordinates in other color spaces like the above mentioned sRGB.
The inability of the sRGB monitors to display spectral colors manifests in the fact that, after you convert $XYZ$ coordinates to sRGB's $RGB$ ones, you'll get some negative components. Of course, negative amount of light is not something a display device can emit, so it needs some workaround to display these colors (or something close to them). Displaying the spectrum as projected on a gray card is one of these workarounds.
Here's how such a desaturated spectrum (with a scale) would look:
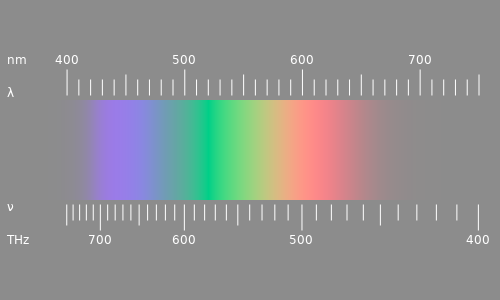
To get this (or any other, actually) image to display "correctly", ideally you need to calibrate your monitor. Some of the consumer devices have better color rendering out of the box, others have quite poor color rendering and show visibly wrong colors. If you don't calibrate, then just be aware of this nuance.
Also, if you happen to be a tetrachromat (virtually never happens in males, rare
in females), then the above image will look incorrect to you in any case.
How to see an actual spectrum, without the workarounds discussed above? For this you should use not a computer monitor. Instead you need a spectroscope. These can be found in online stores like AliExpress quite cheap, some using a diffraction grating, others a prism. The ones with grating will give you almost linear expansion in wavelengths, while the ones with a prism will have wider blue-violet part and thinner orange-red part of the spectrum.


