Time spent by a comet inside the Earth's orbital (Kepler problem re-visit)

Question

I come across this interesting problem comet-x-earth. It was an exam problem asking the time that a comet will be spent inside the Earth's orbital. I make an illustration for the problem:

The comet is in a parabolic orbit (i.e. its total energy = 0). It comes inside the Earth's orbit. The nearest position of the comet's orbit is $\beta R$, where $\beta <1$ and $R$ the radius of earth orbit (assume circular). The problem asked for the time that the comet travels from point $A$ to point $B$.

The link had a nice answer starting with the polar equation of a parabolic, determined the parameter of equation at the nearest point, found out the intersection position $A$ and $B$; then wrote down the integral.

Out of curiosity, I like to ask the question: is there a way of solving this problem without referring to the equation of the comet's orbit?

Answer 1

Is there a way of solving this problem without referring to the equation of the comets?

Yes. You do not need to use either the polar form or the Cartesian form of the comet's parabolic trajectory. You can work with the differential equations satisfied by the trajectory, rather than with their solution.

Write the differential equations for the conservation of energy and the conservation of angular momentum, in polar coordinates. They will involve $\dot r$, $\dot\theta$, and $r$. Eliminate $\dot\theta$ to get a relationship between $\dot r$ and $r$.

There will be an unknown constant, the specific angular momentum of the comet. (This is its angular momentum divided by its mass.) Evaluate it by imposing the conditions that at closest approach, $r=\beta R$ and $\dot r=0$. (Do this in both of the original differential equations.)

Separate the variables in the equation relating $\dot r$ and $r$ to get a differential relationship of the form $dt=f(r)dr$. Integrate the right side from $r=\beta $R to $r=R$. (This integral has a different form from the integral hint in the original question.) Double this time to get the time from A to B.

At the end, you will need to recognize a particular combination of $G$, $M$ (the mass of the Sun), and $R$ as the period of the Earth's orbit, namely one year.

Answer 2

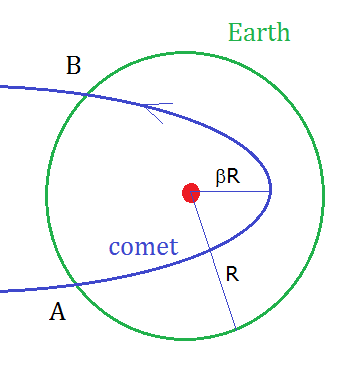
I try to work it out following the clues from G.Smith for a complete record.

in 2-d polar coordinate, the velocity $\vec{v}$

$$ \vec{v} = \dot{r} \hat{r} + r \dot{\theta} \hat{\theta} = v_r \hat{r} + v_{\theta} \hat{\theta} $$

The radial component $ v_r = \dot{r} = \frac{dr}{dt}$. The time $T$ from point $A$ to point $B$ can be write as the integral:

$$ T = 2 \int_{\beta R}^R \frac{dr}{v_r}. \tag{1} $$ We then will try to relate $v_r$ as function of $r$ in order to do this integral.

Conservation Laws

Conservation of total energy $$ E(t) = -\frac{G M m}{r(t)} + \frac{1}{2} m ( v_r^2 + v_{\theta}^2 ) = 0 \tag{2} $$

At the nearest point, $\vec{r} = v_{\theta 0} \hat{\theta}$, $v_{r0} = 0$ at $r_o = \beta R$. We find the speed at the nearest point.

$$ v_{\theta 0} = \sqrt{ \frac{2GM}{\beta R} } \tag{3} $$

Conservation of total angular momentum

$$ L_z = m r v_\theta = m r_o v_{\theta 0} = m \sqrt{2 G M\beta R } \tag{4} $$ We obtain a $v_\theta$ as a function of $r$ from Eq.(4) $$ v_\theta (r) = \sqrt{2 G M\beta R } \frac{1}{r} \tag{5} $$

Function $v_r(r)$

Substitute Eq.(5) into Eq.(2) to find the $v_r$ as function of $r$:

$$ v_r = \sqrt{ \frac{2GM}{R} } \sqrt{ \frac{R}{r} - \beta \frac{R^2}{r^2} }. $$

Place this relation into the Eq.(1) to complete the integral form:

$$ T = 2 \sqrt{\frac{\beta R}{2 G M}} \int_{\beta R}^{R} \frac{dr}{\sqrt{ \frac{\beta R}{r} - \beta^2 \frac{R^2}{r^2} } } $$

Integral

Let $\xi = \frac{r}{\beta R}$, the integral becomes

$$ T =2\beta R \sqrt{\frac{\beta R}{2 G M}} \int_1^{1/\beta} \frac{\xi d\xi}{\sqrt{\xi - 1} } = \sqrt{\frac{2 \beta^3 R^3}{ G M}} \int_0^{1/\beta - 1} (\sqrt{\eta} + \frac{1}{\sqrt{\eta}} ) d\eta. $$ In the fianl expression, we substitue $\eta = \xi -1$.

$$ T = \sqrt{\frac{2 \beta^3 R^3}{ G M}} \{ \frac{2}{3} (\frac{1}{\beta} - 1)^{3/2} + 2 (\frac{1}{\beta}-1)^{1/2} \} $$ Replace $R$ by the period of Earth $T_0 = 1 year$ using relation $T_0^2 = \frac{4\pi^2}{GM} R^3$, we finally have: $$ T = \frac{T_0}{ 3 \pi } (1 + 2 \beta) \sqrt{2(1-\beta)}. $$