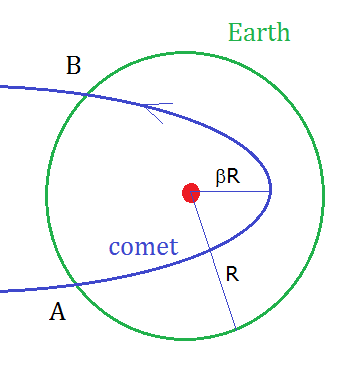
I come across this interesting problem comet-x-earth. It was an exam problem asking the time that a comet will be spent inside the Earth's orbital. I make an illustration for the problem:
The comet is in a parabolic orbit (i.e. its total energy = 0). It comes inside the Earth's orbit. The nearest position of the comet's orbit is $\beta R$, where $\beta <1$ and $R$ the radius of earth orbit (assume circular). The problem asked for the time that the comet travels from point $A$ to point $B$.
The link had a nice answer starting with the polar equation of a parabolic, determined the parameter of equation at the nearest point, found out the intersection position $A$ and $B$; then wrote down the integral.
Out of curiosity, I like to ask the question: is there a way of solving this problem without referring to the equation of the cometscomet's orbit?