0
$\begingroup$

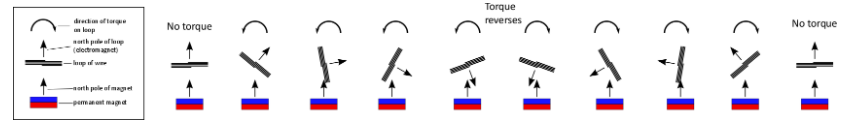
I understand how the torque depends on the number of turns as $$\tau = NiAB \sin{\theta}$$ where $\tau$ is the torque, $N$ the number of loops, $i$ the DC intensity, $B$ the magnetic field strength and $\theta$ the angle between the coil and the magnetic field.

Therefore, I understand how the torque will increase if we increase the number of loops and keep the rest constant. However, the angular speed $\omega$ will also depend on the mass of the coil though the moment of inertia $I$ because $$\tau = NI\alpha = NI \frac{\mathrm{d}\omega}{\mathrm{d}t}$$ where $I$ is the moment of inertia of a single coil. Therefore, $$ \frac{\mathrm{d}\omega}{\mathrm{d}t} = \frac{i A B}{I} \sin{\theta}.$$

So, regardless of the solution to this equation, it should not depend on the number of turns, but here and here it says it depends.

What did I do wrong?

Improve this question
$\endgroup$
4
  • $\begingroup$ Where did you get this from? $\tau = NI\alpha$ $\endgroup$
    – Daddy Kropotkin
    Commented Jan 8, 2021 at 12:46
  • $\begingroup$ From the equation of the relationship between angular acceleration and torque T=Ia. Using a total moment of inertia NI $\endgroup$
    – jrglez
    Commented Jan 8, 2021 at 13:07
  • $\begingroup$ Why is the "total inertia" simply additive? $\endgroup$
    – Daddy Kropotkin
    Commented Jan 8, 2021 at 13:09
  • $\begingroup$ I assume that the radius of the loops is much bigger than the thickness of the coils, which is the same approximation that you make when you calculate to total torque of several loops. Under that assumption, when you calculate $I = \int r^2 dm$, you can write $dm=\lambda dl $ ($\lambda$ is the linear density and $dl$ a small fragment of the coil). When you have $N $wires, the linear density is $N \lambda$, and $I_{total} = N I$ $\endgroup$
    – jrglez
    Commented Jan 8, 2021 at 17:35

1 Answer 1

Reset to default
0
$\begingroup$

Your mistake is in your conclusion that $$\tau = NI\alpha$$ where $I$ is the moment of inertia of a single loop. It's not that simple. The moment of inertia along any particular axis depends on the mass of the body and also on the distribution of that mass relative to that axis: $$I=\int r^2\mathrm{d}m$$ where $r$ is the distance of an elemental mass $\mathrm{d}m$ from the axis of rotation.

Now consider a current carrying loop in a DC motor. Here, the loop rotates around an axis that passes through its plane and is parallel to one of its sides as shown here.

1

See how the axis of rotation is perpendicular to the screen of the device you are reading this on. Now if we add more loops to this motor, then each loop wont contribute equally to the moment of inertia. The distance of every loop from the axis is not the same.

As $I=\int r^2\mathrm{d}m$ the contribution of each loop to the moment of inertia dies off as the square of the distance of that loop from the axis. Hence we cannot say that the moment of inertia of the whole body is $N$ times the moment of inertia of one loop.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Can you provide a reference for the source of your diagram? That would help interested people to learn more about basic properties of simple electric motors. $\endgroup$
    – Carl Witthoft
    Commented Jan 8, 2021 at 15:08
  • $\begingroup$ I assume that the radius of the loops is much bigger than the thickness of the coils, which is the same approximation that you make when you calculate to total torque of several loops. Under that assumption all the loops are at the same distance from the axis of rotation. When you calculate $I = \int r^2 dm$, you can write $dm=\lambda dl $ ($\lambda$ is the linear density and $dl$ a small fragment of the coil). When you have $N $wires, the linear density is $N \lambda$ and $dm=N\lambda dl$. Therefore $I_{total} = N I$ $\endgroup$
    – jrglez
    Commented Jan 8, 2021 at 17:41
  • $\begingroup$ @CarlWitthoft I took the diagram from a link mentioned in the question itself. Here is the link physics.stackexchange.com/questions/64892/… $\endgroup$
    – Ethan
    Commented Jan 8, 2021 at 18:17
  • $\begingroup$ @jrglez No all the loops aren't at the same distance from the axis of rotation. Think of the plane of the loop as the $xy$ plane. The axis of rotation will also lie in this plane. When we add loops we add it both in the $+z$ and $-z$ directions. Therefore each successive loop will be farther and farther away from the axis of rotation which is in the $xy$ plane. $\endgroup$
    – Ethan
    Commented Jan 8, 2021 at 18:20
  • $\begingroup$ @Aryamann thanks -- guess now I gotta ask that answerperson for the source. $\endgroup$
    – Carl Witthoft
    Commented Jan 8, 2021 at 18:44

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.